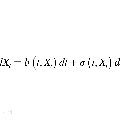随机辛算法和多辛算法

项目名称： 随机辛算法和多辛算法

项目编号： No.11471310

项目类型： 面上项目

立项/批准年度： 2015

项目学科： 数理科学和化学

项目作者： 王丽瑾

作者单位： 中国科学院大学

项目金额： 65万元

中文摘要： 本项目围绕两个课题进行研究。课题一：随机Hamilton常微分方程辛算法，研究基于分裂法，复合法，Pade逼近和随机积分插值的随机辛算法的构造、收敛阶分析和数值实验。这些方法属于基于方程本身的算法，是三大类构造辛算法的途径之一，项目旨在将确定性辛算法基于这一途径的方法推广到随机系统。此外，利用我们前期关于随机生成函数的研究结果对随机辛算法进行向后误差分析；课题二：随机多辛Hamilton偏微分方程的多辛算法，包括时间上采用分裂步、指数积分子等方法构造随机多辛格式，以及空间上采用有限元、拟谱、高维空间采用分裂方法等构造随机多辛格式，并将这些格式应用于一些物理中的方程，研究其长时行为和保结构特性。

中文关键词： 随机微分方程；辛几何算法；数值分析；数值方法；数值计算

英文摘要： The project is aimed at investigating two subjects. Subject 1 is about stochastic symplectic methods for stochastic Hamiltonian ODEs, including the construction of the stochastic symplectic methods based on splitting methods, composition methods, Pade approximation and stochastic interpolation, which belong to the class of approaches establishing symplectic methods for deterministic Hamiltonian systems based on the differential equations themselves. We hope to generalize these methods to the stochastic context. Convergence order will be analyzed and numerical tests will be performed. On the other hand, we apply our former results on stochastic generating functions to the backward error analysis of stochastic symplectic methods; Subject 2 is about stochastic multi-symplectic methods for stochastic Hamiltonian PDEs, including the construction of such methods using splitting methods, exponential integrators in the time direction, as well as using finite element methods, pseudo-spectral methods and splitting methods in the space direction. Further we apply these methods to some stochastic models in physics to test their long time behavior and structure-preserving properties.

英文关键词： stochastic differential equations;symplectic methods;numerical analysis;numerical methods;numerical computation