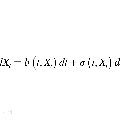保险中两类随机最优控制问题及策略过程概率分布研究

项目名称： 保险中两类随机最优控制问题及策略过程概率分布研究

项目编号： No.11471183

项目类型： 面上项目

立项/批准年度： 2015

项目学科： 数理科学和化学

项目作者： 梁宗霞

作者单位： 清华大学

项目金额： 58万元

中文摘要： 本项目基于保险业中经济问题,保险数学和随机分析的新问题及最新成果,开展保险中两类随机最优控制问题及策略过程概率分布研究： 第一,混合保费原理下有破产概率和VaR(在险值)约束(或无约束)的分红与融资最优控制问题; 第二,随机利率、随机通胀率和随机波动率环境中养老金的最优管理与控制问题. 对相关各类风险(过程)模型(1)求解上述最优控制问题的值函数和最优策略过程;(2)研究值函数的正则性,特别分数Soblev性质;(3)研究最优策略过程的概率分布极限性质和关于空间变量及轨道变量的Soblev正则性质;(4)研究相应的反射SDE和HJB方程系统的可解性问题;(5)数值分析. 这两类问题本质上是复杂脉冲和time-inconsistency随机最优控制,具有非线性,非正则性,非一致椭圆性,不完备性和时间不相容性等创新特色,是保险数学中热点和难点问题,也是有根的数学前沿问题.

中文关键词： 随机分析；随机微分方程；随机最优控制；最优策略；值函数

英文摘要： Based on real insurance market,the recent results and new problems in insurance mathematics and stochastic analysis,this project will study the following two stochastic optimal control problems: 1.Stochastic optimal control of dividend and (re)investment with(or without)ruin probability and VaR constraints in mixed premium principles; 2. Optimal management and controls of pension funds under interest rate and inflation as well as volatility risks. We focus on (1)closed-forms of value functions and optimal strategies;(2) Sobolev regularities of the value functions;(3)probability distributions of the optimal strategy processes and their Sobolev regularity with respect to (x,w);(4) SDE with reflections and HJB equations associated the two optimal controls problems 1-2;(5)sensitivity analysis. The two optimal controls problems are essentially complex impulse and time- inconsistency control problems.They are open and innovation in insurance mathematics and stochastic analysis due to the appearance of non-linearity,non-regularity, non-uniform ellipse,incompleteness and time-inconsistency. So they are also advance studies of mathematics that come from real insurance economic problems.

英文关键词： Stochastic Analysis;Stochastic differential equations;Stochastic Optimization and controls;Optimal strategy;The value function