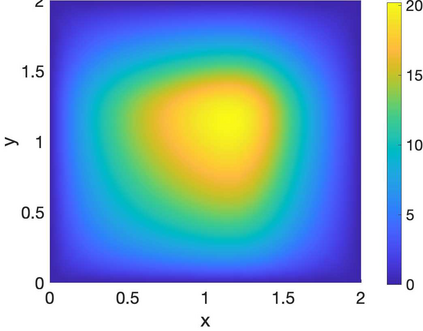
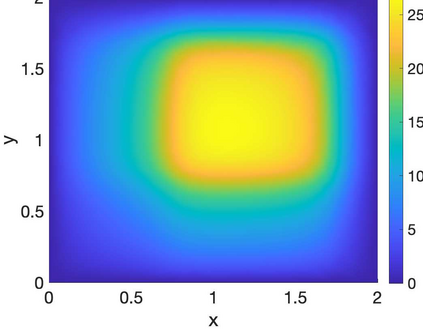
The main focus of this article is to provide a mathematical study of the algorithm proposed in \cite{boyaval2010variance} where the authors proposed a variance reduction technique for the computation of parameter-dependent expectations using a reduced basis paradigm. We study the effect of Monte-Carlo sampling on the theoretical properties of greedy algorithms. In particular, using concentration inequalities for the empirical measure in Wasserstein distance proved in \cite{fournier2015rate}, we provide sufficient conditions on the number of samples used for the computation of empirical variances at each iteration of the greedy procedure to guarantee that the resulting method algorithm is a weak greedy algorithm with high probability. These theoretical results are not fully practical and we therefore propose a heuristic procedure to choose the number of Monte-Carlo samples at each iteration, inspired from this theoretical study, which provides satisfactory results on several numerical test cases.
翻译:本文的主要重点是提供一份数学研究,研究在\cite{boyaval2010 varience}中提议的算法,作者在其中提议了一种减少差异的方法,用于利用一个减少的基础范式来计算依赖参数的预期值。我们研究了蒙特-卡洛抽样对贪婪算法的理论性质的影响。特别是,利用在\cite{fournier2015rate}中证明的瓦瑟斯坦距离的经验性测量中的集中不平等,我们为计算贪婪程序每次反复出现的实验差异时使用的样本数量提供了充分的条件,以保证由此产生的方法算法是一种弱的贪婪算法,其概率很高。这些理论结果并不完全实用,因此我们建议了一种超常程序,以选择每次反复出现的蒙特-卡洛样本数量,这从这一理论研究中得到启发,它为几个数字测试案例提供了令人满意的结果。