Efficient GPU implementation of a class of array permutations

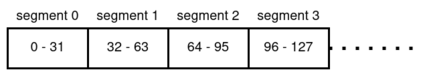
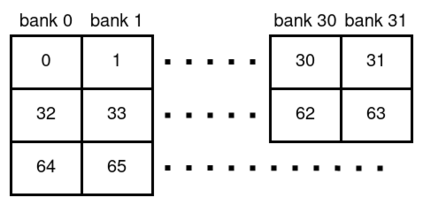
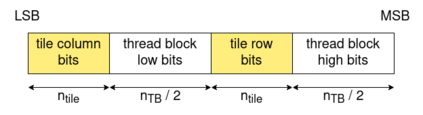
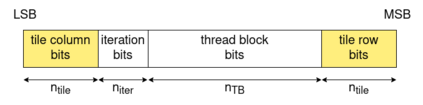
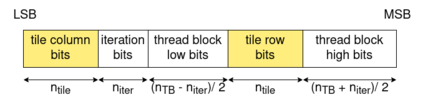
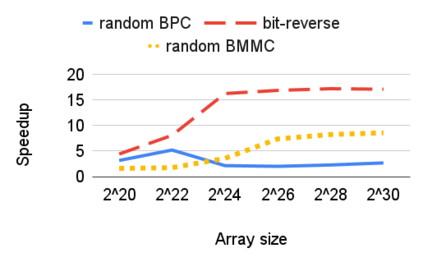
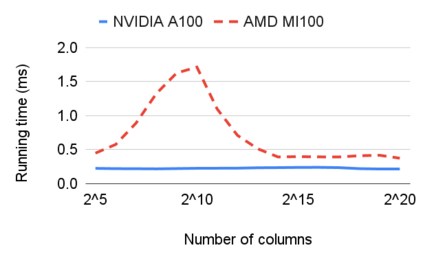
Optimal usage of the memory system is a key element of fast GPU algorithms. Unfortunately many common algorithms fail in this regard despite exhibiting great regularity in memory access patterns. In this paper we propose efficient kernels to permute the elements of an array, which can be used to improve the access patterns of many algorithms. We handle a class of permutations known as Bit Matrix Multiply Complement (BMMC) permutations, for which we design kernels of speed comparable to that of a simple array copy. This is a first step towards implementing a set of array combinators based on these permutations.