A weighted Hybridizable Discontinuous Galerkin method for drift-diffusion problems

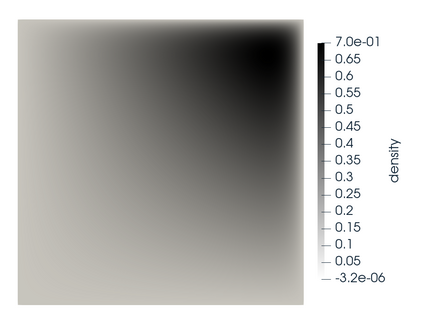
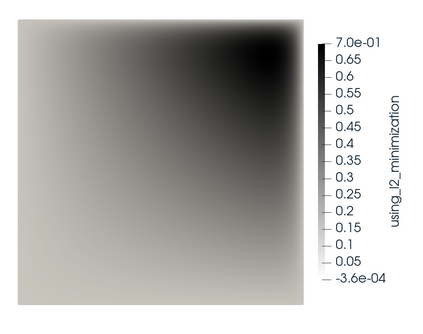
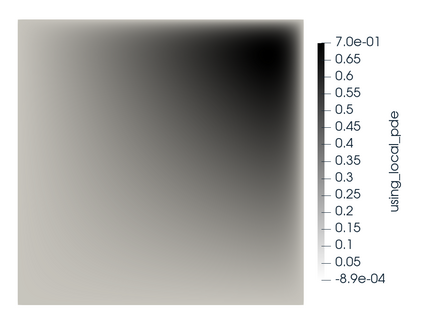
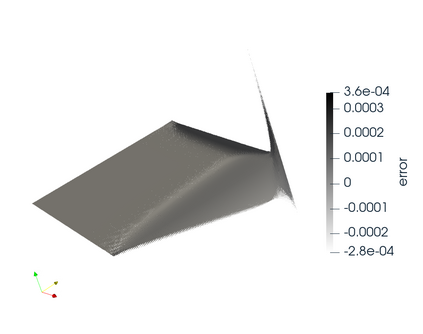
In this work we propose a weighted hybridizable discontinuous Galerkin method (W-HDG) for drift-diffusion problems. By using specific exponential weights when computing the $L^2$ product in each cell of the discretization, we are able to replicate the behavior of the Slotboom change of variables, and eliminate the drift term from the local matrix contributions. We show that the proposed numerical scheme is well-posed, and numerically validates that it has the same properties of classical HDG methods, including optimal convergence, and superconvergence of postprocessed solutions. For polynomial degree zero, dimension one, and vanishing HDG stabilization parameter, W-HDG coincides with the Scharfetter-Gummel stabilized finite volume scheme (i.e., it produces the same system matrix). The use of local exponential weights generalizes the Scharfetter-Gummel stabilization (the state-of-the-art for Finite Volume discretization of transport-dominated problems) to arbitrary high-order approximations.