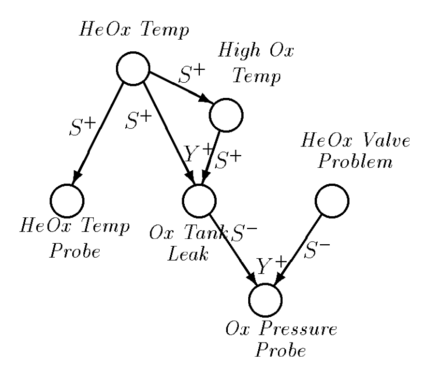
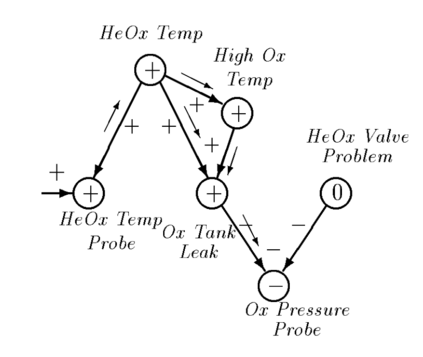
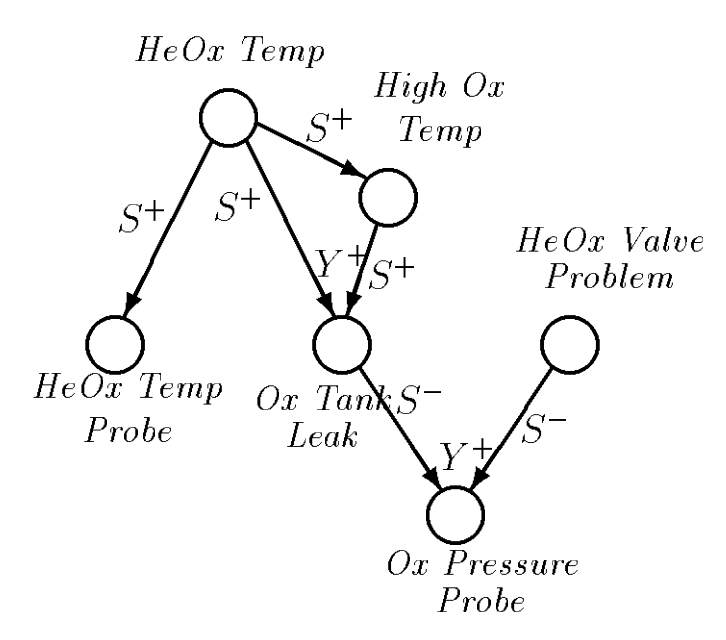
Qualitative probabilistic networks (QPNs) combine the conditional independence assumptions of Bayesian networks with the qualitative properties of positive and negative dependence. They formalise various intuitive properties of positive dependence to allow inferences over a large network of variables. However, we will demonstrate in this paper that, due to an incorrect symmetry property, many inferences obtained in non-binary QPNs are not mathematically true. We will provide examples of such incorrect inferences and briefly discuss possible resolutions.
翻译:定性概率网络(QPNs)将巴伊西亚网络的有条件独立假设与正依赖性和负依赖性的质量特性结合起来,它们将正依赖性的各种直觉特性正规化,以便能够对大型变量网络作出推论,然而,我们将在本文中表明,由于不正确的对称性属性,许多非二进制QPNs获得的推论在数学上是不真实的,我们将举例说明这种不正确的推论,并简要讨论可能的解决办法。
相关内容
Networking:IFIP International Conferences on Networking。
Explanation:国际网络会议。
Publisher:IFIP。
SIT: http://dblp.uni-trier.de/db/conf/networking/index.html
专知会员服务
78+阅读 · 2022年3月15日
专知会员服务
36+阅读 · 2019年10月17日
Arxiv
14+阅读 · 2019年9月17日