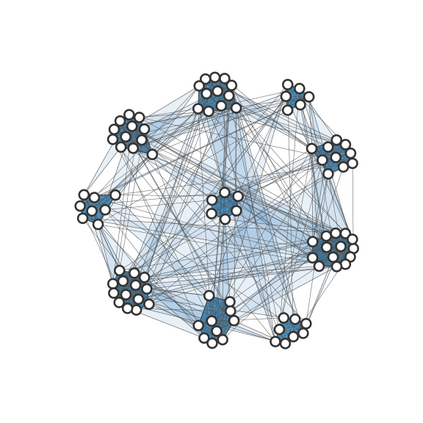
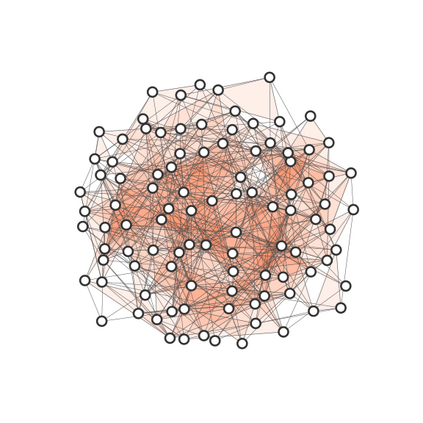
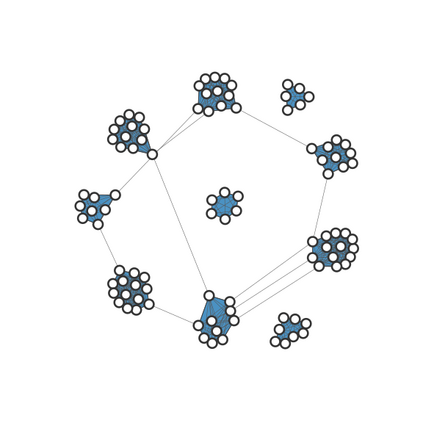
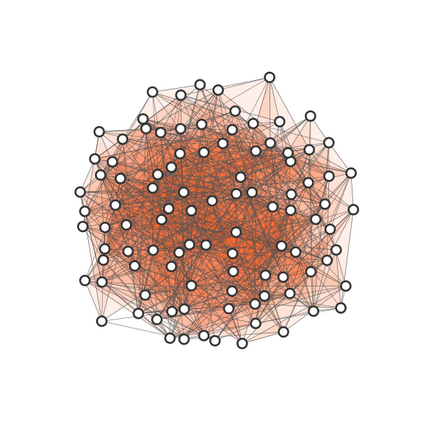
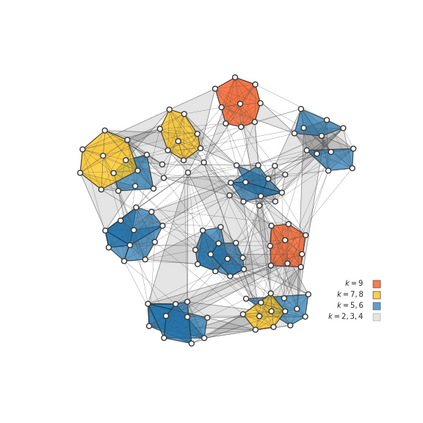
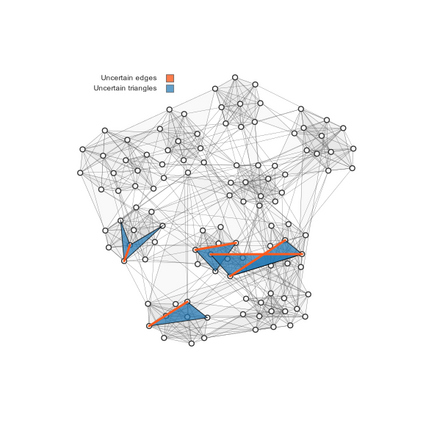
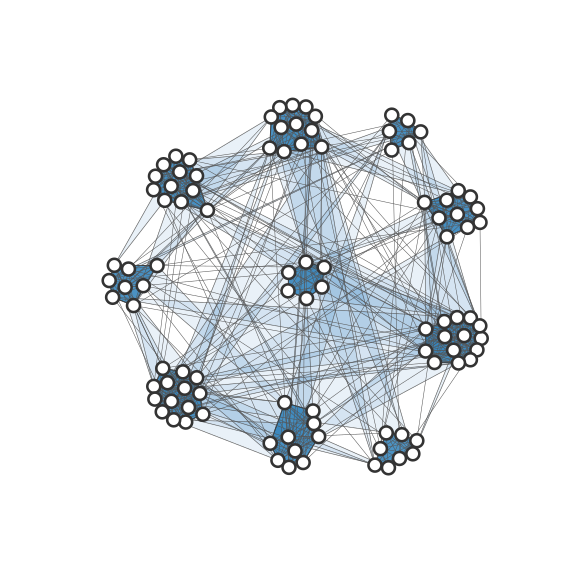
Networks can describe the structure of a wide variety of complex systems by specifying which pairs of entities in the system are connected. While such pairwise representations are flexible, they are not necessarily appropriate when the fundamental interactions involve more than two entities at the same time. Pairwise representations nonetheless remain ubiquitous, because higher-order interactions are often not recorded explicitly in network data. Here, we introduce a Bayesian approach to reconstruct latent higher-order interactions from ordinary pairwise network data. Our method is based on the principle of parsimony and only includes higher-order structures when there is sufficient statistical evidence for them. We demonstrate its applicability to a wide range of datasets, both synthetic and empirical.
翻译:网络可以描述各种复杂系统的结构,具体指明系统中哪些实体相互连接,这种对称式虽然具有灵活性,但当基本互动同时涉及两个以上实体时,它们不一定适当,但对称式仍然无处不在,因为网络数据中往往没有明确记录较高层次的相互作用。在这里,我们采用巴伊斯式方法,从普通对称网络数据中重建潜在的较高层次的相互作用。我们的方法基于面孔原则,只有在有足够的统计证据时,才包括较高层次的结构。我们证明它适用于广泛的数据集,包括合成数据集和经验数据集。