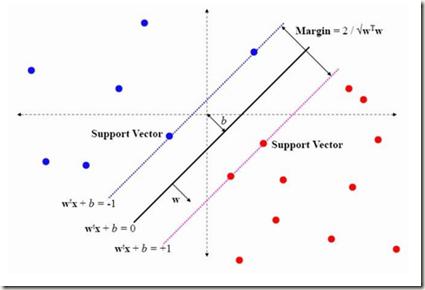
This paper presents approaches to compute sparse solutions of Generalized Singular Value Problem (GSVP). The GSVP is regularized by $\ell_1$-norm and $\ell_q$-penalty for $0<q<1$, resulting in the $\ell_1$-GSVP and $\ell_q$-GSVP formulations. The solutions of these problems are determined by applying the proximal gradient descent algorithm with a fixed step size. The inherent sparsity levels within the computed solutions are exploited for feature selection, and subsequently, binary classification with non-parallel Support Vector Machines (SVM). For our feature selection task, SVM is integrated into the $\ell_1$-GSVP and $\ell_q$-GSVP frameworks to derive the $\ell_1$-GSVPSVM and $\ell_q$-GSVPSVM variants. Machine learning applications to cancer detection are considered. We remarkably report near-to-perfect balanced accuracy across breast and ovarian cancer datasets using a few selected features.
翻译:暂无翻译