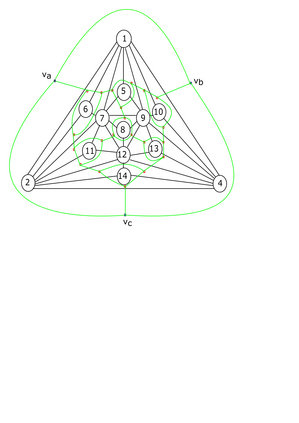
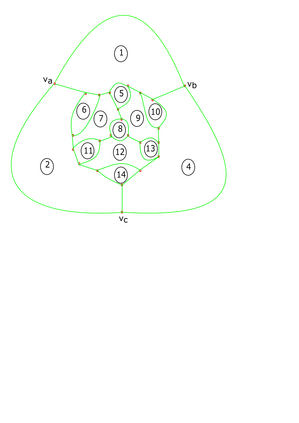
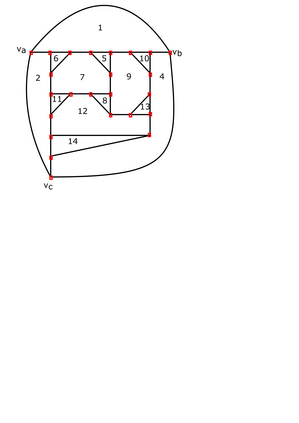
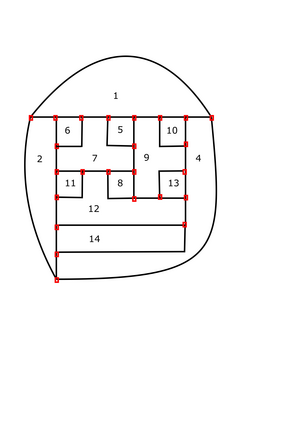
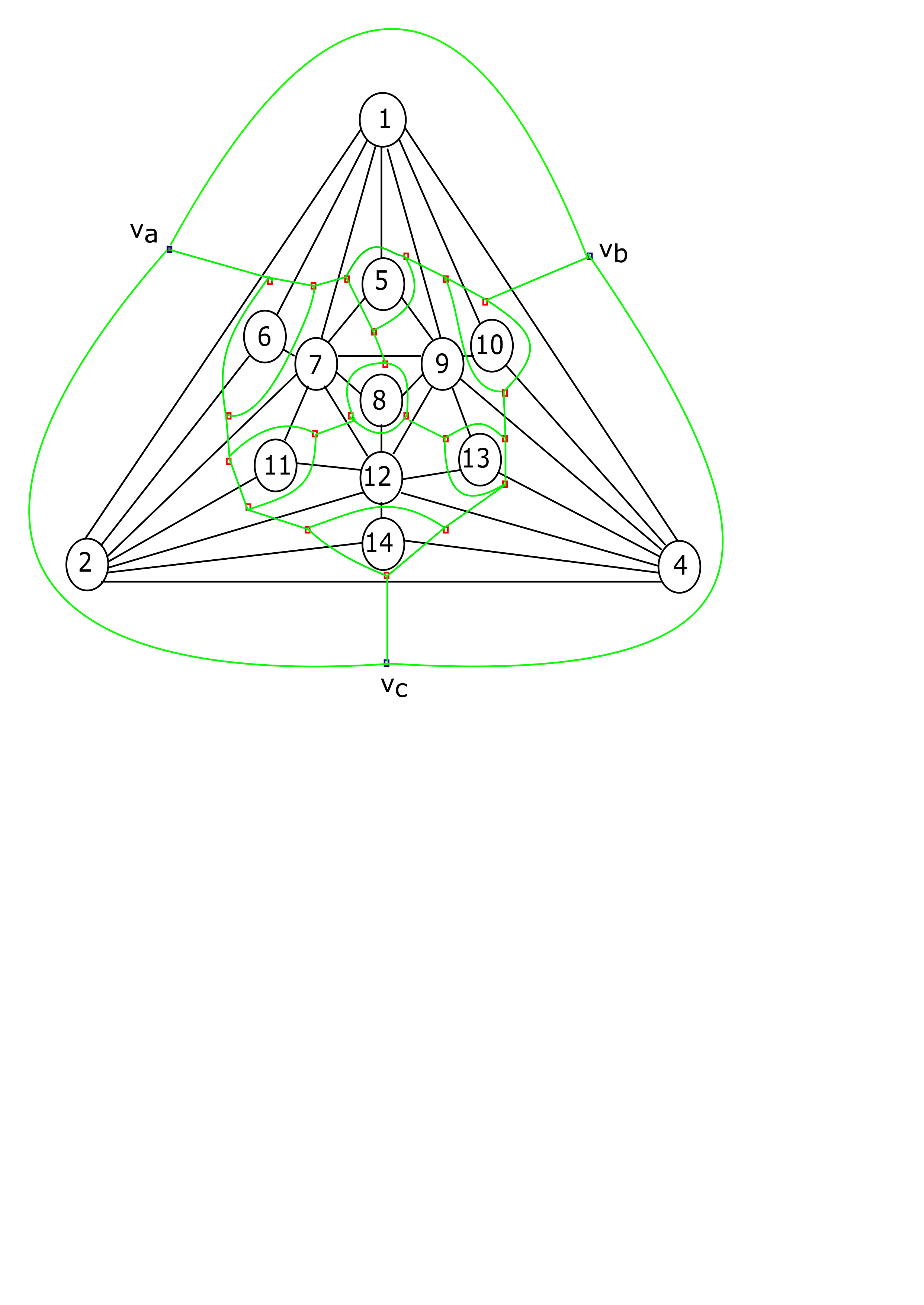
Let G = (V, E) be a planar triangulated graph (PTG) having every face triangular. A rectilinear dual or an orthogonal floor plan (OFP) of G is obtained by partitioning a rectangle into \mid V \mid rectilinear regions (modules) where two modules are adjacent if and only if there is an edge between the corresponding vertices in G. In this paper, a linear-time algorithm is presented for constructing an OFP for a given G such that the obtained OFP has B_{min} bends, where a bend in a concave corner in an OFP. Further, it has been proved that at least B_{min} bends are required to construct an OFP for G, where \rho - 2 \leq B_{min} \leq \rho + 1 and \rho is the sum of the number of leaves of the containment tree of G and the number of K_4 (4-vertex complete graph) in G.
翻译:Let G = (V, E) 是一个带有每个面形三角形的平面三角图( PTG ) 。 通过将一个矩形分割到两个模块在 G 中对应的顶端之间有边缘的等离心矩形区域( modules), 可以获得一个G 的直线双向或正方平面平面平面平面平面图( OFP ) 。 此外, 已经证明至少需要 B ⁇ min} 弯曲才能为 G 构造一个矩形平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面图。 在本文中, 直面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面图。 此外平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面图( OP 。 此外,至少平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平