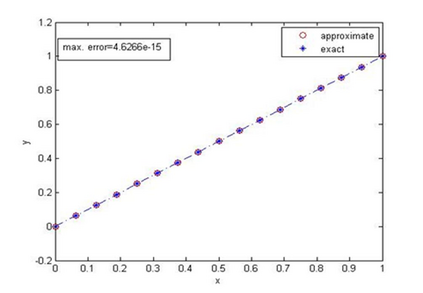
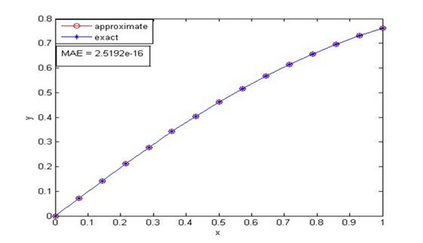
The purpose of this paper is to show and explain a new formula that indicates with finality the derivatives of Shifted Monic Ultraspherical polynomials (SMUPs) of any degree and for any fractional-order using the shifted Monic Ultraspherical polynomials themselves. We also create a direct method solution for the linear or nonlinear multi-order fractional differential equations (FDEs) with constant coefficients involving a spectral Galerkin method. The spatial approximation with its fractional order derivatives (described in the Caputo sense) are built using shifted Monic Ultraspherical polynomials.
翻译:本文的目的是展示和解释一个新的公式,该公式以最终方式表明任何程度和任何分序的转离超超球多球多球多球多球多球多球多球多球多球多球多球多球多球多球多球多球多球多球组合的衍生物。我们还为线性或非线性多线性多级分数方程(FDEs)创建了一个直接的方法解决方案,该方程式的常数包含光谱加列金方法。空间近似及其分序衍生物(在Caputo 感知中描述)的衍生物是用转离的超球多球多球多球多球多球多球多球多球多子计算法构建的。