Minimization of Age-of-Information in Remote Sensing with Energy Harvesting

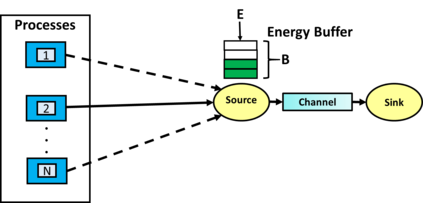
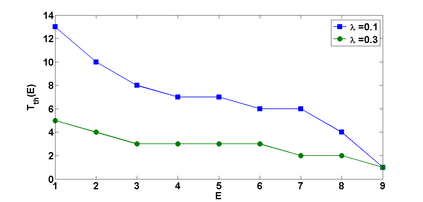
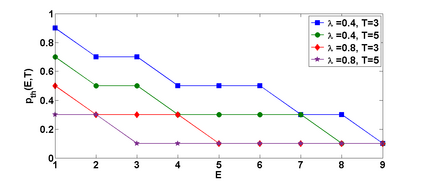
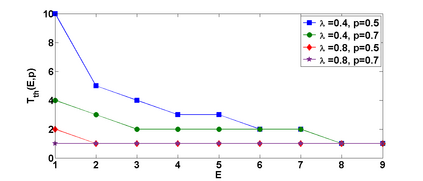
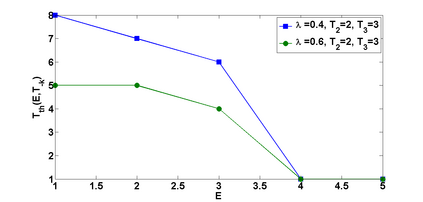
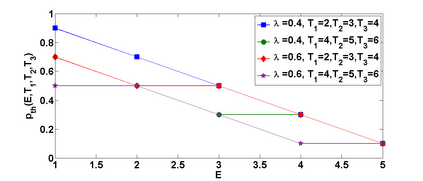
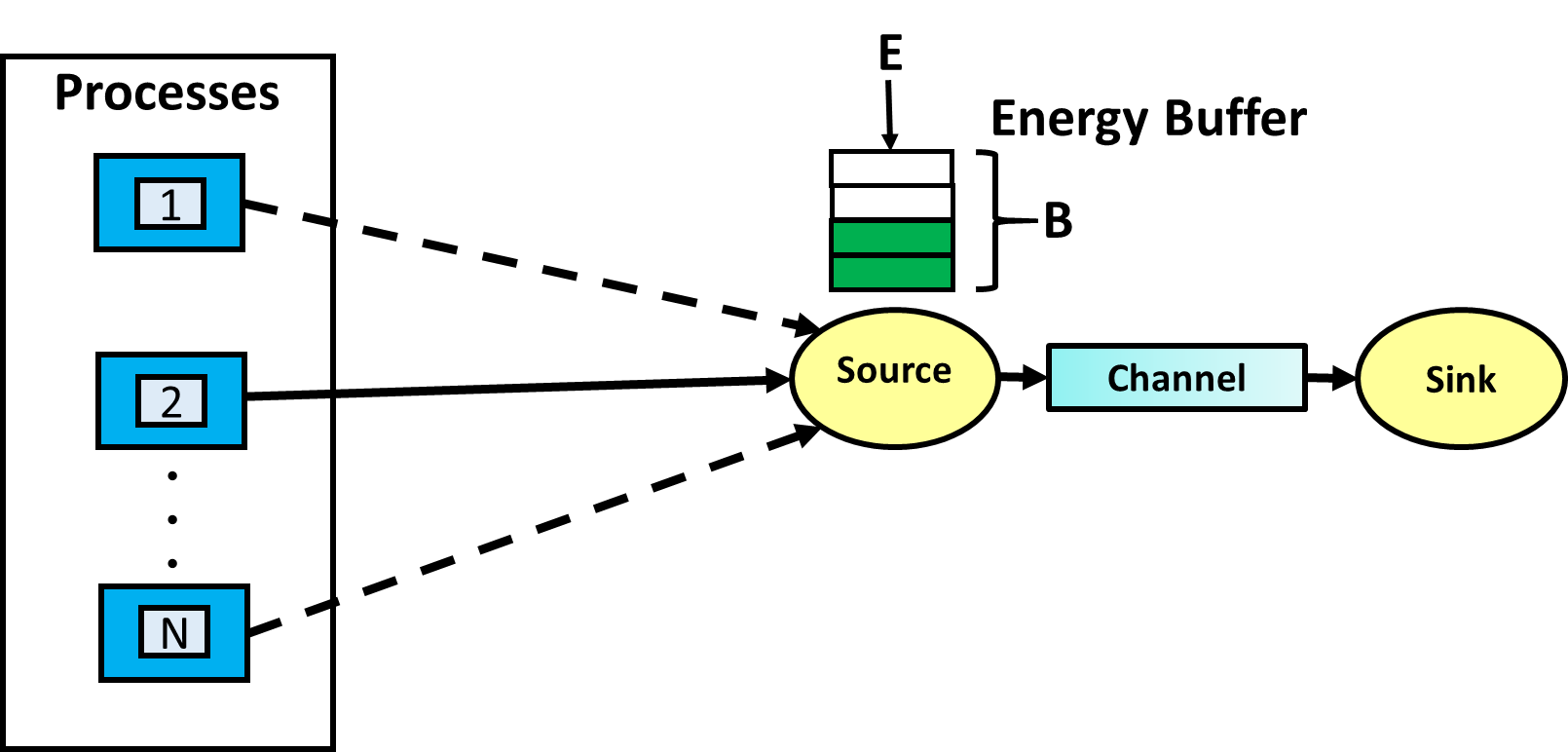
In this paper, minimization of time-averaged age-of-information (AoI) in an energy-harvesting sensor equipped remote sensing setting is considered. An energy harvesting (EH) sensor generates energy packets according to a Bernoulli process at discrete time instants. These energy packets are used by the sensor to make measurements of physical processes and send the observation packets to a remote estimator or a sink node. The trade-off is between the freshness of information available at the sink node and the available energy at the energy buffer of the sensor, which requires the sensor to opportunistically sample and communicate the observations to the sink node. To this end, infinite horizon Markov decision process theory is used to formulate the problem of minimization of time-averaged expected AoI for a single energy harvesting sensor. The following progression of scenarios is considered: (i) single process, perfect communication channel between sensor and sink node, (ii) single process, fading channel with channel state information at transmitter (CSIT), (iii) multiple processes, perfect channel, (iv) multiple processes, fading channel with CSIT. In each scenario, the optimal sensor sampling policy is shown to be a threshold policy involving the instantaneous age of the process, the available energy in the buffer and the instantaneous channel quality as the decision variables. Finally, numerical results are provided to demonstrate the policy structures and trade-offs.