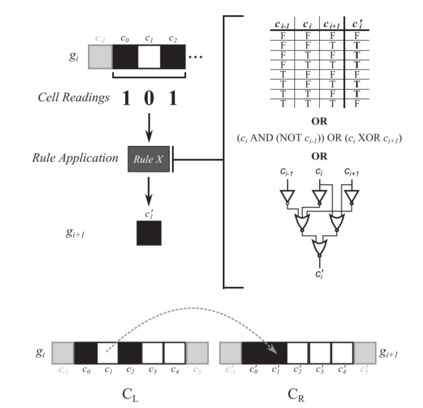
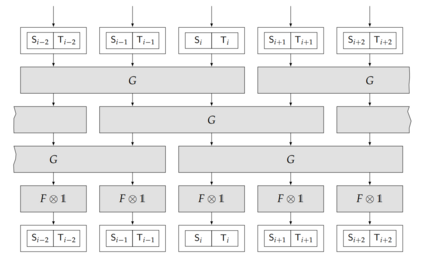
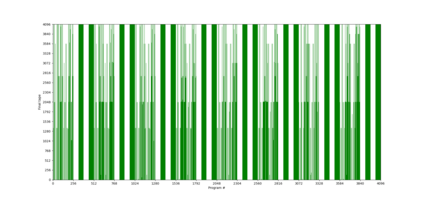
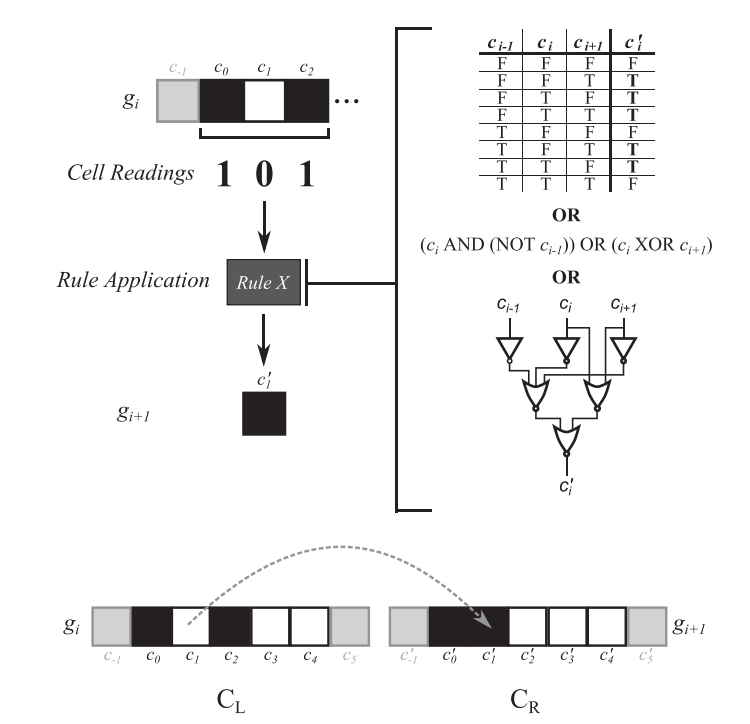
In this research, we present a quantum circuit design and implementation for a parallel universal linear bounded automata. This circuit is able to accelerate the inference of algorithmic structures in data for discovering causal generative models. The computation model is practically restricted in time and space resources. A classical exhaustive enumeration of all possible programs on the automata is shown for a couple of example cases. The precise quantum circuit design that allows executing a superposition of programs, along with a superposition of inputs as in the standard quantum Turing machine formulation, is presented. This is the first time, a superposition of classical automata is implemented on the circuit model of quantum computation, having the corresponding mechanistic parts of a classical Turing machine. The superposition of programs allows our model to be used for experimenting with the space of program-output behaviors in algorithmic information theory. Our implementations on OpenQL and Qiskit quantum programming language is copy-left and is publicly available on GitHub.
翻译:在此研究中,我们展示了平行的通用线性捆绑自动成形器的量子电路设计和实施。 该电路能够加速数据中算法结构的推断, 以发现因果基因模型。 计算模型实际上在时间和空间资源上受到限制。 典型地对自动成形器上的所有可能程序进行详尽的罗列, 用于几个例子。 演示了精确量子电路设计, 允许执行程序叠加, 以及标准量子图灵机配制中输入的叠加。 这是第一次在量子计算电路模型上执行古典自动成形器的叠加, 配有古典型图灵机的相应机械部件。 程序的叠加位置允许我们的模型用于在算法信息理论中实验程序输出行为空间。 我们在 OpenQL 和 Qiskit 量子编程语言上的应用是复制的, 并在 GitHub 上公开提供。