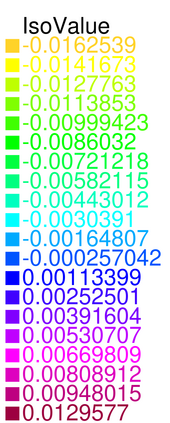
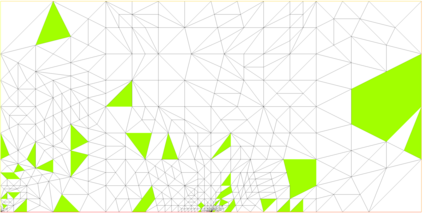
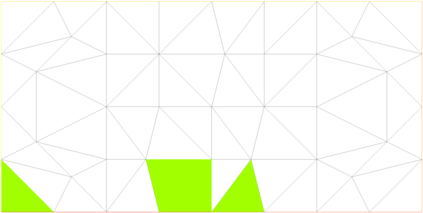
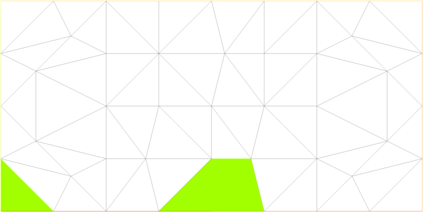
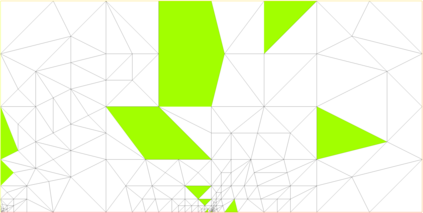
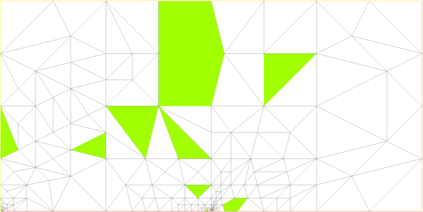
We present an a posteriori error estimate based on equilibrated stress reconstructions for the finite element approximation of a unilateral contact problem with weak enforcement of the contact conditions. We start by proving a guaranteed upper bound for the dual norm of the residual. This norm is shown to control the natural energy norm up to a boundary term, which can be removed under a saturation assumption. The basic estimate is then refined to distinguish the different components of the error, and is used as a starting point to design an algorithm including adaptive stopping criteria for the nonlinear solver and automatic tuning of a regularization parameter. We then discuss an actual way of computing the stress reconstruction based on the Arnold-Falk-Winther finite elements. Finally, after briefly discussing the efficiency of our estimators, we showcase their performance on a panel of numerical tests.
翻译:我们提出一个事后误差估计,其依据是,在接触条件执行不力的情况下,对单方接触问题的有限元素近似值进行了平衡的应激反应重建;我们首先证明剩余物的双重规范具有保障的上限;该规范被证明可以控制自然能源规范,直至边界期,在饱和假设下可以去除;然后对基本估算进行细化,以区分错误的不同组成部分,并用作设计算法的起点,包括非线性求解器的适应性停止标准以及规范参数的自动调整;然后我们讨论根据Arnold-Falk-Winther的限定要素计算压力重建的实际方法;最后,在简要讨论我们的估算器的效率之后,我们在数字测试板上展示其表现。