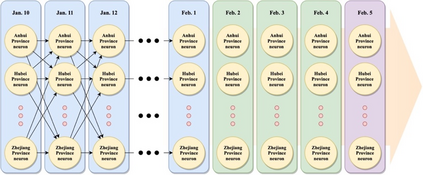
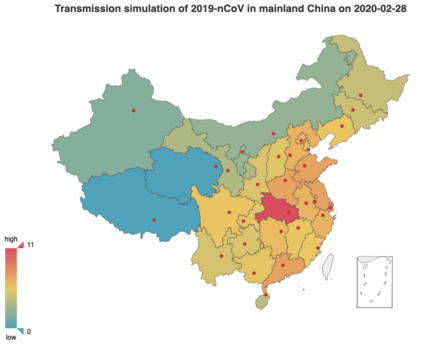
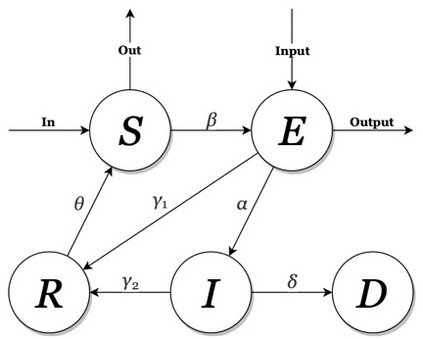
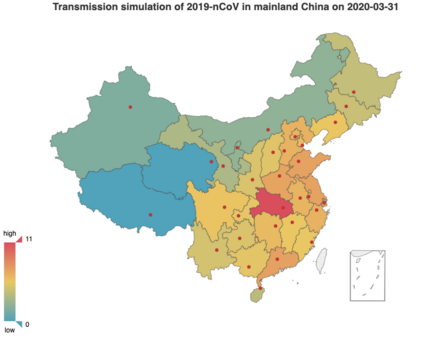
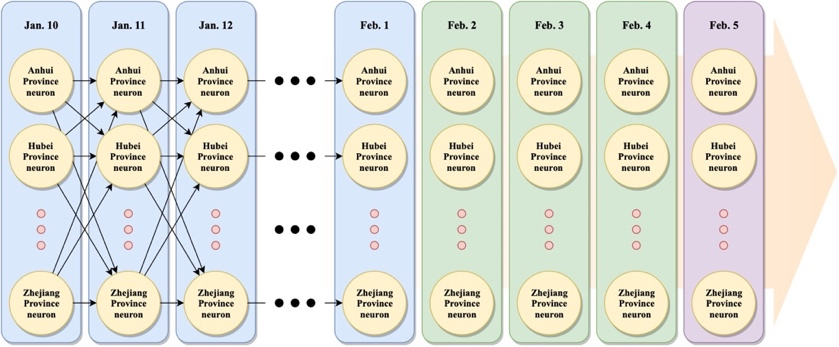
Since the SARS outbreak in 2003, a lot of predictive epidemiological models have been proposed. At the end of 2019, a novel coronavirus, termed as 2019-nCoV, has broken out and is propagating in China and the world. Here we propose a multi-model ordinary differential equation set neural network (MMODEs-NN) and model-free methods to predict the interprovincial transmissions in mainland China, especially those from Hubei Province. Compared with the previously proposed epidemiological models, the proposed network can simulate the transportations with the ODEs activation method, while the model-free methods based on the sigmoid function, Gaussian function, and Poisson distribution are linear and fast to generate reasonable predictions. According to the numerical experiments and the realities, the special policies for controlling the disease are successful in some provinces, and the transmission of the epidemic, whose outbreak time is close to the beginning of China Spring Festival travel rush, is more likely to decelerate before February 18 and to end before April 2020. The proposed mathematical and artificial intelligence methods can give consistent and reasonable predictions of the 2019-nCoV ending. We anticipate our work to be a starting point for comprehensive prediction researches of the 2019-nCoV.
翻译:自2003年SARS疫情爆发以来,提出了许多预测性流行病学模型。2019年底,中国和全世界爆发并传播了被称为2019-nCOV的新型冠状病毒,在中国和世界传播。我们在此建议采用多模型普通差异方程式神经神经网络(MMODEs-NNN)和模型不使用的方法来预测中国大陆的跨省传播,特别是来自湖北省的传播。与先前提议的流行病学模型相比,拟议的网络可以模拟使用ODEs激活方法的运输,而基于Sigmoid函数、Gaussian函数和Poisson分布的无模型方法则是线性且快速生成合理预测。根据数字实验和现实,控制该疾病的特殊政策在一些省份是成功的,而疫情的传播(其爆发时间接近于中国春节旅行的开始时间)则更有可能在2月18日之前和2020年4月之前减速。拟议的数学和人工智能方法可以提供2019-VCO综合预测的连续和合理预测。我们预计的2019-VCO工作将开始阶段的工作。