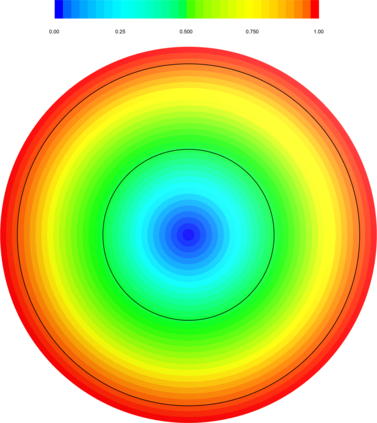
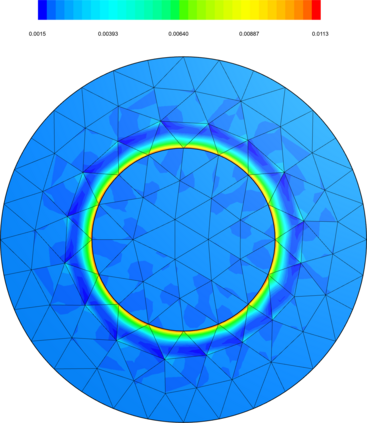
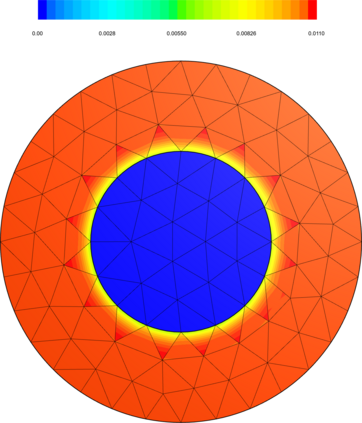
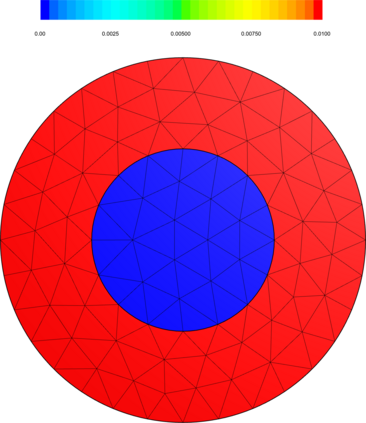
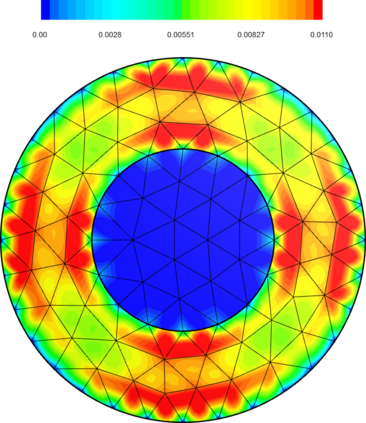
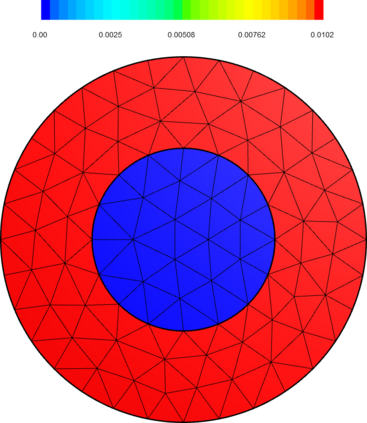
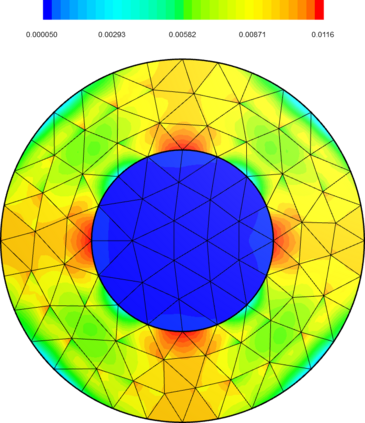
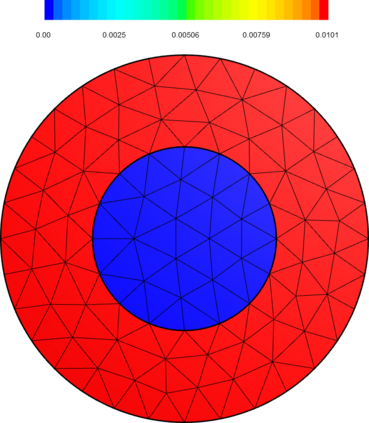
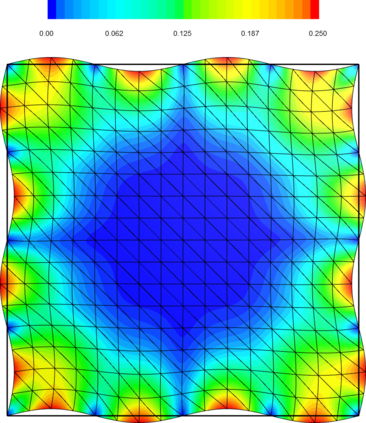
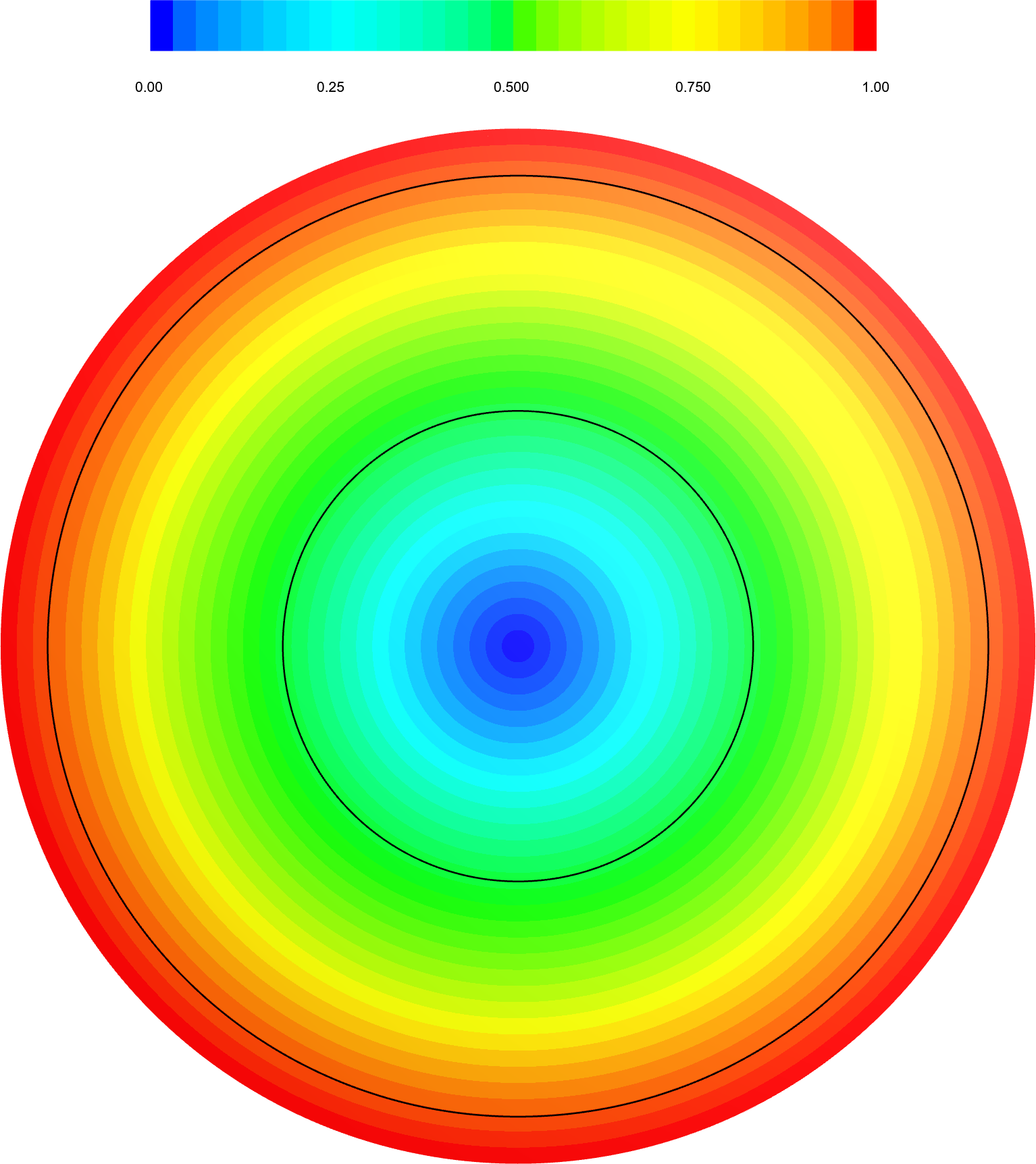
In this work we present a consistent reduction of the relaxed micromorphic model to its corresponding two-dimensional planar model, such that its capacity to capture discontinuous dilatation fields is preserved. As a direct consequence of our approach, new conforming finite elements for $H^\mathrm{dev}(\mathrm{Curl},A)$ become necessary. We present two novel $H^\mathrm{dev}(\mathrm{Curl},A)$-conforming finite element spaces, of which one is a macro element based on Clough--Tocher splits, as well as primal and mixed variational formulations of the planar relaxed micromorphic model. Finally, we demonstrate the effectiveness of our approach with two numerical examples.
翻译:暂无翻译
相关内容
ACM/IEEE第23届模型驱动工程语言和系统国际会议,是模型驱动软件和系统工程的首要会议系列,由ACM-SIGSOFT和IEEE-TCSE支持组织。自1998年以来,模型涵盖了建模的各个方面,从语言和方法到工具和应用程序。模特的参加者来自不同的背景,包括研究人员、学者、工程师和工业专业人士。MODELS 2019是一个论坛,参与者可以围绕建模和模型驱动的软件和系统交流前沿研究成果和创新实践经验。今年的版本将为建模社区提供进一步推进建模基础的机会,并在网络物理系统、嵌入式系统、社会技术系统、云计算、大数据、机器学习、安全、开源等新兴领域提出建模的创新应用以及可持续性。
官网链接:http://www.modelsconference.org/
专知会员服务
33+阅读 · 2020年4月26日
专知会员服务
34+阅读 · 2020年1月15日
专知会员服务
36+阅读 · 2019年10月17日
Arxiv
0+阅读 · 2024年7月1日