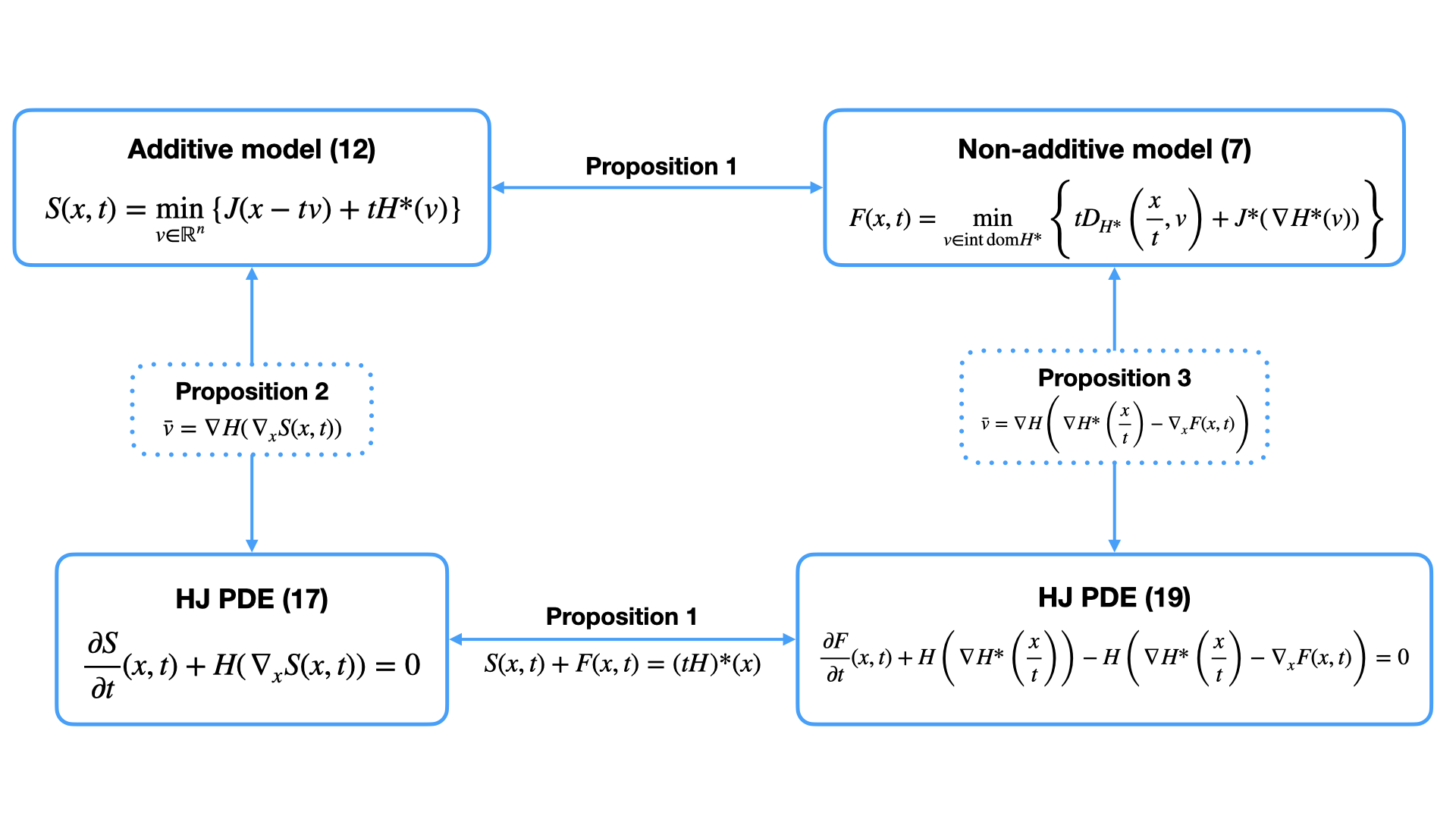
We consider image denoising problems formulated as variational problems. It is known that Hamilton-Jacobi PDEs govern the solution of such optimization problems when the noise model is additive. In this work, we address certain non-additive noise models and show that they are also related to Hamilton-Jacobi PDEs. These findings allow us to establish new connections between additive and non-additive noise imaging models. With these connections, some non-convex models for non-additive noise can be solved by applying convex optimization algorithms to the equivalent convex models for additive noise. Several numerical results are provided for denoising problems with Poisson noise or multiplicative noise.
翻译:我们认为,将图像脱色问题视为变异问题,已知汉密尔顿-Jacobi PDEs在噪声模型是添加剂时会决定这种优化问题的解决方案。在这项工作中,我们处理某些非添加型噪声模型,并表明它们也与汉密尔顿-Jacobi PDEs有关。这些发现使我们能够在添加型和非添加型噪声成像模型之间建立新的联系。有了这些联系,一些非添加型噪声的非碳化型模型可以通过对等的添加型噪声的convex优化算法来解决。为Poisson噪声或倍增噪的解调问题提供了若干数字结果。