Comparing the effectiveness of k-different treatments through the area under the ROC curve

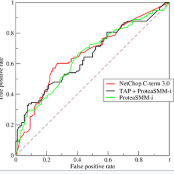
The area under the receiver-operating characteristic curve (AUC) has become a popular index not only for measuring the overall prediction capacity of a marker but also the association strength between continuous and binary variables. In the current study, it has been used for comparing the association size of four different interventions involving impulsive decision making, studied through an animal model, in which each animal provides several negative (pre-treatment) and positive (post-treatment) measures. The problem of the full comparison of the average AUCs arises therefore in a natural way. We construct an analysis of variance (ANOVA) type test for testing the equality of the impact of these treatments measured through the respective AUCs, and considering the random-effect represented by the animal. The use (and development) of a post-hoc Tukey's HSD type test is also considered. We explore the finite-sample behavior of our proposal via Monte Carlo simulations, and analyze the data generated from the original problem. An R package implementing the procedures is provided as supplementary material.