Energy dissipation of Strang splitting for Allen--Cahn

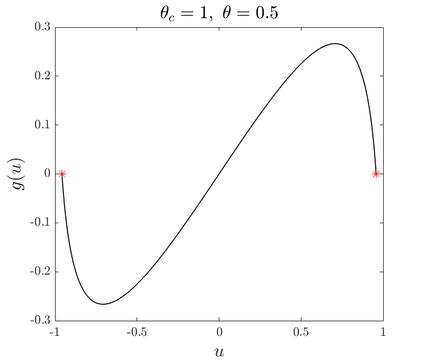
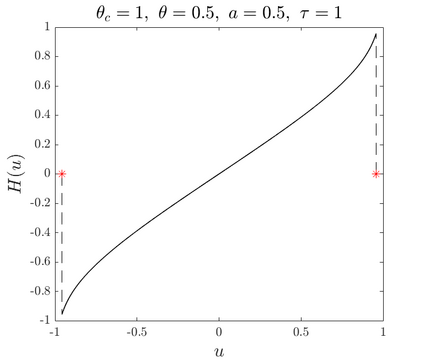
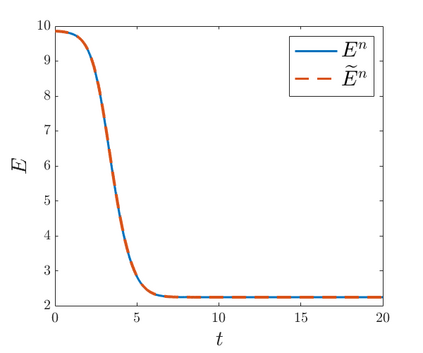
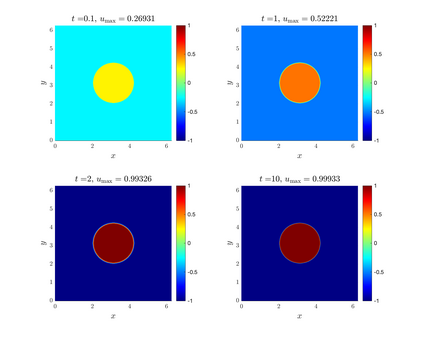
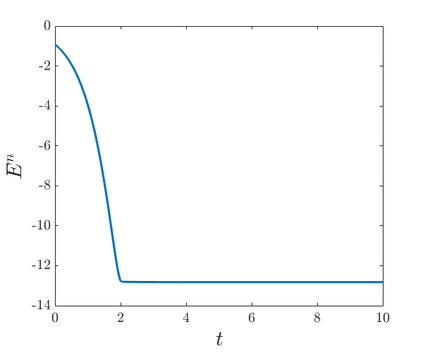
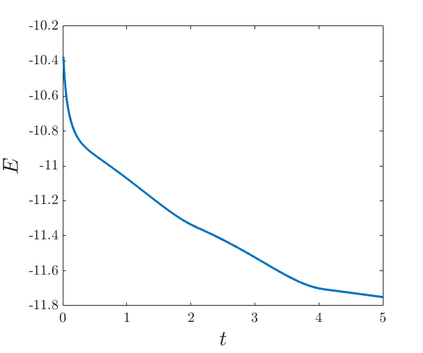
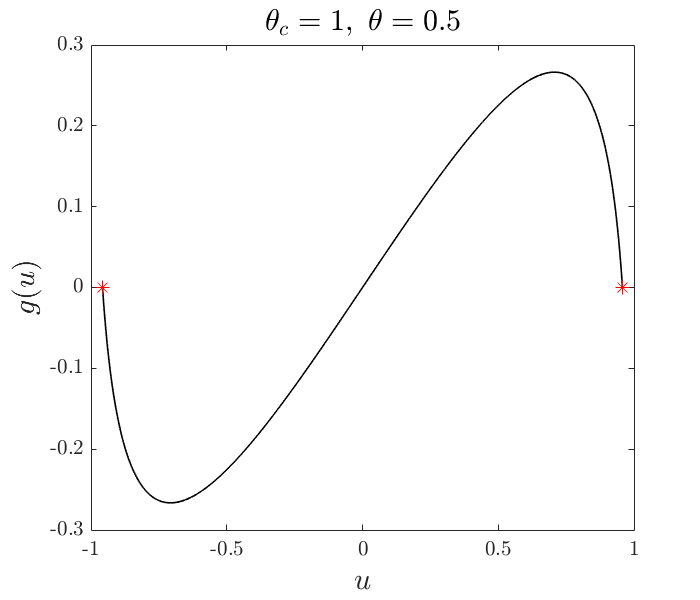
We consider a class of second-order Strang splitting methods for Allen-Cahn equations with polynomial or logarithmic nonlinearities. For the polynomial case both the linear and the nonlinear propagators are computed explicitly. We show that this type of Strang splitting scheme is unconditionally stable regardless of the time step. Moreover we establish strict energy dissipation for a judiciously modified energy which coincides with the classical energy up to $\mathcal O(\tau)$ where $\tau$ is the time step. For the logarithmic potential case, since the continuous-time nonlinear propagator no longer enjoys explicit analytic treatments, we employ a second order in time two-stage implicit Runge--Kutta (RK) nonlinear propagator together with an efficient Newton iterative solver. We prove a maximum principle which ensures phase separation and establish energy dissipation law under mild restrictions on the time step. These appear to be the first rigorous results on the energy dissipation of Strang-type splitting methods for Allen-Cahn equations.