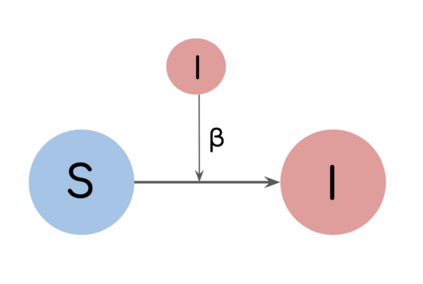
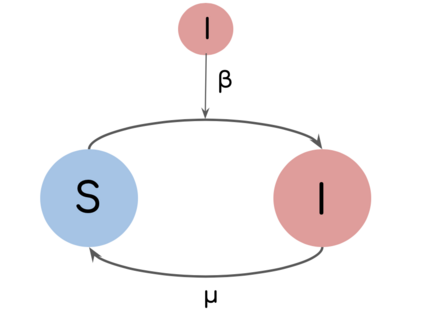
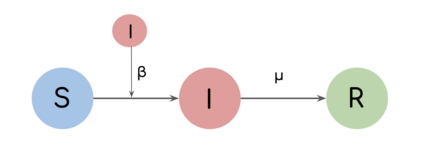
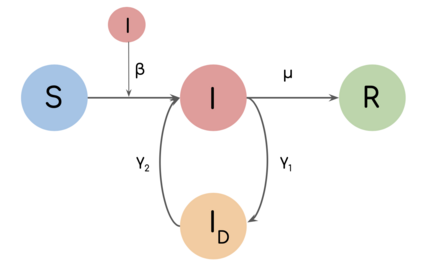
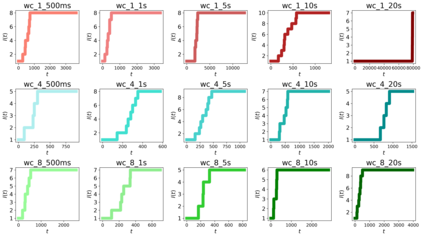
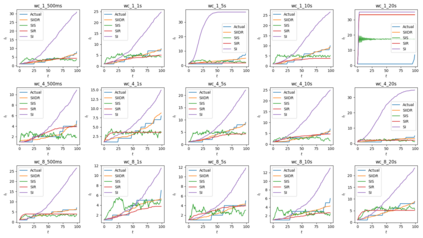
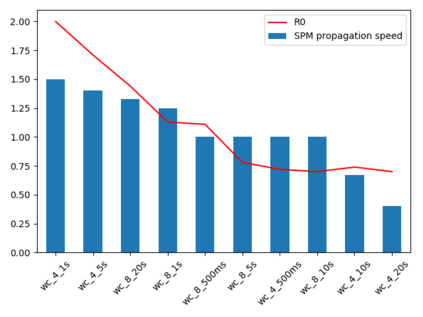
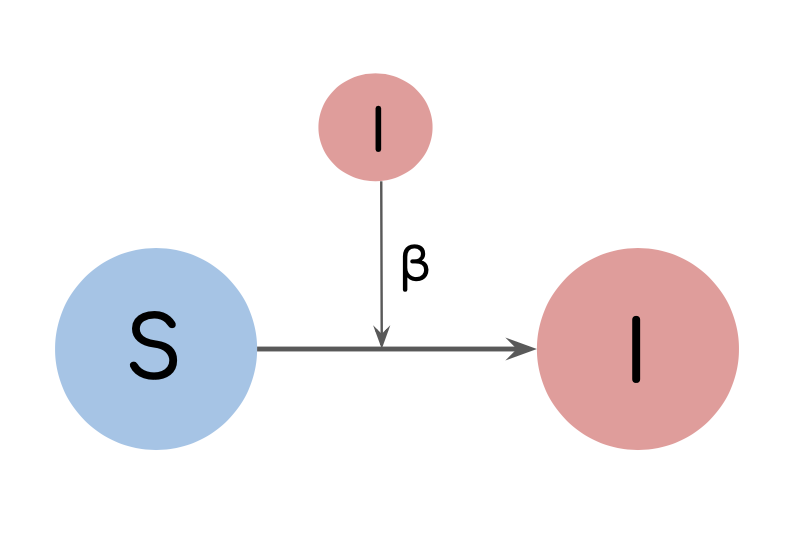
Self-propagating malware (SPM) has recently resulted in large financial losses and high social impact, with well-known campaigns such as WannaCry and Colonial Pipeline being able to propagate rapidly on the Internet and cause service disruptions. To date, the propagation behavior of SPM is still not well understood, resulting in the difficulty of defending against these cyber threats. To address this gap, in this paper we perform a comprehensive analysis of a newly proposed epidemiological model for SPM propagation, Susceptible-Infected-Infected Dormant-Recovered (SIIDR). We perform a theoretical analysis of the stability of the SIIDR model and derive its basic reproduction number by representing it as a system of Ordinary Differential Equations with continuous time. We obtain access to 15 WananCry attack traces generated under various conditions, derive the model's transition rates, and show that SIIDR fits best the real data. We find that the SIIDR model outperforms more established compartmental models from epidemiology, such as SI, SIS, and SIR, at modeling SPM propagation.
翻译:自我宣传的恶意软件(SPM)最近造成了巨大的财政损失和巨大的社会影响,著名的运动,如《WantaCry》和《殖民地管道》等,能够在因特网上迅速传播,并造成服务中断。迄今为止,SPM的传播行为仍然没有得到很好地理解,因此难以防范这些网络威胁。为弥补这一差距,我们在本文件中全面分析了新提议的SPM传播流行病学模型,即可感知感染的Dormant-Recovered(SIIDR),我们对SIID模型的稳定性进行了理论分析,并得出其基本复制数字,将SIIDR模型代表成一个具有持续时间的普通差异分布的系统。我们获得了在各种条件下产生的15个WanCry攻击痕迹,得出模型的过渡率,并表明SIIDR最符合真实数据。我们发现,SIIDR模型比SIS、SIIS和SIR等流行病学更固定的分包模型,在SPM传播模型上建模。