A novel approach to asteroid impact monitoring and hazard assessment

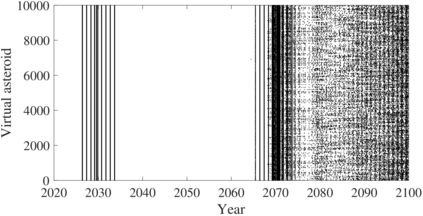
Orbit-determination programs find the orbit solution that best fits a set of observations by minimizing the RMS of the residuals of the fit. For near-Earth asteroids, the uncertainty of the orbit solution may be compatible with trajectories that impact Earth. This paper shows how incorporating the impact condition as an observation in the orbit-determination process results in a robust technique for finding the regions in parameter space leading to impacts. The impact pseudo-observation residuals are the b-plane coordinates at the time of close approach and the uncertainty is set to a fraction of the Earth radius. The extended orbit-determination filter converges naturally to an impacting solution if allowed by the observations. The uncertainty of the resulting orbit provides an excellent geometric representation of the virtual impactor. As a result, the impact probability can be efficiently estimated by exploring this region in parameter space using importance sampling. The proposed technique can systematically handle a large number of estimated parameters, account for nongravitational forces, deal with nonlinearities, and correct for non-Gaussian initial uncertainty distributions. The algorithm has been implemented into a new impact monitoring system at JPL called Sentry-II, which is undergoing extensive testing. The main advantages of Sentry-II over JPL's currently operating impact monitoring system Sentry are that Sentry-II can systematically process orbits perturbed by nongravitational forces and that it is generally more robust when dealing with pathological cases. The runtimes and completeness of both systems are comparable, with the impact probability of Sentry-II for 99% completeness being $3\times10^{-7}$.