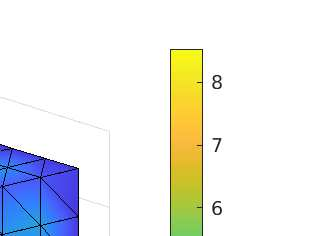
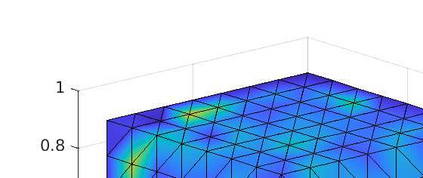
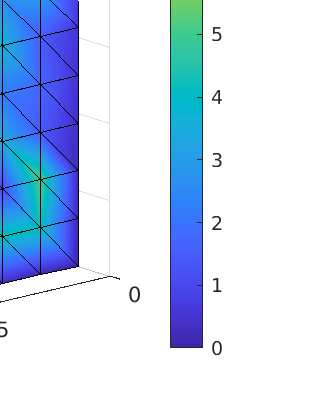
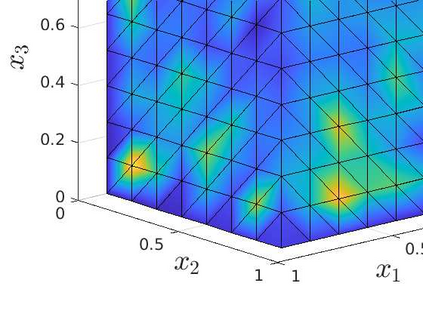
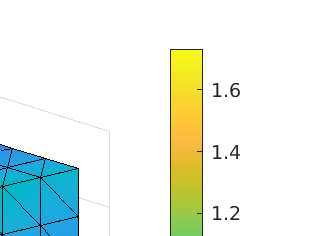
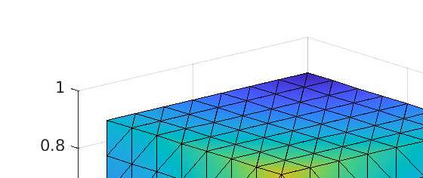
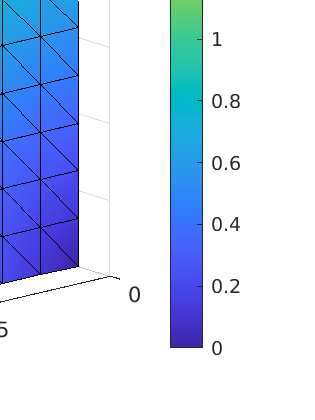
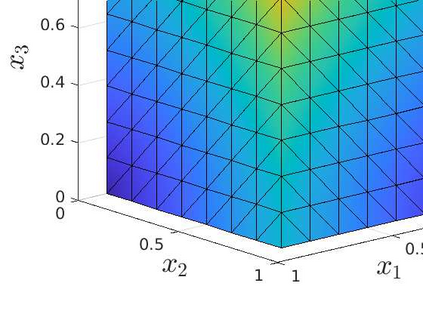
This work proposes a new stabilized $P_1\times P_0$ finite element method for solving the incompressible Navier--Stokes equations. The numerical scheme is based on a reduced Bernardi--Raugel element with statically condensed face bubbles and is pressure-robust in the small viscosity regime. For the Stokes problem, an error estimate uniform with respect to the kinematic viscosity is shown. For the Navier--Stokes equation, the nonlinear convection term is discretized using an edge-averaged finite element method. In comparison with classical schemes, the proposed method does not require tuning of parameters and is validated for competitiveness on several benchmark problems in 2 and 3 dimensional space.
翻译:这项工作提出了一个新的稳定的 $P_ 1\time P_ 0$ 有限元素方法, 用于解决无法压缩的导航- Stokes 方程式。 数字方法基于一个使用静电压缩面泡的Bernardi- Raugel 元素, 以静态压缩面泡为基数, 是小型粘度系统中的压力- 硬质。 对于斯托克斯 问题, 显示一个与运动相对立性一致的错误估计。 对于纳维- Stokes 方程式, 非线性对流术语使用边缘平均有限元素法分离。 与经典方法相比, 拟议的方法不需要调整参数, 并被验证为在 2 和 3 维空间的若干基准问题上的竞争力 。