Calderón Preconditioning for Acoustic Scattering at Multi-Screens

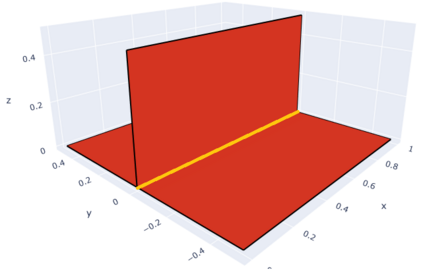
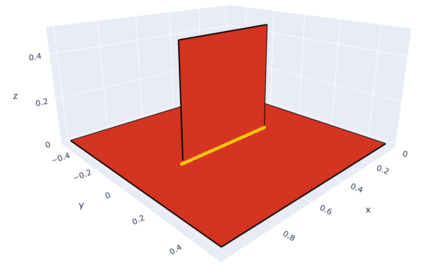
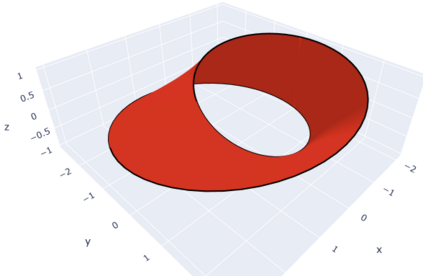
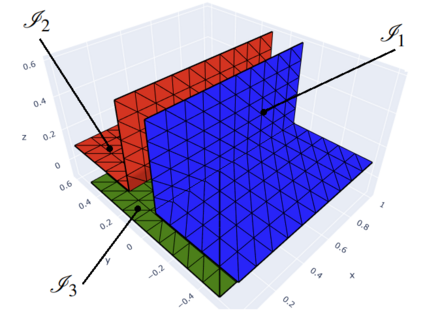
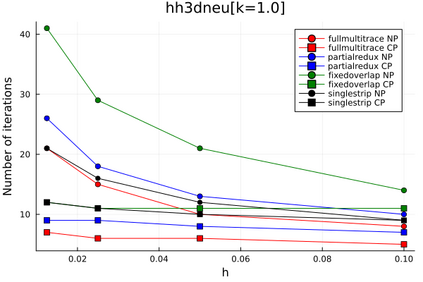
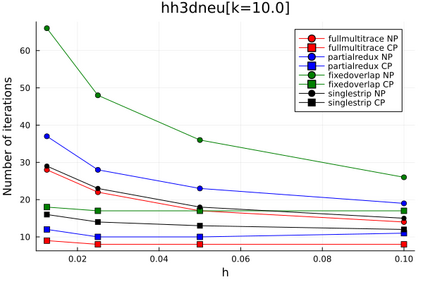
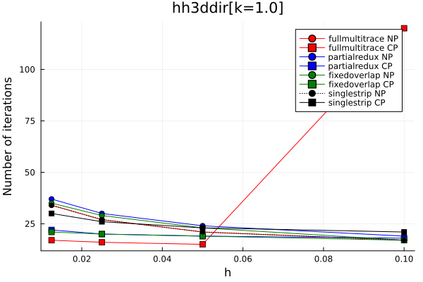
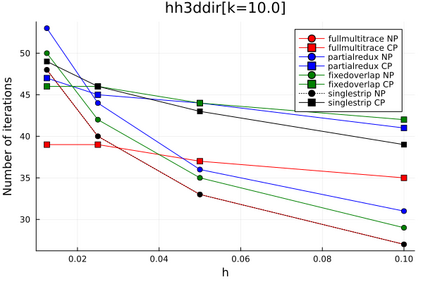
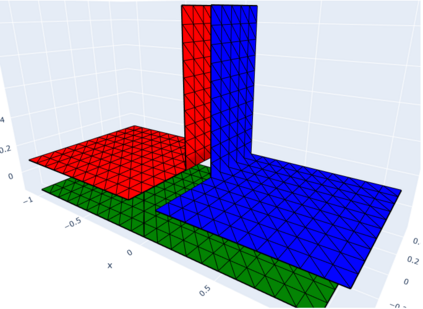
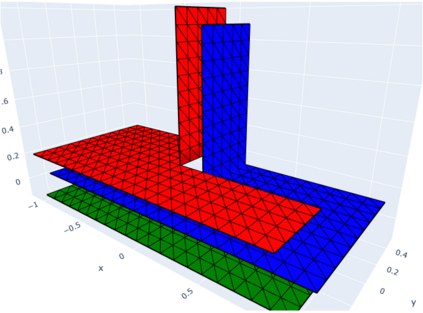
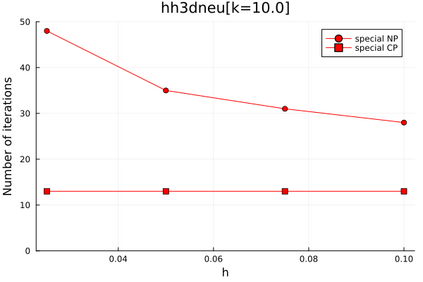
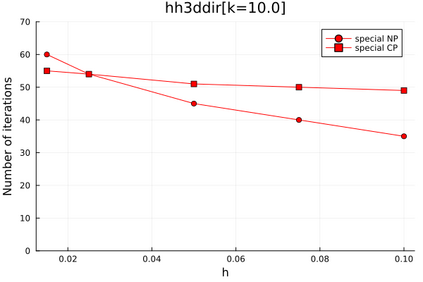
We propose a preconditioner for the Helmholtz exterior problems on multi-screens. For this, we combine quotient-space BEM and operator preconditioning. For a class of multi-screens (which we dub \emph{type A} multi-screens), we show that this approach leads to block diagonal Calder\'on preconditioners and results in a spectral condition number that grows only logarithmically with $h$, just as in the case of simple screens. Since the resulting scheme contains many more DoFs than strictly required, we also present strategies to remove almost all redundancy without significant loss of effectiveness of the preconditioner. We verify these findings by providing representative numerical results. Further numerical experiments suggest that these results can be extended beyond type A multi-screens and that the numerical method introduced here can be applied to essentially all multi-screens encountered by the practitioner, leading to a significantly reduced simulation cost.