Smooth Surfaces via Nets of Geodesics

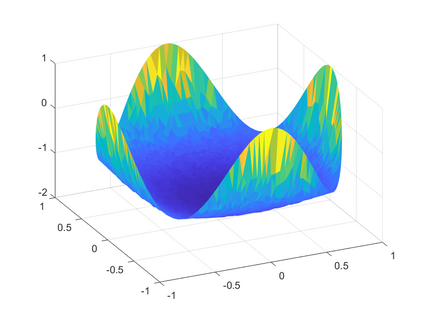
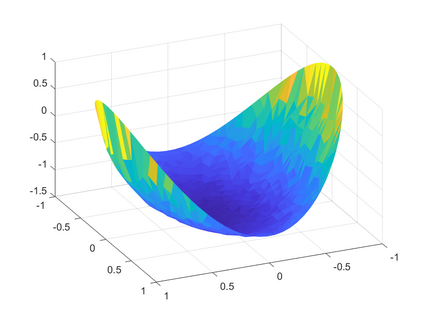
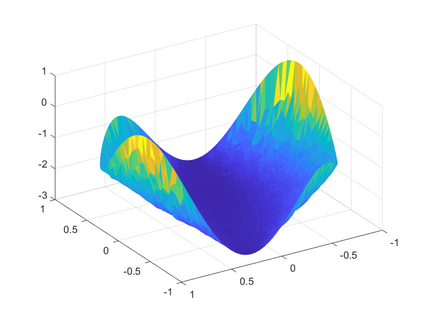
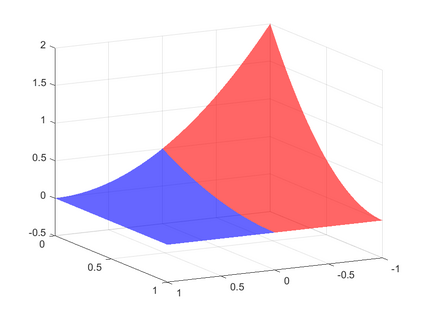
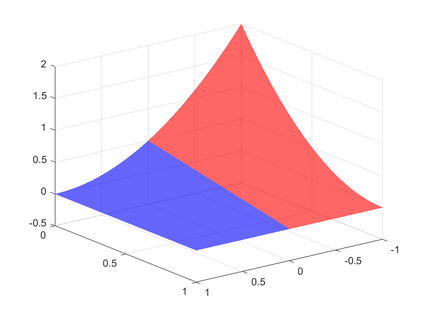
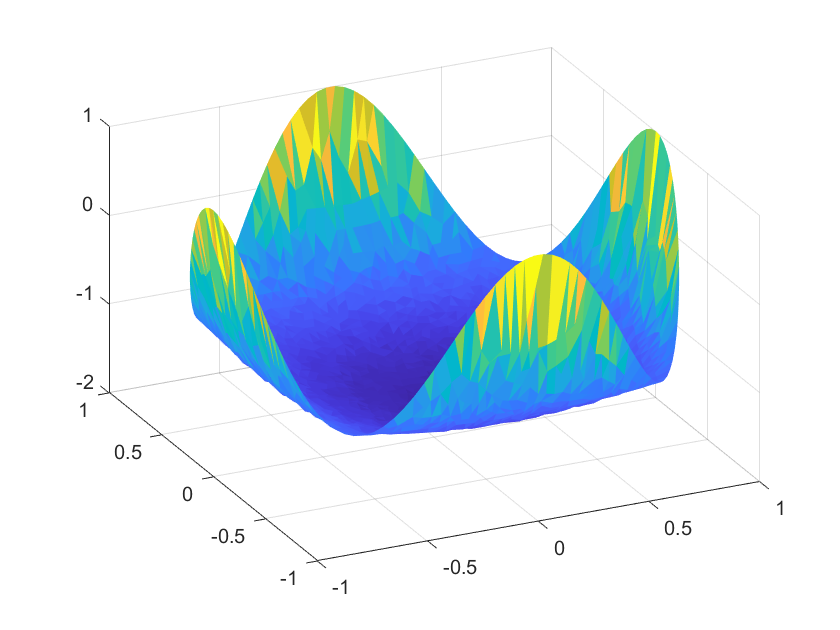
This work presents an algorithm for the computation and visualization of an underlying unknown surface from a given net of geodesics. It is based on a theoretical result by the author regarding minimal Gaussian curvature surfaces with geodesic boundary conditions. The novelty of the method is that it consists of the computation of each patch in the net independently with the union of the patches being a smooth surface. This complements a seminal work by the late David Knill, which suggests that the human visual system infers different objects by markings along geodesics on their surface.