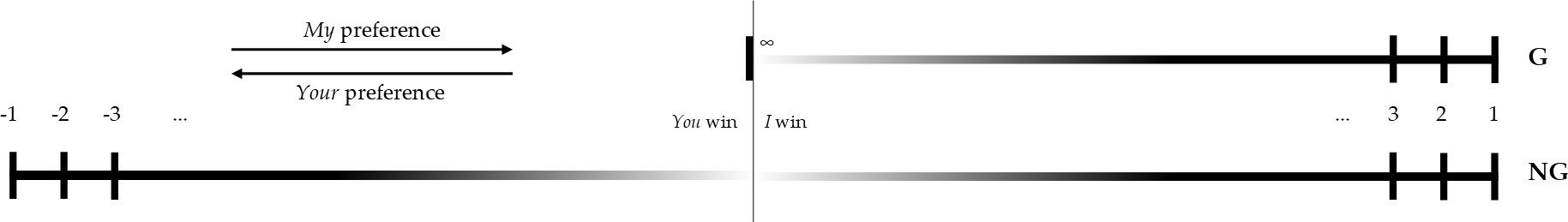
In this paper, we introduce game-theoretic semantics (GTS) for Qualitative Choice Logic (QCL), which, in order to express preferences, extends classical propositional logic with an additional connective called ordered disjunction. Firstly, we demonstrate that game semantics can capture existing degree-based semantics for QCL in a natural way. Secondly, we show that game semantics can be leveraged to derive new semantics for the language of QCL. In particular, we present a new semantics that makes use of GTS negation and, by doing so, avoids problems with negation in existing QCL-semantics.
翻译:在本文中,我们为定性选择逻辑(QCL)引入了游戏理论语义学(GTS),它为表达偏好,扩展了古典理论逻辑,并增加了连接性,称为命令脱节。首先,我们证明游戏语义学可以自然地捕捉QCL现有的基于学位的语义学。第二,我们证明可以利用游戏语义学来为QCL语言产生新的语义学。特别是,我们提出了一个新的语义学学,利用GTS的否定,从而避免了现有的QCL-semanics的否定问题。