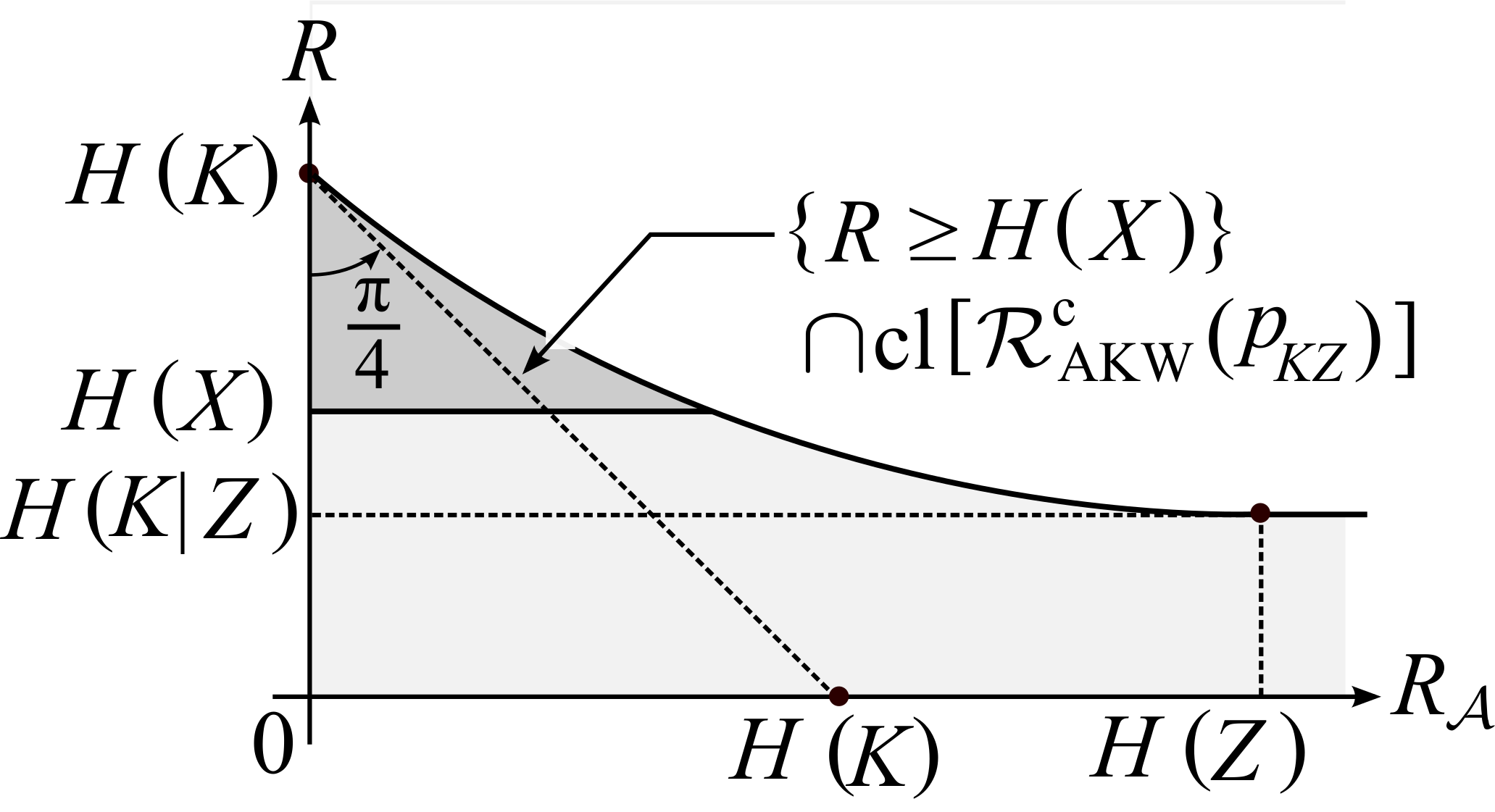
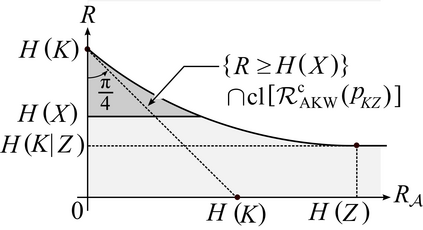
We are interested in investigating the security of source encryption with a symmetric key under side-channel attacks. In this paper, we propose a general framework of source encryption with a symmetric key under the side-channel attacks, which applies to \emph{any} source encryption with a symmetric key and \emph{any} kind of side-channel attacks targeting the secret key. We also propose a new security criterion for strong secrecy under side-channel attacks, which is a natural extension of mutual information, i.e., \emph{the maximum conditional mutual information between the plaintext and the ciphertext given the adversarial key leakage, where the maximum is taken over all possible plaintext distribution}. Under this new criterion, we successfully formulate the rate region, which serves as both necessary and sufficient conditions to have secure transmission even under side-channel attacks. Furthermore, we also prove another theoretical result on our new security criterion, which might be interesting in its own right: in the case of the discrete memoryless source, no perfect secrecy under side-channel attacks in the standard security criterion, i.e., the ordinary mutual information, is achievable without achieving perfect secrecy in this new security criterion, although our new security criterion is more strict than the standard security criterion.
翻译:我们有兴趣在侧道攻击中用对称钥匙调查源加密的安全性。 在本文中,我们提议了一个在侧道攻击中用对称钥匙进行源加密的一般框架,在侧道攻击中用对称钥匙进行源加密,这个框架适用于对称钥匙进行源加密,以及以对称钥匙为目标的侧道攻击。我们还提出了在侧道攻击中以对称钥匙进行严格保密的新的安全性标准。我们还提出了在侧道攻击中采用严格保密的又一个理论性结果,这是相互信息的一个自然延伸,即:在对称钥匙泄漏的情况下,平面和对称钥匙渗漏情况下,对源加密的最大有条件的相互信息。在对称关键线攻击中,对准钥匙的所有可能的对称密。根据这一新标准,我们成功地制定了费率区域,它既是必要又充分的条件,即使在侧道钥匙攻击中也能保证传输。 此外,我们还证明了我们新的安全标准的另一个理论性结果,它本身可能令人感兴趣:在离散的源中,在侧道攻击下没有完全的相互保密性信息,在对准钥匙攻击下没有完全的保密性保密性,而在标准中,尽管这个标准是新的安全标准中,但是在新的标准中,实现共同安全性标准是可实现的。 。在新的安全标准是新的标准。在新的标准。 。在新的安全性标准中,在新的安全性标准是新的标准是安全的性标准。