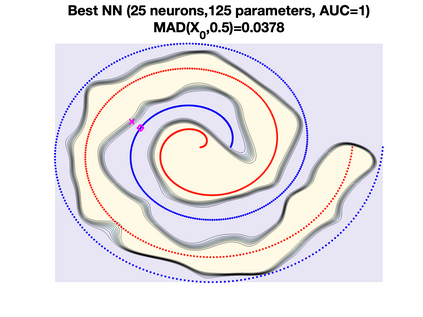
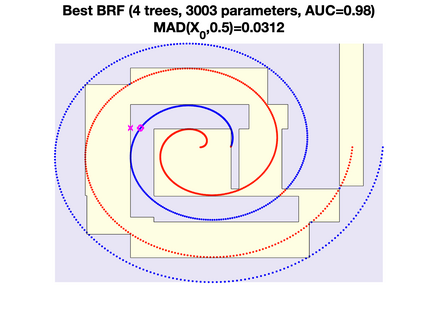
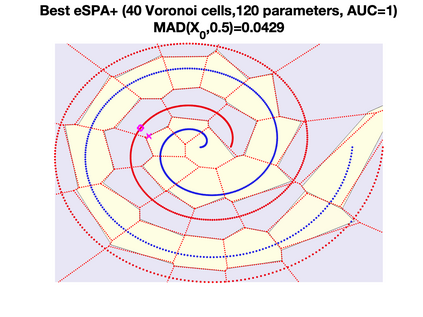
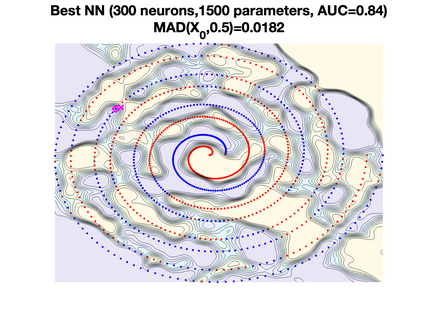
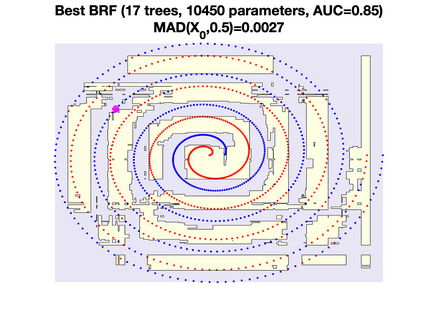
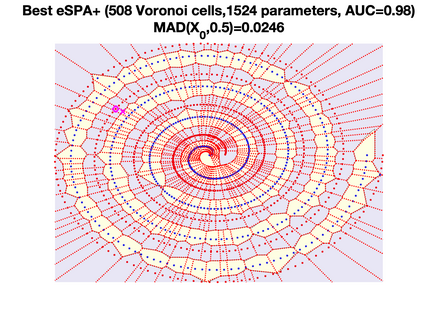
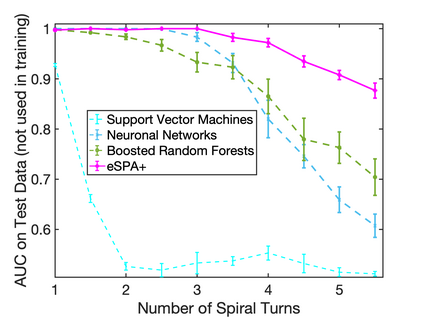
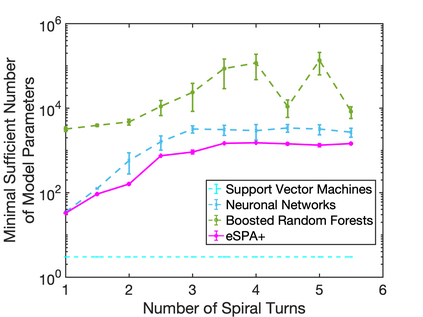
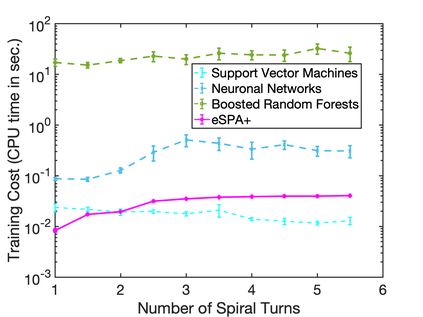
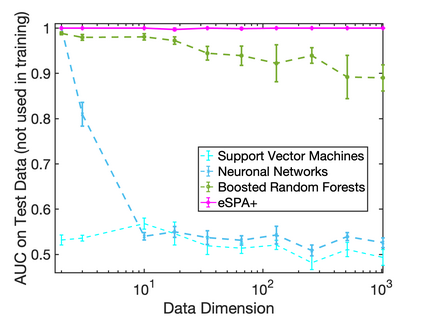
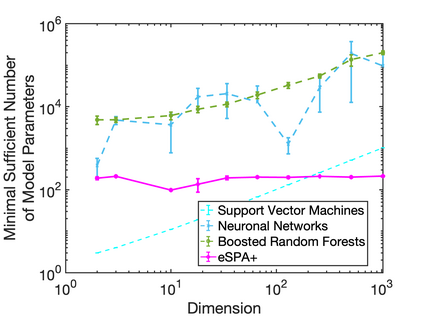
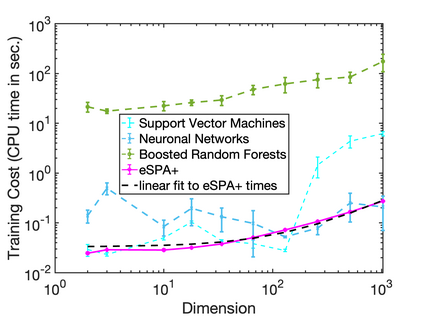
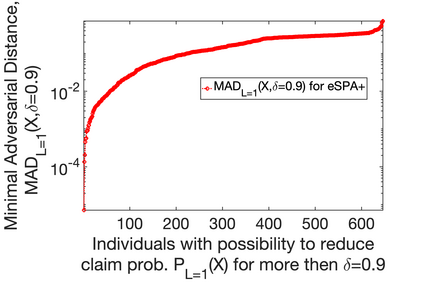
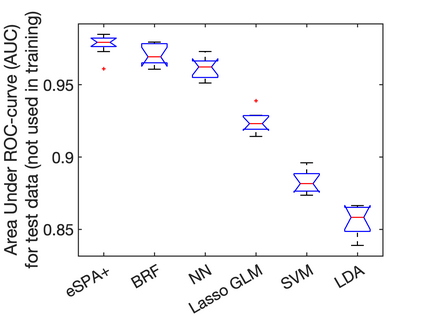
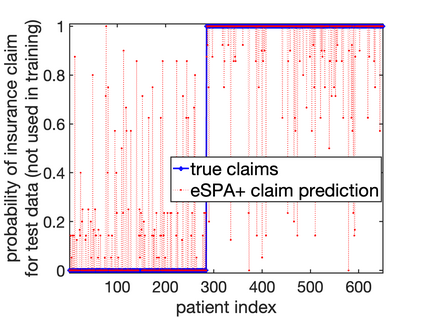
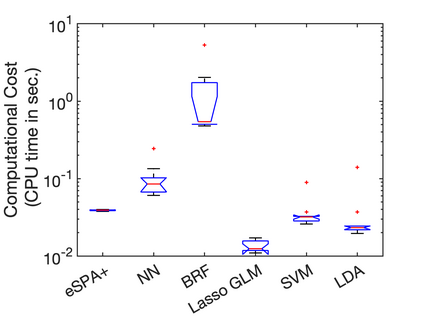
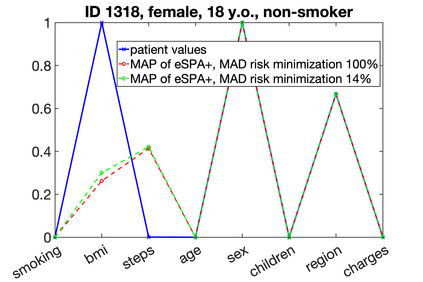
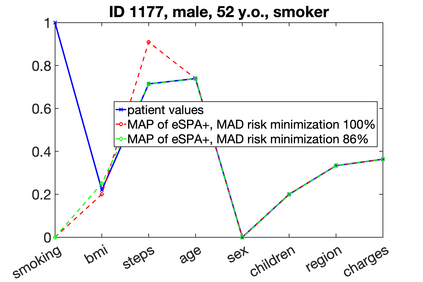
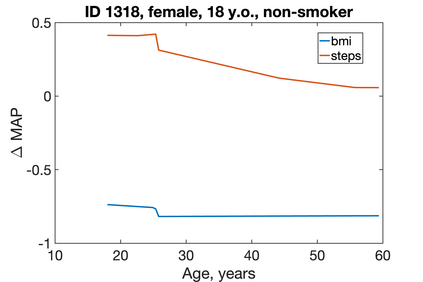
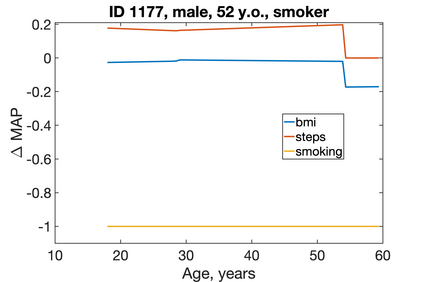
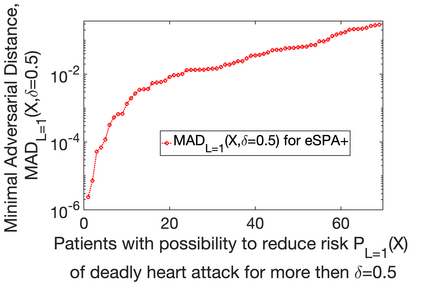
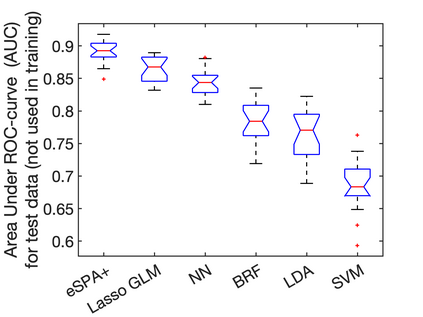
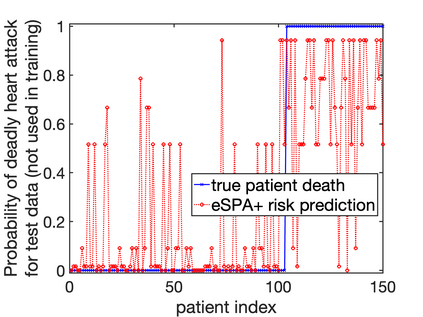
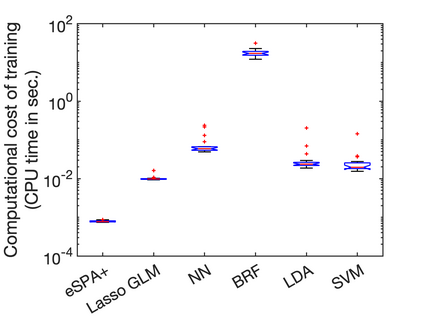
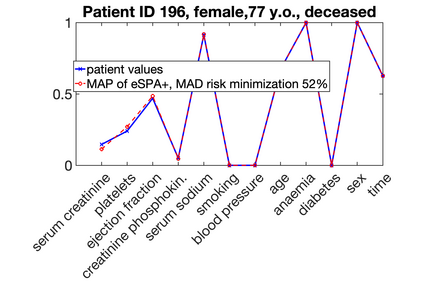
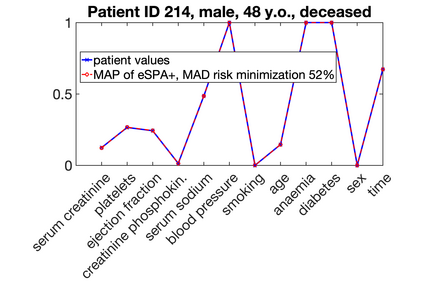
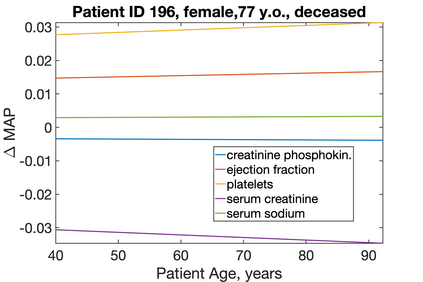
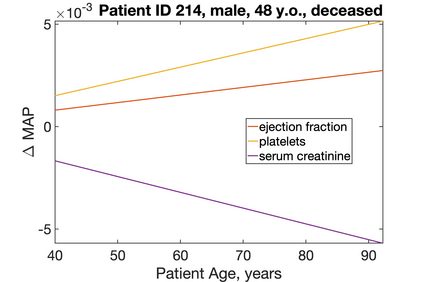
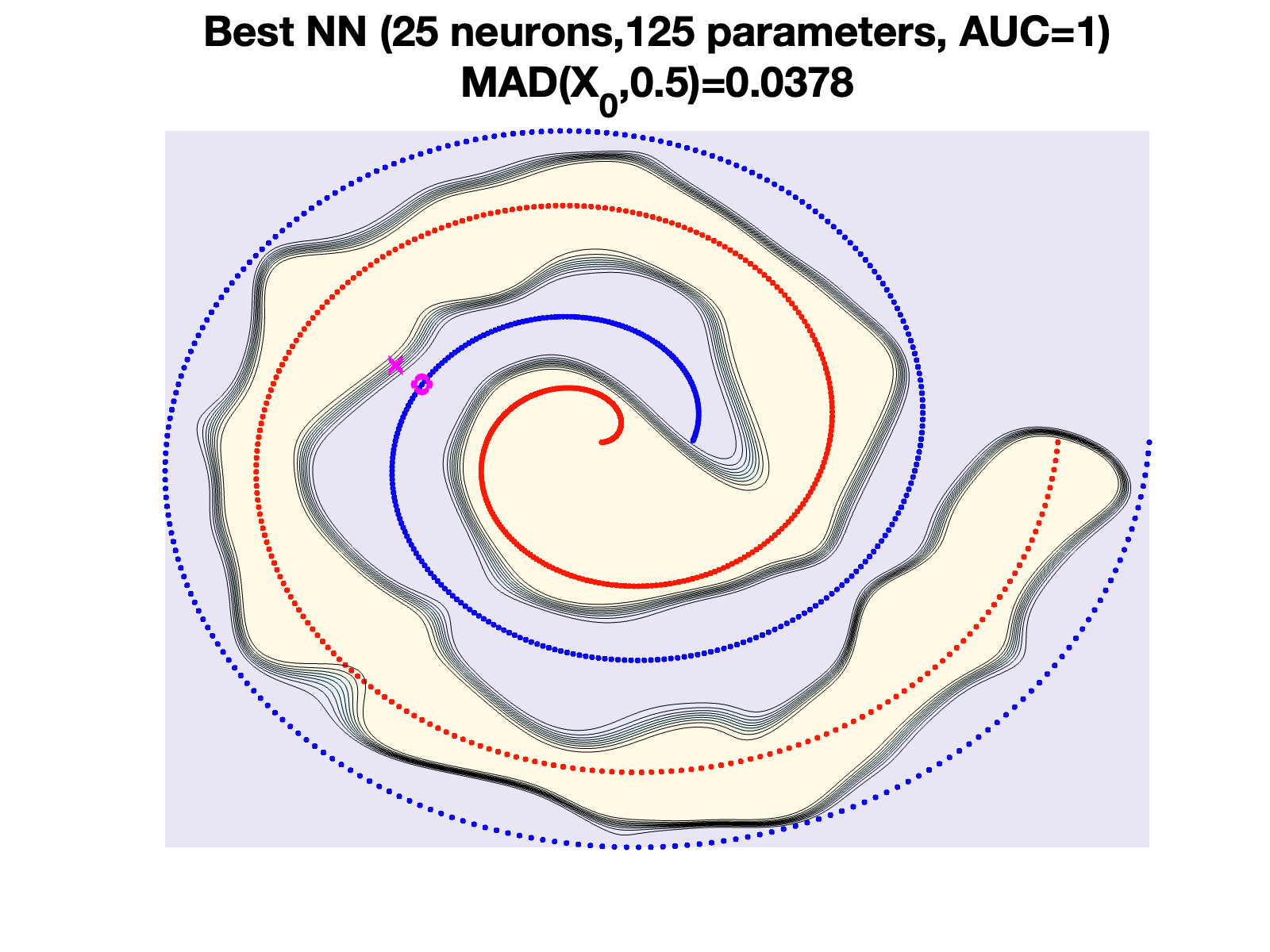
Simply-verifiable mathematical conditions for existence, uniqueness and explicit analytical computation of minimal adversarial paths (MAP) and minimal adversarial distances (MAD) for (locally) uniquely-invertible classifiers, for generalized linear models (GLM), and for entropic AI (EAI) are formulated and proven. Practical computation of MAP and MAD, their comparison and interpretations for various classes of AI tools (for neuronal networks, boosted random forests, GLM and EAI) are demonstrated on the common synthetic benchmarks: on a double Swiss roll spiral and its extensions, as well as on the two biomedical data problems (for the health insurance claim predictions, and for the heart attack lethality classification). On biomedical applications it is demonstrated how MAP provides unique minimal patient-specific risk-mitigating interventions in the predefined subsets of accessible control variables.
翻译:暂无翻译