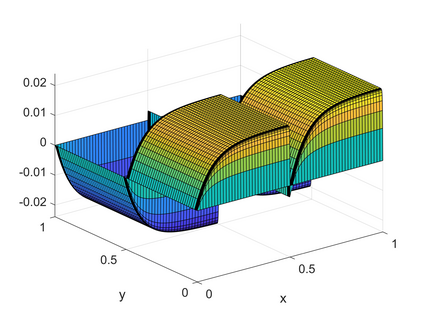
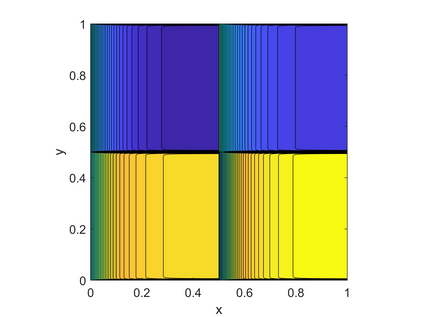
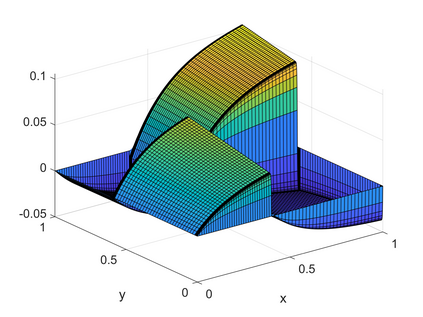
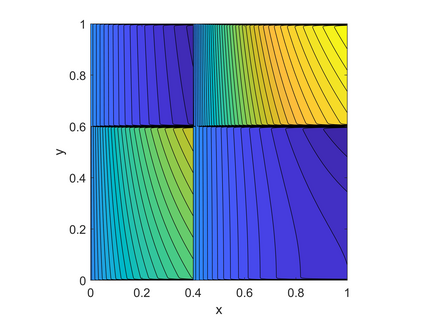
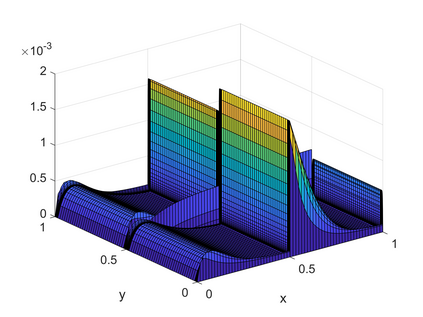
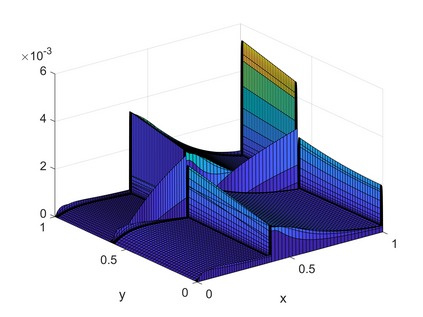
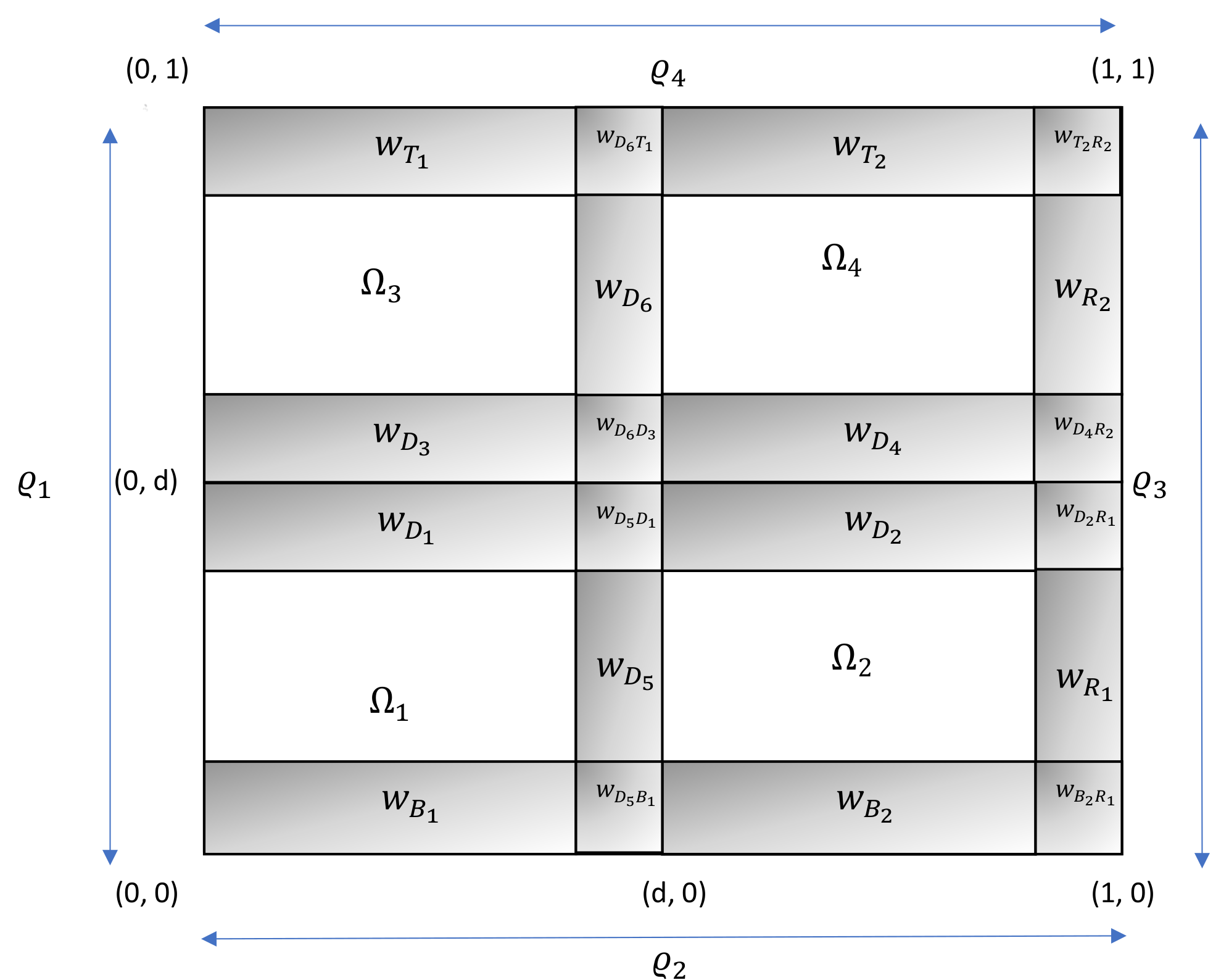
We propose a finite difference scheme for the numerical solution of a two-dimensional singularly perturbed convection-diffusion partial differential equation whose solution features interacting boundary and interior layers, the latter due to discontinuities in source term. The problem is posed on the unit square. The second derivative is multiplied by a singular perturbation parameter, $\epsilon$, while the nature of the first derivative term is such that flow is aligned with a boundary. These two facts mean that solutions tend to exhibit layers of both exponential and characteristic type. We solve the problem using a finite difference method, specially adapted to the discontinuities, and applied on a piecewise-uniform (Shishkin). We prove that that the computed solution converges to the true one at a rate that is independent of the perturbation parameter, and is nearly first-order. We present numerical results that verify that these results are sharp.
翻译:暂无翻译