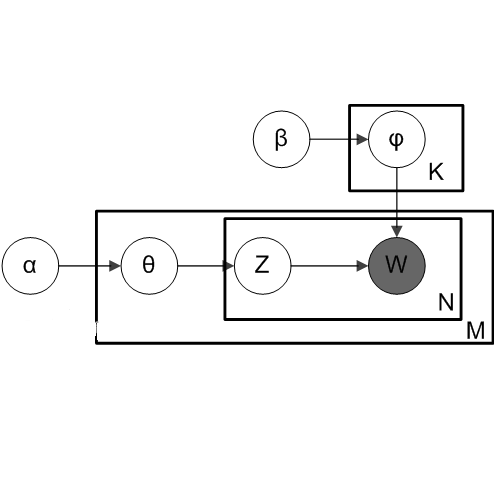
Non-negative matrix factorization with the generalized Kullback-Leibler divergence (NMF) and latent Dirichlet allocation (LDA) are two popular approaches for dimensionality reduction of non-negative data. Here, we show that NMF with $\ell_1$ normalization constraints on the columns of both matrices of the decomposition and a Dirichlet prior on the columns of one matrix is equivalent to LDA. To show this, we demonstrate that explicitly accounting for the scaling ambiguity of NMF by adding $\ell_1$ normalization constraints to the optimization problem allows a joint update of both matrices in the widely used multiplicative updates (MU) algorithm. When both of the matrices are normalized, the joint MU algorithm leads to probabilistic latent semantic analysis (PLSA), which is LDA without a Dirichlet prior. Our approach of deriving joint updates for NMF also reveals that a Lasso penalty on one matrix together with an $\ell_1$ normalization constraint on the other matrix is insufficient to induce any sparsity.
翻译:暂无翻译