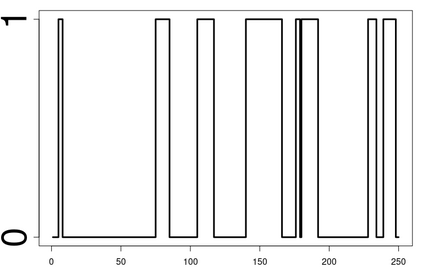
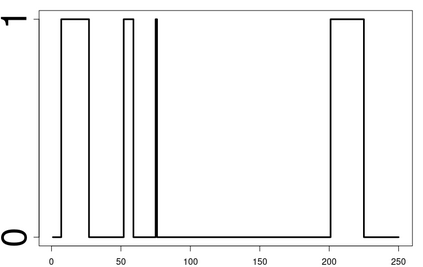
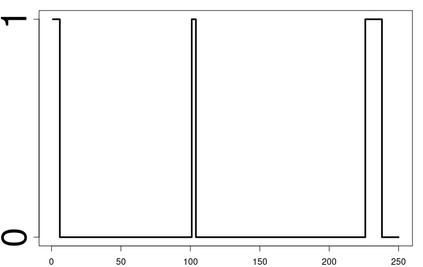
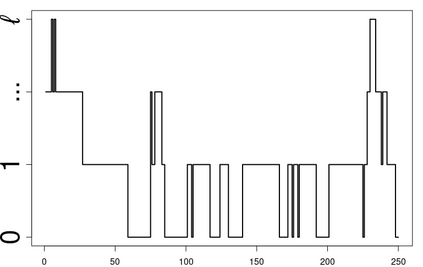
We propose and investigate a hidden Markov model (HMM) for the analysis of dependent, aggregated, superimposed two-state signal recordings. A major motivation for this work is that often these signals cannot be observed individually but only their superposition. Among others, such models are in high demand for the understanding of cross-talk between ion channels, where each single channel cannot be measured separately. As an essential building block, we introduce a parameterized vector norm dependent Markov chain model and characterize it in terms of permutation invariance as well as conditional independence. This building block leads to a hidden Markov chain sum process which can be used for analyzing the dependence structure of superimposed two-state signal observations within an HMM. Notably, the model parameters of the vector norm dependent Markov chain are uniquely determined by the parameters of the sum process and are therefore identifiable. We provide algorithms to estimate the parameters, discuss model selection and apply our methodology to real-world ion channel data from the heart muscle, where we show competitive gating.
翻译:暂无翻译