Random matrices and safety rest areas on interstates in the United States

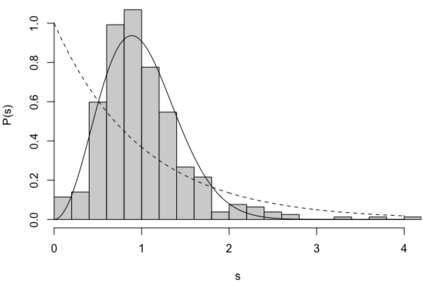
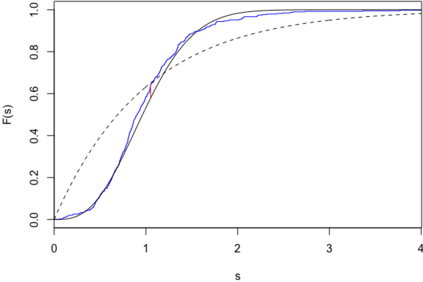
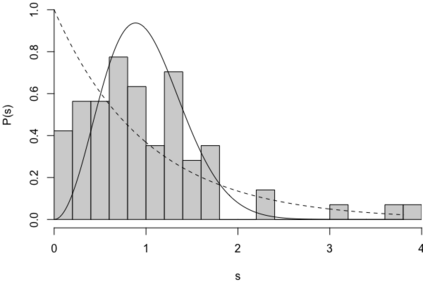
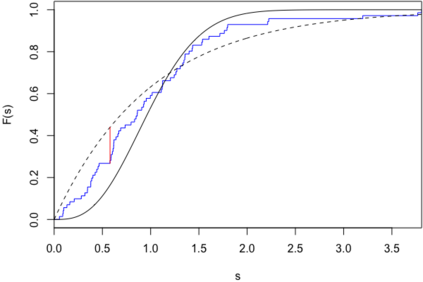
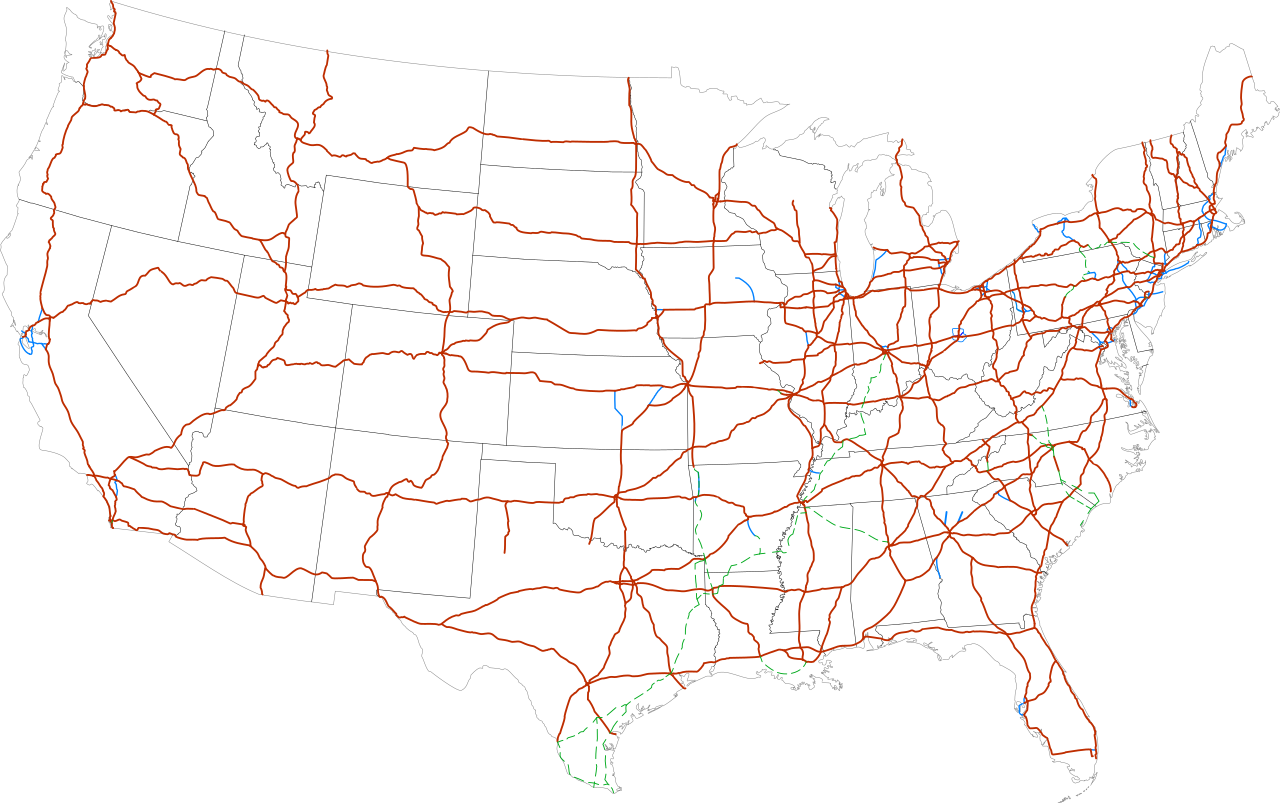
We analyze physical spacings between locations of safety rest areas on interstates in the United States. We show normalized safety rest area spacings on major interstates exhibit Wigner surmise statistics, which align with the eigenvalue spacings for the Gaussian Unitary Ensemble from random matrix theory as well as the one-dimensional gas interactions via the Coulomb potential. We identify economic and geographic regional traits at the state level that exhibit Poissonian statistics, which become more pronounced with increased geographical obstacles in interstate travel. Moreover, the long time scale of planning and construction of safety rest area sites appears to mitigate any profile distinctions due to potential political influences.