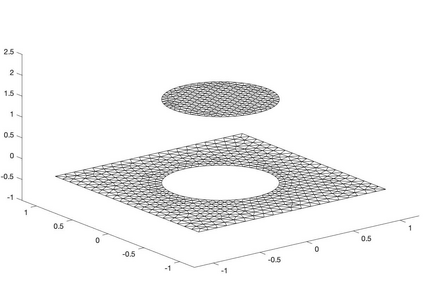
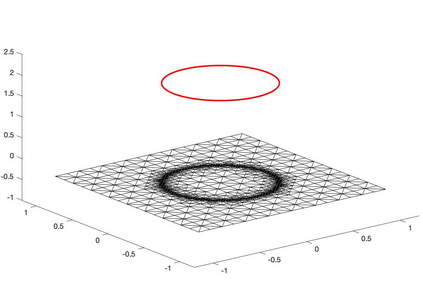
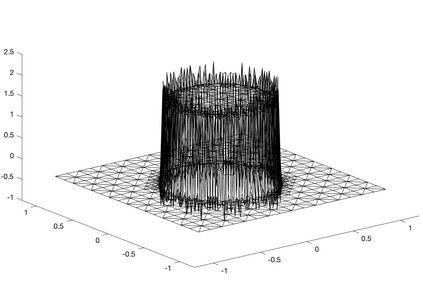
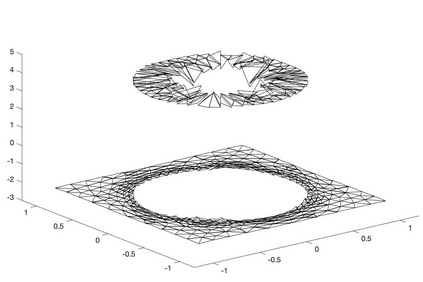
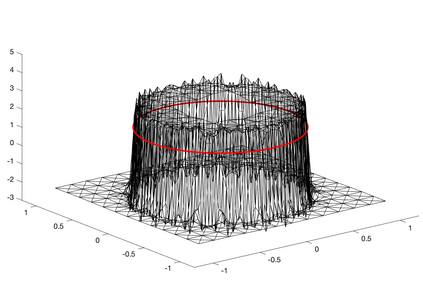
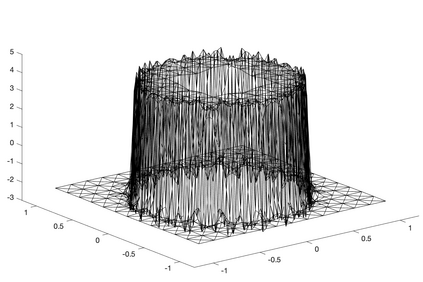
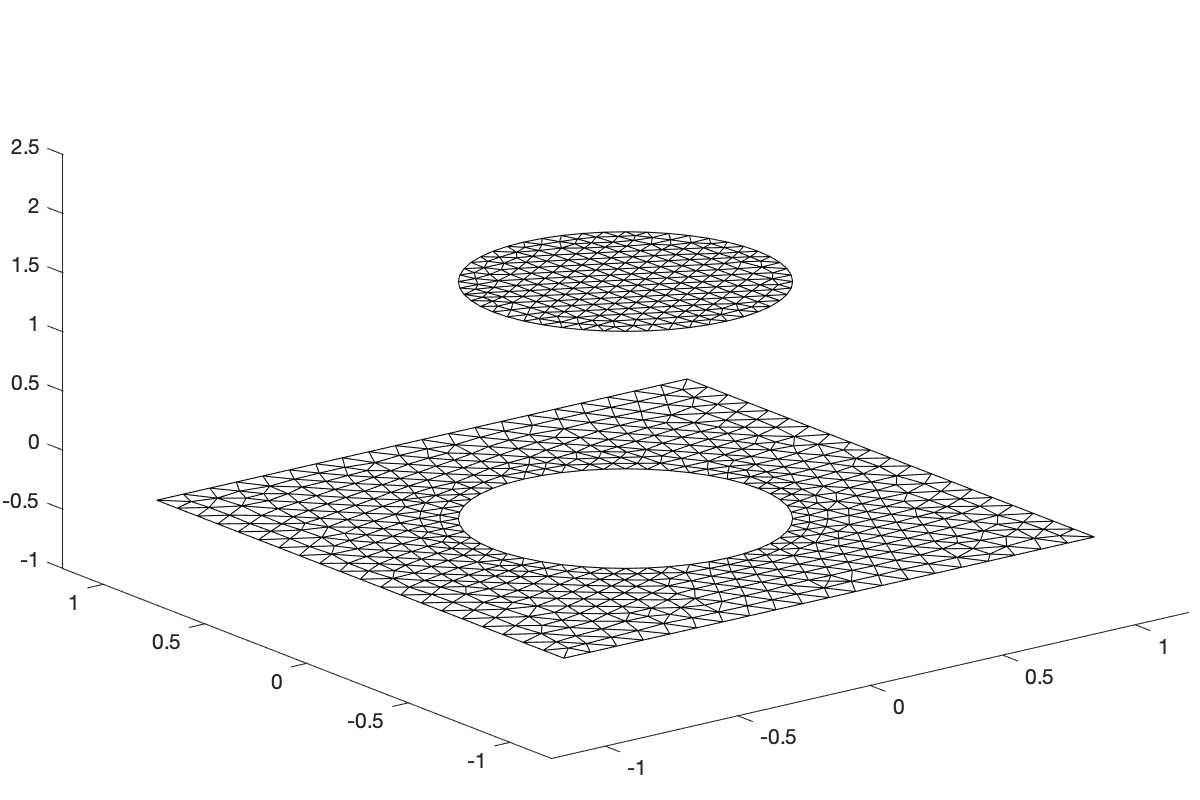
We consider the numerical approximation of a sharp-interface model for two-phase flow, which is given by the incompressible Navier-Stokes equations in the bulk domain together with the classical interface conditions on the interface. We propose structure-preserving finite element methods for the model, meaning in particular that volume preservation and energy decay are satisfied on the discrete level. For the evolving fluid interface, we employ parametric finite element approximations that introduce an implicit tangential velocity to improve the quality of the interface mesh. For the two-phase Navier-Stokes equations, we consider two different approaches: an unfitted and a fitted finite element method, respectively. In the unfitted approach, the constructed method is based on an Eulerian weak formulation, while in the fitted approach a novel arbitrary Lagrangian-Eulerian (ALE) weak formulation is introduced. Using suitable discretizations of these two formulations, we introduce two finite element methods and prove their structure-preserving properties. Numerical results are presented to show the accuracy and efficiency of the introduced methods.
翻译:暂无翻译