Do forecasts of bankruptcy cause bankruptcy? A machine learning sensitivity analysis

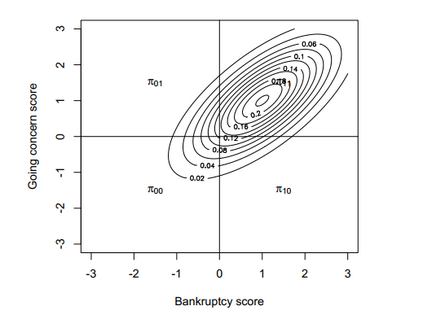
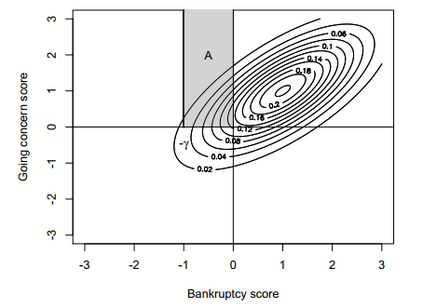
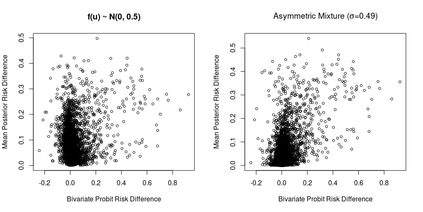
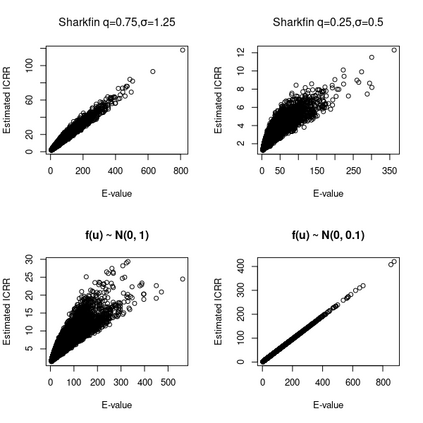
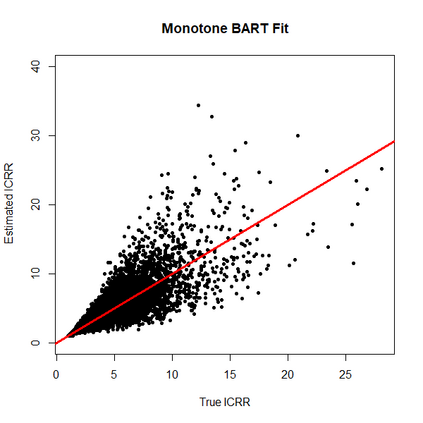
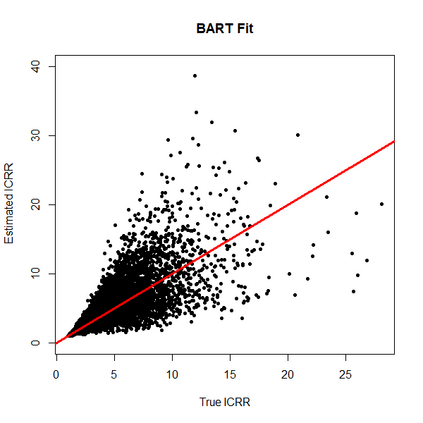
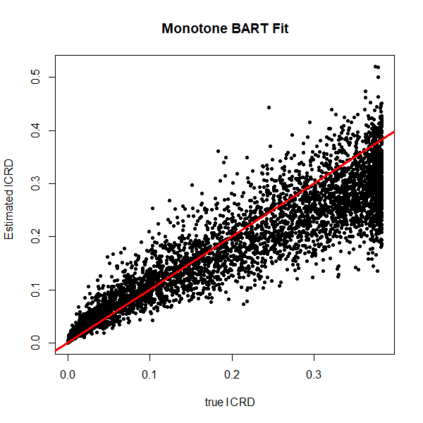
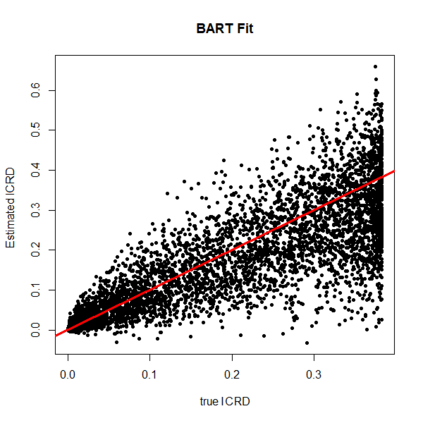
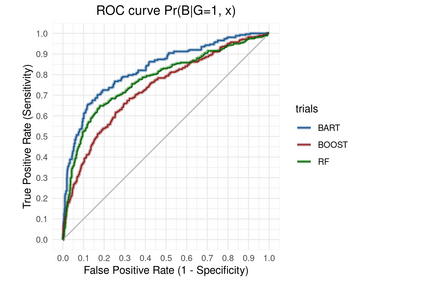
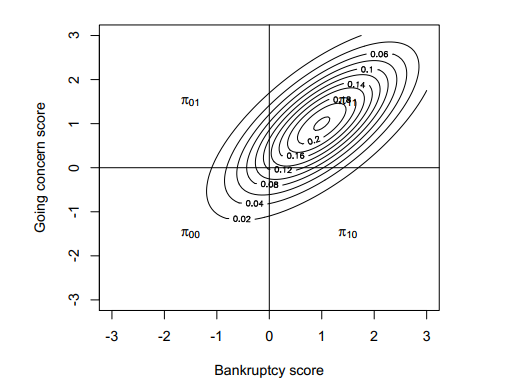
It is widely speculated that auditors' public forecasts of bankruptcy are, at least in part, self-fulfilling prophecies in the sense that they might actually cause bankruptcies that would not have otherwise occurred. This conjecture is hard to prove, however, because the strong association between bankruptcies and bankruptcy forecasts could simply indicate that auditors are skillful forecasters with unique access to highly predictive covariates. In this paper, we investigate the causal effect of bankruptcy forecasts on bankruptcy using nonparametric sensitivity analysis. We contrast our analysis with two alternative approaches: a linear bivariate probit model with an endogenous regressor, and a recently developed bound on risk ratios called E-values. Additionally, our machine learning approach incorporates a monotonicity constraint corresponding to the assumption that bankruptcy forecasts do not make bankruptcies less likely. Finally, a tree-based posterior summary of the treatment effect estimates allows us to explore which observable firm characteristics moderate the inducement effect.