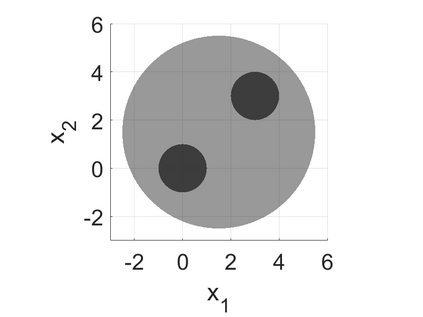
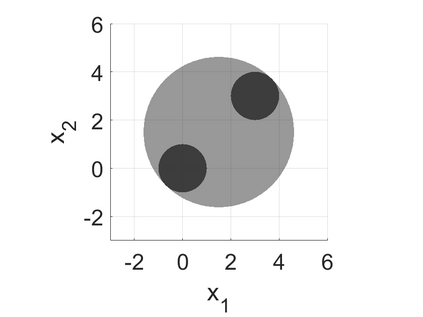
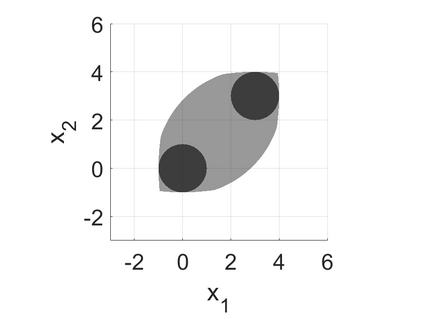
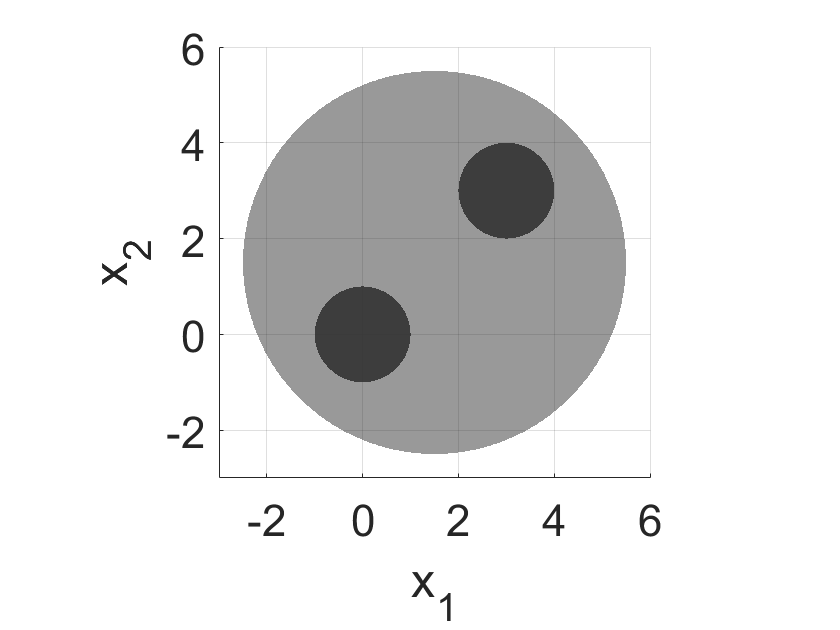
This work develops a class of relaxations in between the big-M and convex hull formulations of disjunctions, drawing advantages from both. The proposed "P-split" formulations split convex additively separable constraints into P partitions and form the convex hull of the partitioned disjuncts. Parameter P represents the trade-off of model size vs. relaxation strength. We examine the novel formulations and prove that, under certain assumptions, the relaxations form a hierarchy starting from a big-M equivalent and converging to the convex hull. We computationally compare the proposed formulations to big-M and convex hull formulations on a test set including: K-means clustering, P_ball problems, and ReLU neural networks. The computational results show that the intermediate P-split formulations can form strong outer approximations of the convex hull with fewer variables and constraints than the extended convex hull formulations, giving significant computational advantages over both the big-M and convex hull.
翻译:这项工作开发了大M型和锥形船体配制之间的松动, 并从两者中引出优势。 拟议的“ P- Split” 配方将大M型和锥形船体配制分开, 将分解成P分区, 形成被分割的分离体的锥体壳。 参数P 代表模型大小与放松强度的权衡。 我们检查了新配方, 并证明在某些假设下, 放松形成了一个等级, 从大M等量开始, 并融合到锥体船体。 我们计算了将拟议配方与大M型和锥体体配制相比较, 测试组包括: K- 平均值组合、 P_ ball 问题和 ReLU 神经网络。 计算结果显示, 中间P- Split 配方可以形成锥体体体体体体体体的强烈外近似, 其变数和制约比扩展的卷式船体配方体都小, 给大M 和锥体壳都带来巨大的计算优势 。