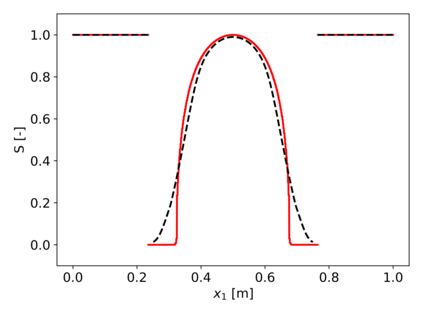
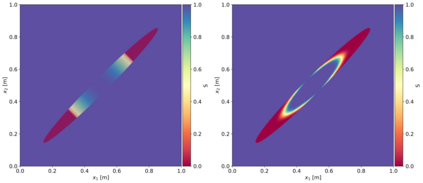
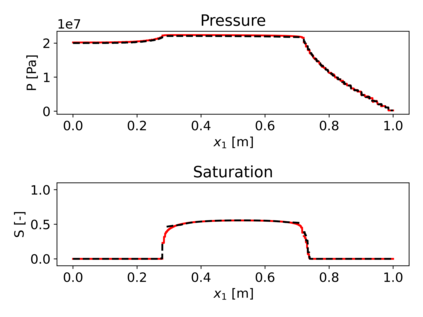
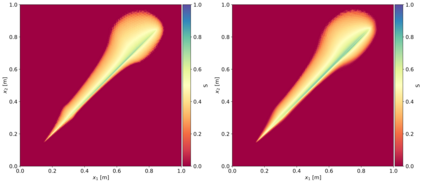
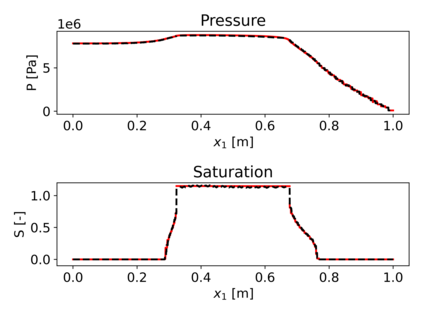
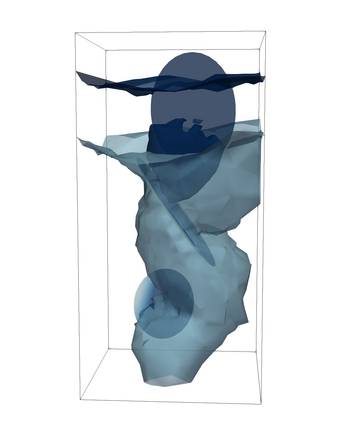
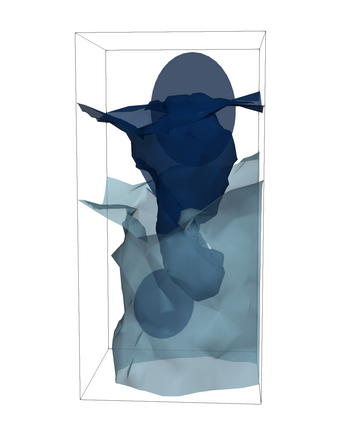
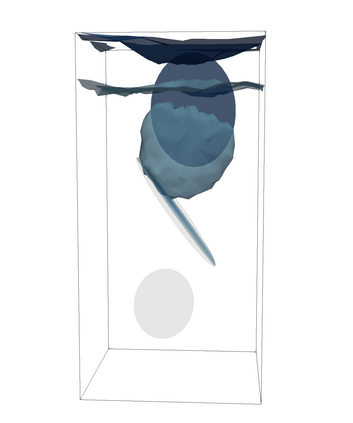
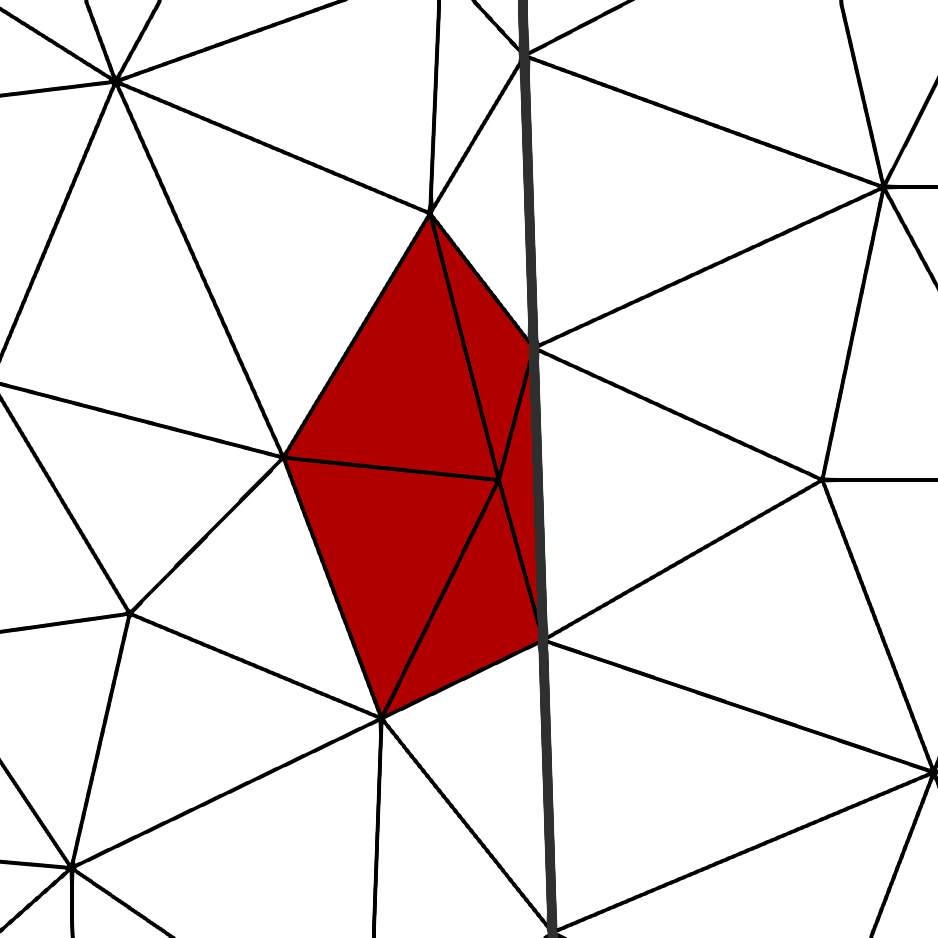
Flow in fractured porous media is modeled frequently by discrete fracture-matrix approaches where fractures are treated as dimensionally reduced manifolds. Generalizing earlier work we focus on two-phase flow in time-dependent fracture geometries including the fracture's aperture. We present the derivation of a reduced model for immiscible two-phase flow in porous media. For the reduced model we present a fully conforming finite-volume discretization coupled with a moving-mesh method. This method permits arbitrary movement of facets of the triangulation while being fully conservative. In numerical examples we show the performance of the scheme and investigate the modeling error of the reduced model.
翻译:在断裂多孔介质中,断裂流往往以离散断裂矩阵法作为模型,骨折被视为尺寸缩小的元件。我们以前的工作重点是按时间划分的两阶段断裂形形体流,包括断裂孔径。我们介绍了在多孔介质中不强迫的两阶段流动的减少模型的衍生结果。在减少的介质中,我们展示了完全符合一定量的离散和移动式移动方法。这种方法允许在完全保守的情况下任意移动三角线的方位。在数字示例中,我们展示了该计划的性能,并调查了减少的模型的建模错误。