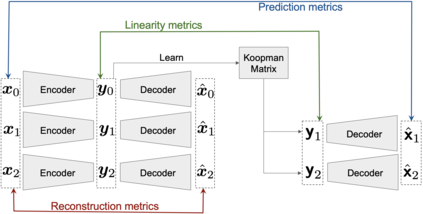
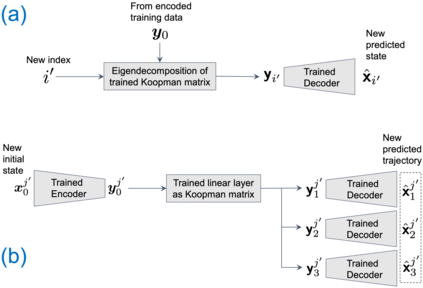
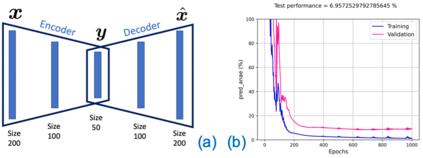
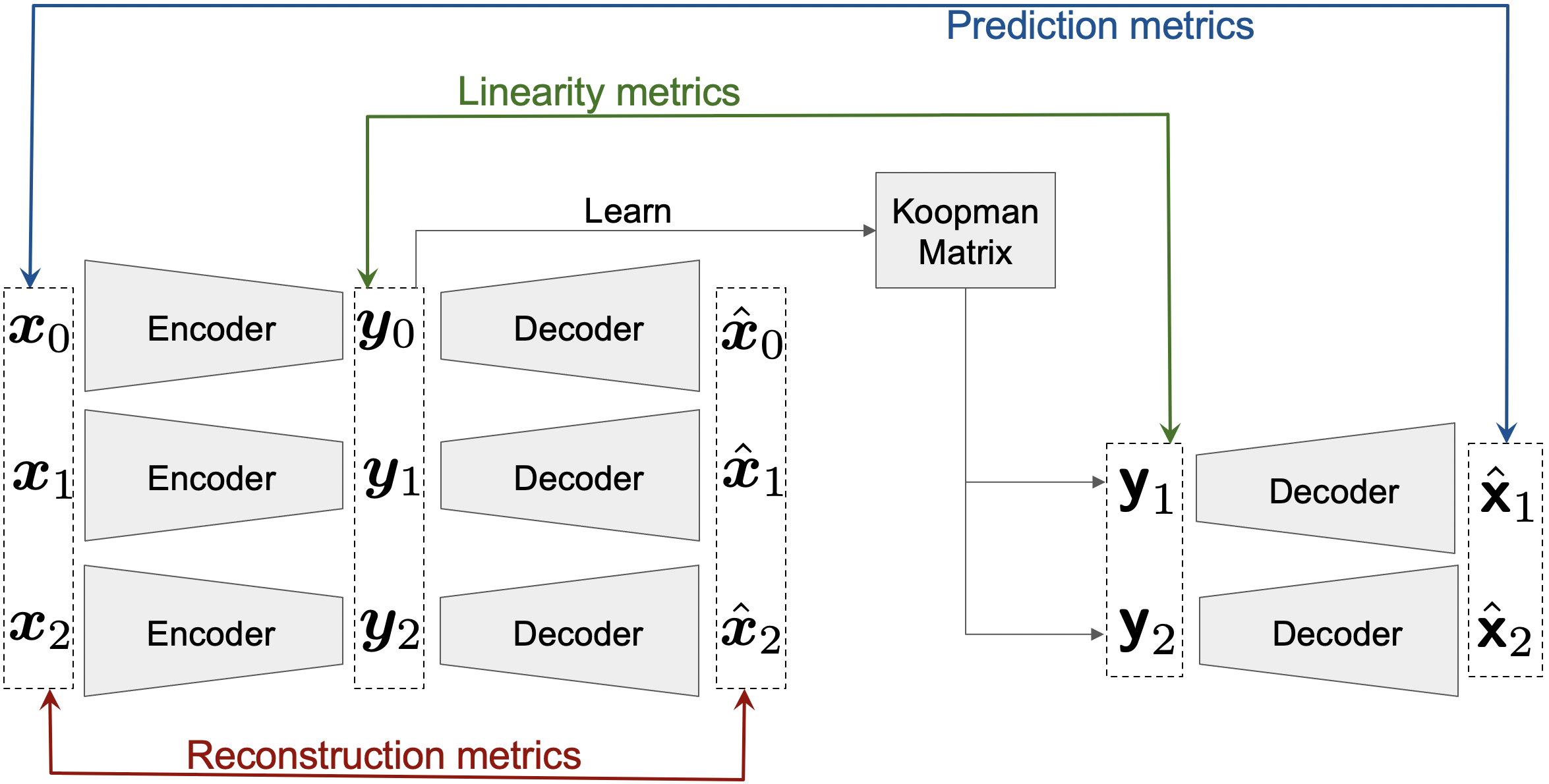
We present DLKoopman -- a software package for Koopman theory that uses deep learning to learn an encoding of a nonlinear dynamical system into a linear space, while simultaneously learning the linear dynamics. While several previous efforts have either restricted the ability to learn encodings, or been bespoke efforts designed for specific systems, DLKoopman is a generalized tool that can be applied to data-driven learning and optimization of any dynamical system. It can either be trained on data from individual states (snapshots) of a system and used to predict its unknown states, or trained on data from trajectories of a system and used to predict unknown trajectories for new initial states. DLKoopman is available on the Python Package Index (PyPI) as 'dlkoopman', and includes extensive documentation and tutorials. Additional contributions of the package include a novel metric called Average Normalized Absolute Error for evaluating performance, and a ready-to-use hyperparameter search module for improving performance.
翻译:暂无翻译