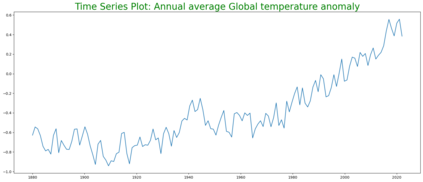
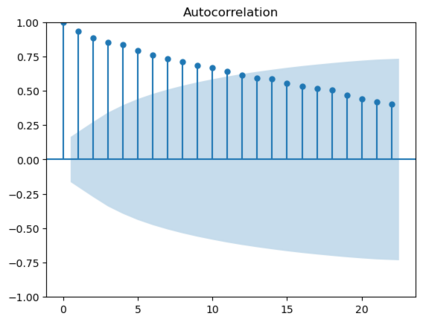
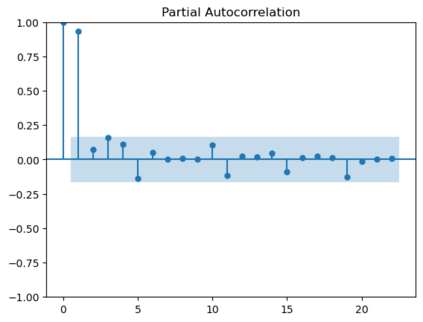
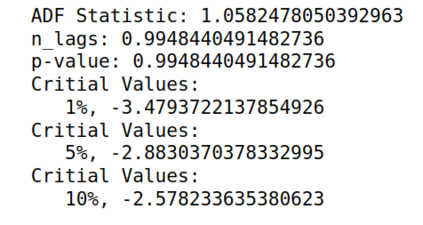
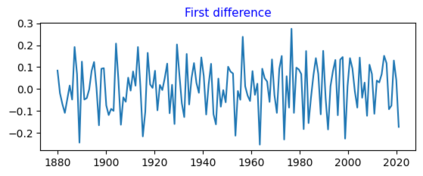
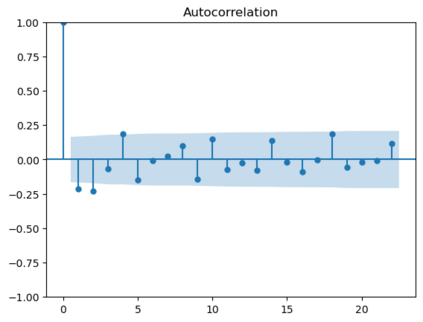
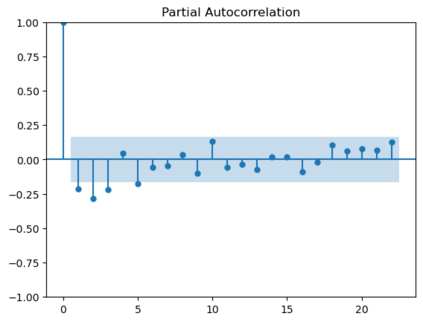
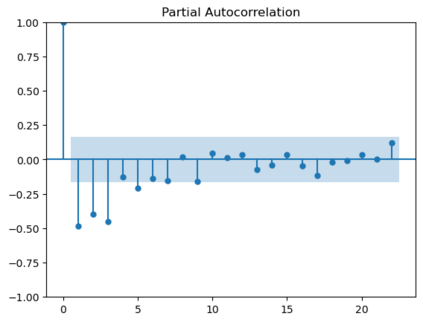
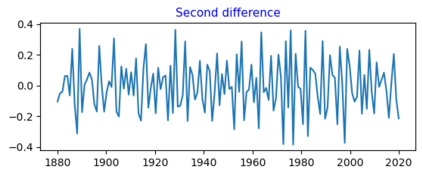
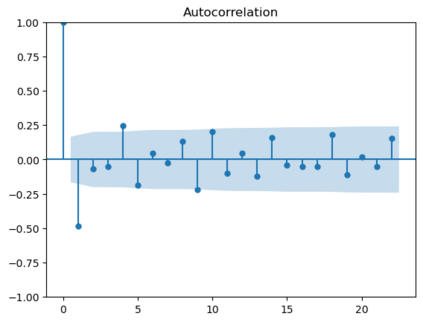
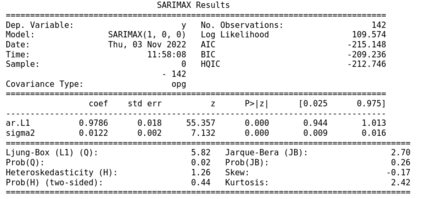
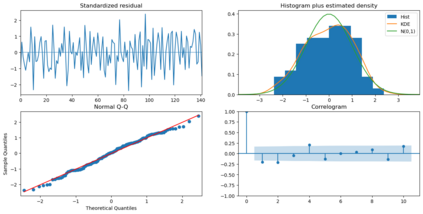
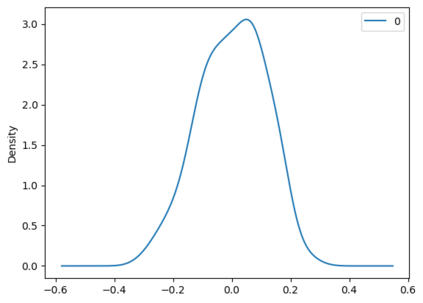
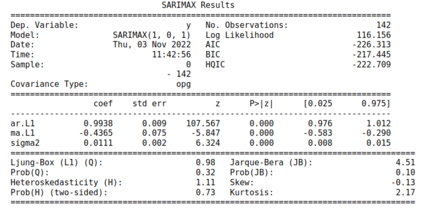
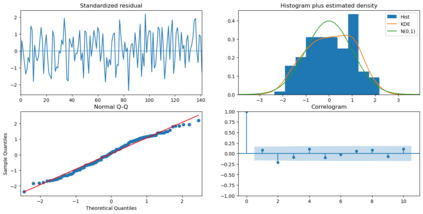
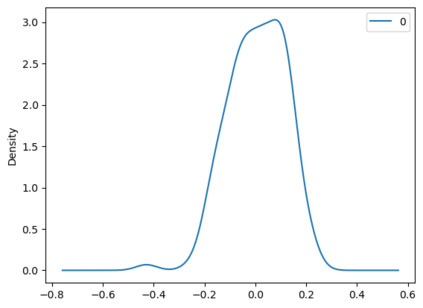
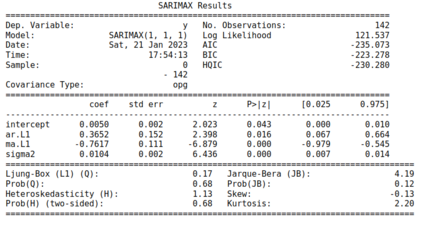
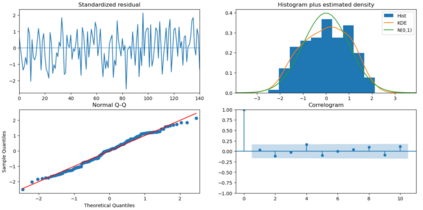
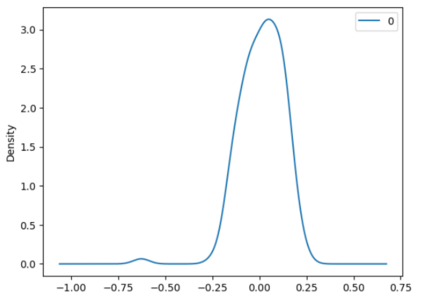
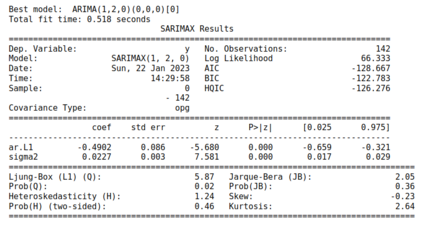
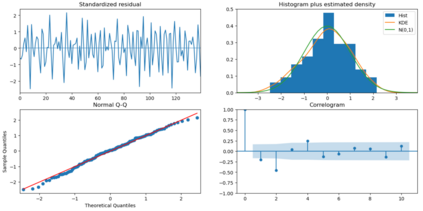
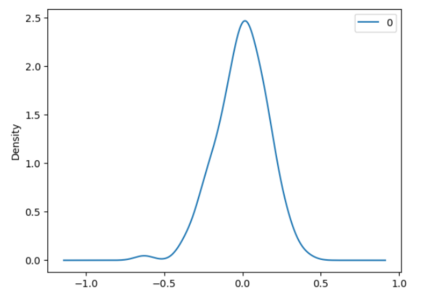
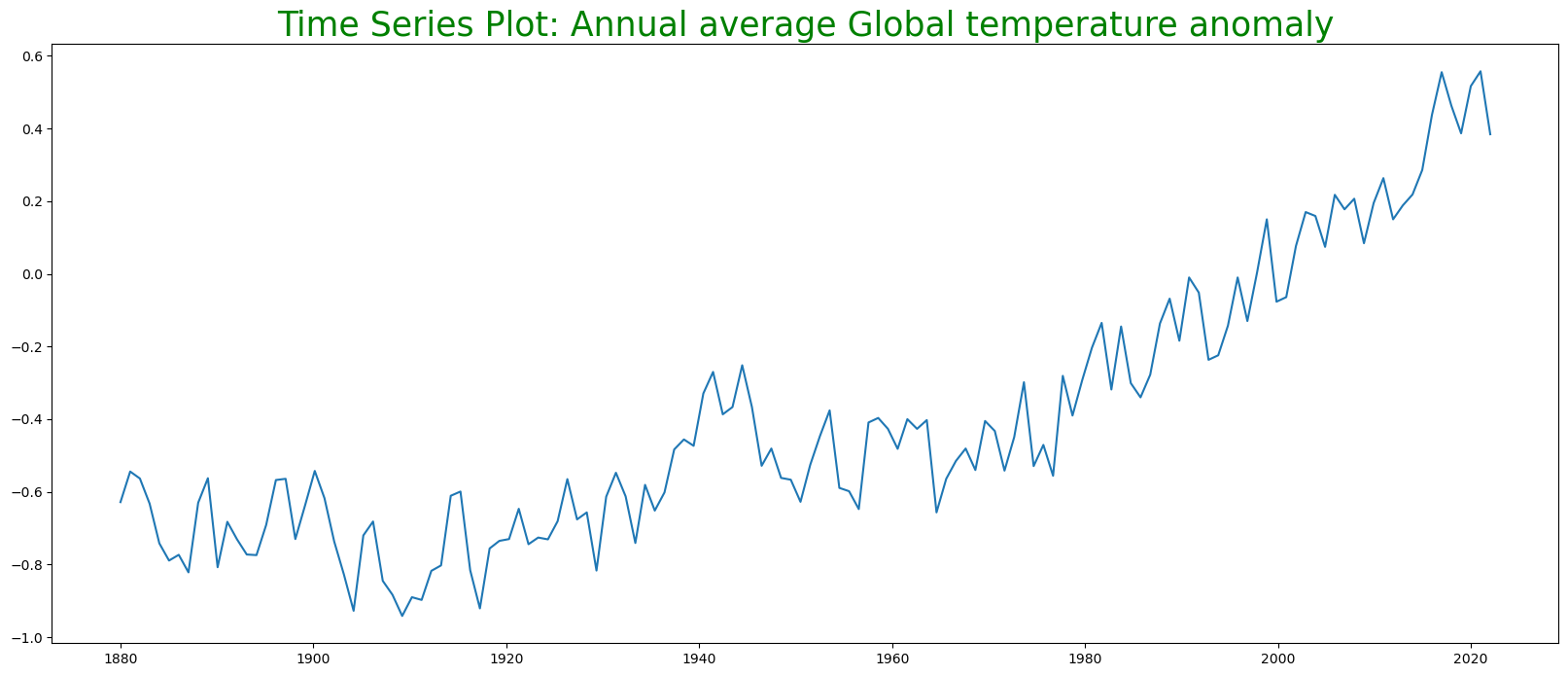
Temperature uncertainty models for land and sea surfaces can be developed based on statistical methods. In this paper, we developed a novel time series temperature uncertainty model which is the Auto-regressive Moving Average (ARMA)(1, 1) model. The model was developed for observed annual mean temperature anomaly X(t) which is a combination of true (latent) global anomaly Y (t) for a year (t) and normal variable w(t). The uncertainty is taken as the variance of w(t) which was decomposed to Land Surface Temperature (LST) uncertainty, Sea Surface Temperature (SST) uncertainty, and the corresponding source of uncertainty. The ARMA model was analyzed and compared with Auto-regressive (AR), and Auto-regressive integrated moving average (ARIMA) for the data taken from NASA, Goddard Institute for space studies Surface Temperature Analysis. The statistical analysis of the Auto-correlation function (ACF), Partial auto-correlation function (PACF), Normal quantile-quantile (Normal Q-Q) plot, the density of the residuals, and variance of normal variable w(t) show that ARMA(1, 1)is better than AR(1) and ARIMA(1, d, 1) for d = 1, 2.
翻译:在本文件中,我们开发了一个新的时间序列温度不确定性模型,即自动递减平均(ARMA) 1,1模型。该模型是为观测的年平均温度异常X(t)而开发的,该模型是一年(t)和正常变数(t)真实的(远)全球异常Y(t)和正常的变数(t)结合的。不确定性被理解为与地表温度(LST)的不确定性、海面温度不确定性(SST)和相应的不确定性源(SST)之间的差异。对ARMA模型进行了分析和比较,并与自动递减平均(AR)和自动递增综合移动平均(ARIMA)结合了美国航天局、哥达德空间研究地面温度分析研究所(Godard Institutom)和普通变数(wt)的数据。对自动调节功能(ACF)、部分自动调节功能(PACF)、正常定量(PACF)、海面温度(NAR-QQ-Q)的不确定性和相应的不确定性源(NARQ-Q)绘图、A- Rema1的密度和正常变差(AR1)、A1、AR1、A1、AR1、AR1和A1的变差(A1)的密度和变数进行了统计分析。</s>