A new Lagrange multiplier approach for constructing positivity preserving schemes

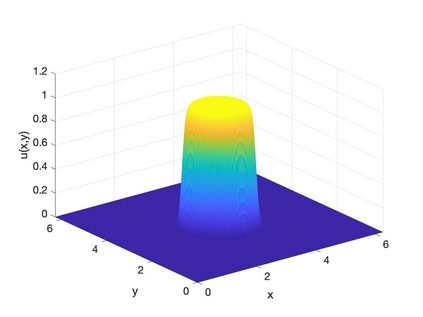
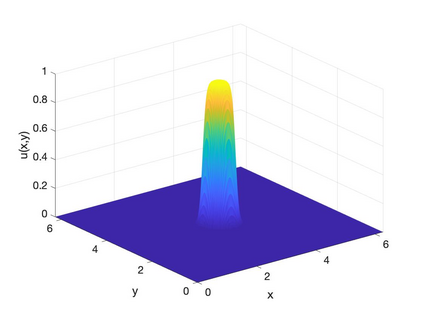
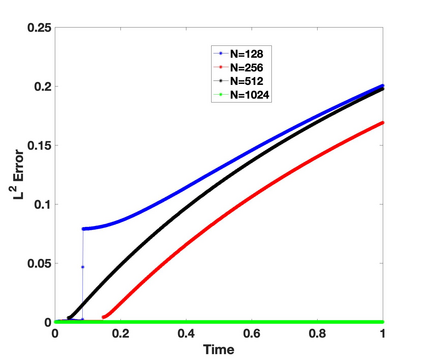
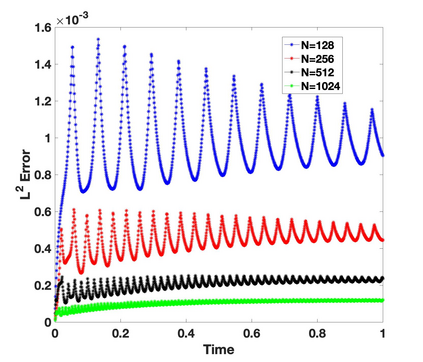
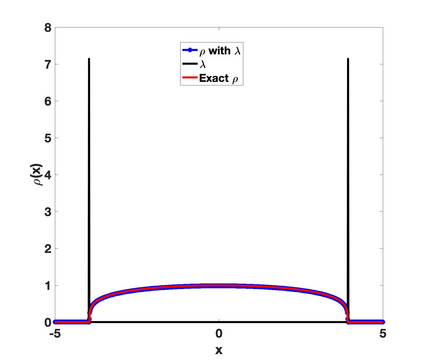
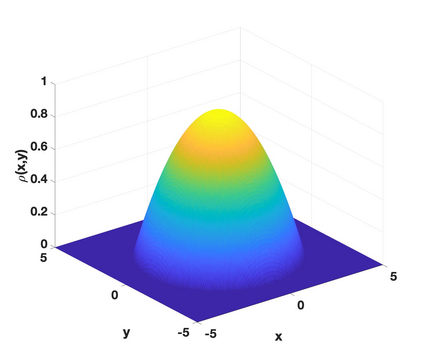
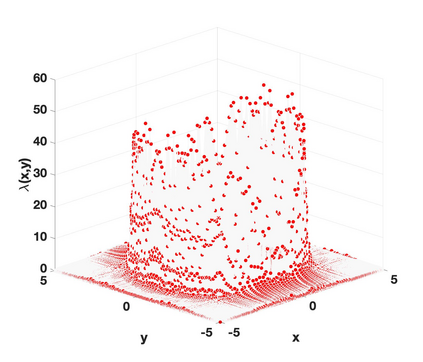
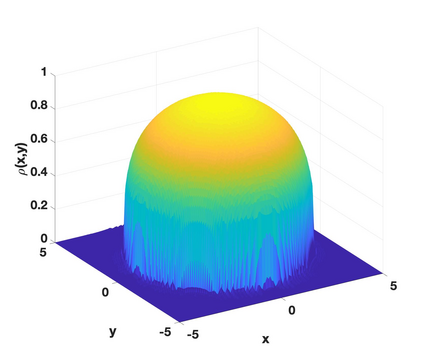
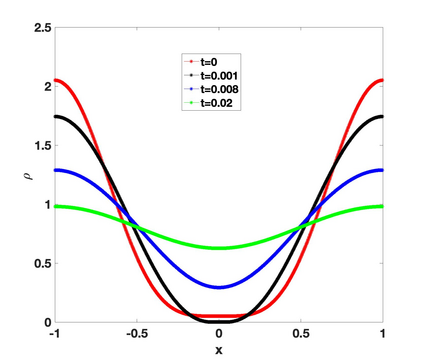
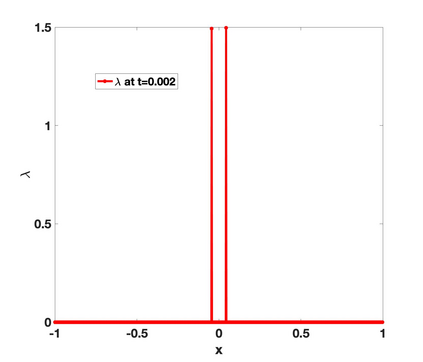
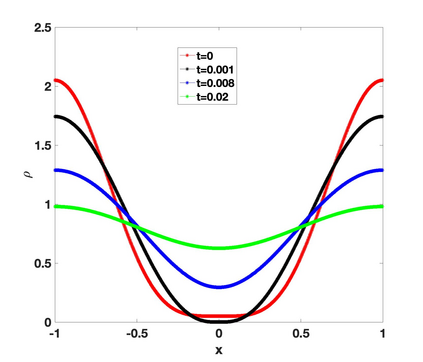
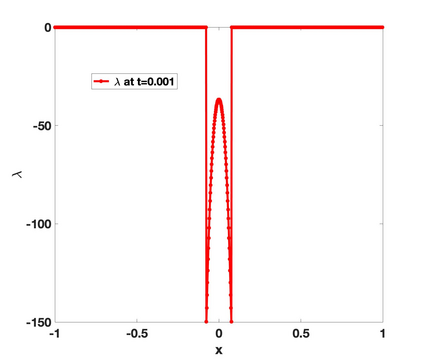
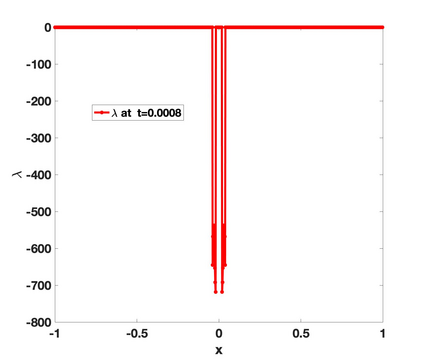
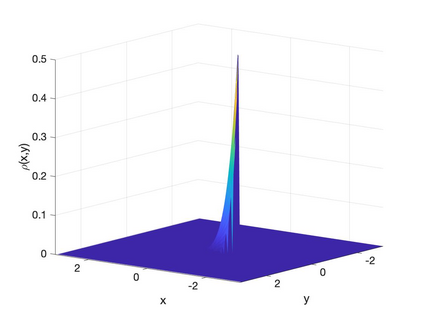
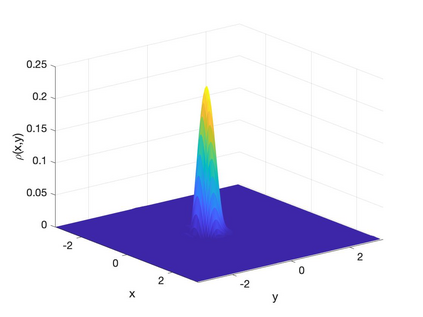
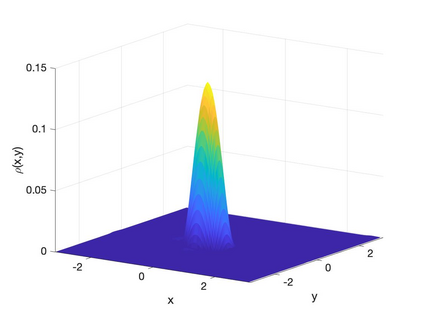
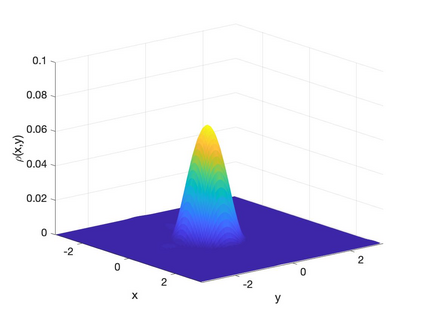
We propose a new Lagrange multiplier approach to construct positivity preserving schemes for parabolic type equations. The new approach is based on expanding a generic spatial discretization, which is not necessarily positivity preserving, by introducing a space-time Lagrange multiplier coupled with Karush-Kuhn-Tucker (KKT) conditions to preserve positivity. The key for an efficient and accurate time discretization of the expanded system is to adopt an operator-splitting or predictor-corrector approach in such a way that (i) the correction step can be implemented with negligible cost, and (ii) it preserves the order of schemes at the prediction step. We establish some stability results under a general setting, and carry out error estimates for first-order versions of our approach and linear parabolic equation. We also present ample numerical results to validate the new approach.