The Randomized $k$-Server Conjecture is False!

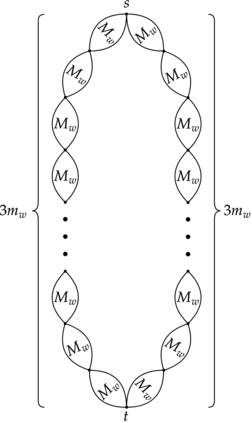
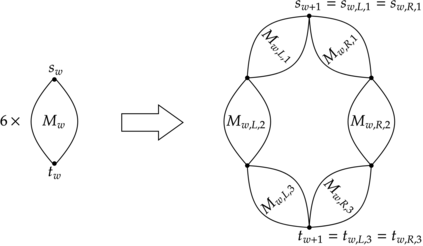
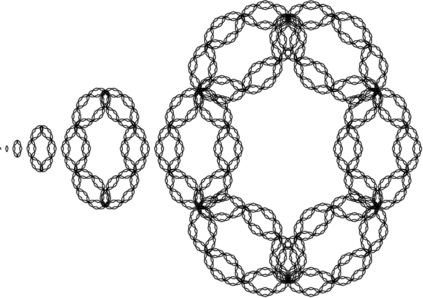
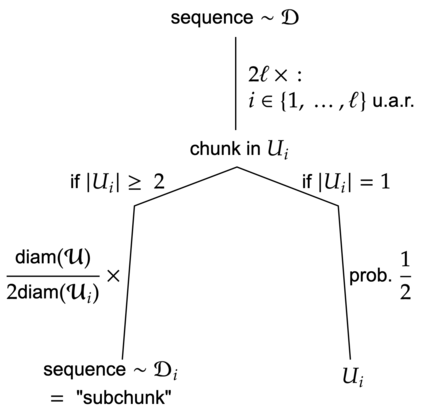
We prove a few new lower bounds on the randomized competitive ratio for the $k$-server problem and other related problems, resolving some long-standing conjectures. In particular, for metrical task systems (MTS) we asympotically settle the competitive ratio and obtain the first improvement to an existential lower bound since the introduction of the model 35 years ago (in 1987). More concretely, we show: 1. There exist $(k+1)$-point metric spaces in which the randomized competitive ratio for the $k$-server problem is $\Omega(\log^2 k)$. This refutes the folklore conjecture (which is known to hold in some families of metrics) that in all metric spaces with at least $k+1$ points, the competitive ratio is $\Theta(\log k)$. 2. Consequently, there exist $n$-point metric spaces in which the randomized competitive ratio for MTS is $\Omega(\log^2 n)$. This matches the upper bound that holds for all metrics. The previously best existential lower bound was $\Omega(\log n)$ (which was known to be tight for some families of metrics). 3. For all $k<n\in\mathbb N$, for *all* $n$-point metric spaces the randomized $k$-server competitive ratio is at least $\Omega(\log k)$, and consequently the randomized MTS competitive ratio is at least $\Omega(\log n)$. These universal lower bounds are asymptotically tight. The previous bounds were $\Omega(\log k/\log\log k)$ and $\Omega(\log n/\log \log n)$, respectively. 4. The randomized competitive ratio for the $w$-set metrical service systems problem, and its equivalent width-$w$ layered graph traversal problem, is $\Omega(w^2)$. This slightly improves the previous lower bound and matches the recently discovered upper bound. 5. Our results imply improved lower bounds for other problems like $k$-taxi, distributed paging and metric allocation. These lower bounds share a common thread, and other than the third bound, also a common construction.