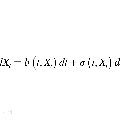We propose a method for sampling from an arbitrary distribution $\exp[-S(\x)]$ with an available gradient $\nabla S(\x)$, formulated as an energy-preserving stochastic differential equation (SDE). We derive the Fokker-Planck equation and show that both the deterministic drift and the stochastic diffusion separately preserve the stationary distribution. This implies that the drift-diffusion discretization schemes are bias-free, in contrast to the standard Langevin dynamics. We apply the method to the $\phi^4$ lattice field theory, showing the results agree with the standard sampling methods but with significantly higher efficiency compared to the current state-of-the-art samplers.
翻译:我们提出了一种从具有可用梯度$\nabla S(\x)$的任意分布$\exp[-S(\x)]$中采样的方法,该方法被称为能量不变的随机微分方程(SDE)。我们推导了福克普朗克方程,并展示了确定性漂移和随机扩散分别保持平稳状态分布。这意味着漂移-扩散离散化方案是无偏差的,与标准的朗之万特动力学相比。我们将该方法应用于$\phi^4$格子场论,显示结果与标准采样方法相一致,但效率比当前现有的最先进的采样器显著提高。