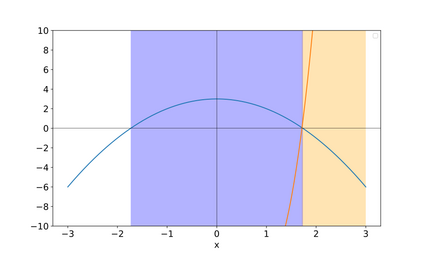
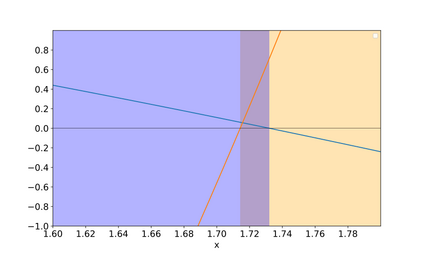
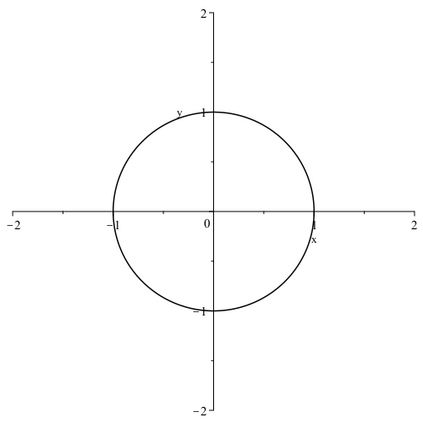
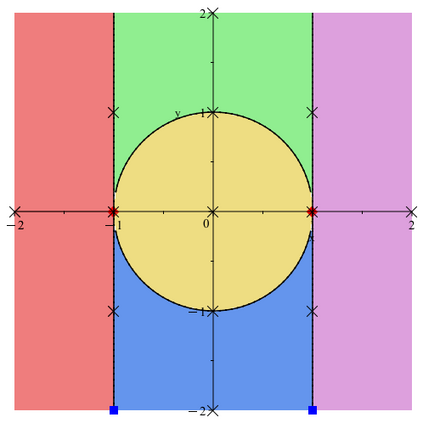
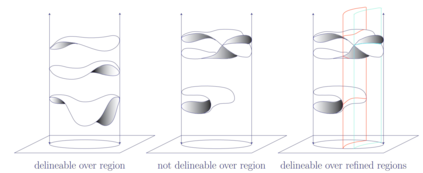
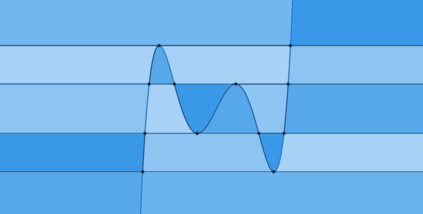
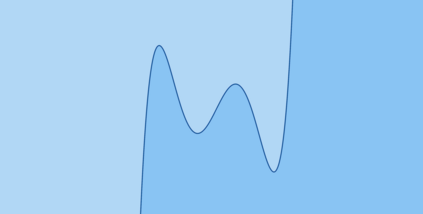
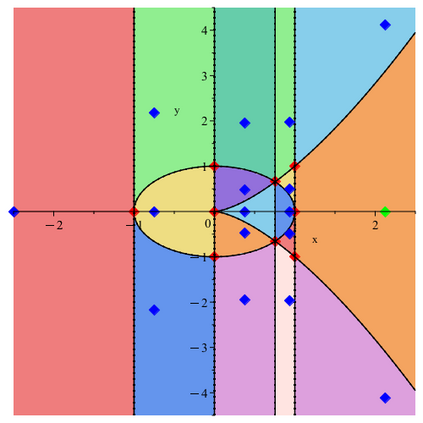
Cylindrical Algebraic Decomposition in Macaulay2

CylindricalAlgebraicDecomposition.m2 is the first implementation of Cylindrical Algebraic Decomposition (CAD) in Macaulay2. CAD decomposes space into 'cells' where input polynomials are sign-invariant. This package computes an Open CAD (full-dimensional cells only) for sets of real polynomials with rational coefficients, enabling users to solve existential problems involving strict inequalities. With the construction of a full CAD (cells of all dimensions), this tool could be extended to solve any real quantifier elimination problem. The current implementation employs the Lazard projection and introduces a new heuristic for choosing the variable ordering.