G-computation for continuous-time data: a comparison with inverse probability weighting

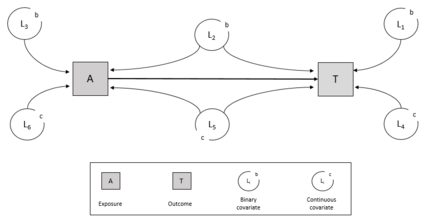
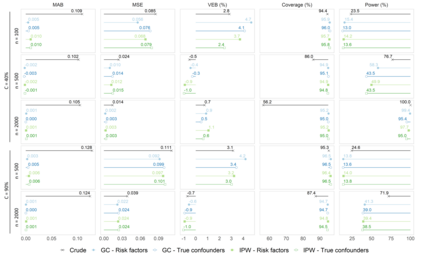
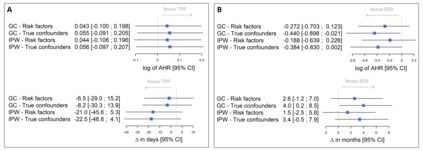
Inverse probability weighting is increasingly used in causal inference, but the g-computation constitutes a promising alternative. We aimed to compare the performances of these methods with time-to-event outcomes. Given the limitations of the interpretability of the hazard ratio for causal inference, our target estimand of interest is the difference in restricted mean survival times. We report the findings of an extensive simulation study showing that both inverse probability weighting and g-computation are unbiased under a correct model specification, but g-computation is generally more efficient. We also analyse two real-world datasets to illustrate these results. Finally, we update the R package RISCA to facilitate the implementation of g-computation.