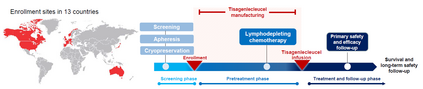
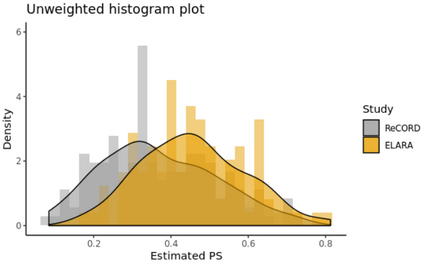
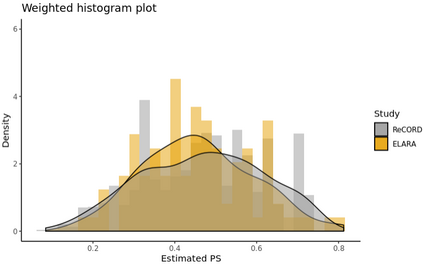
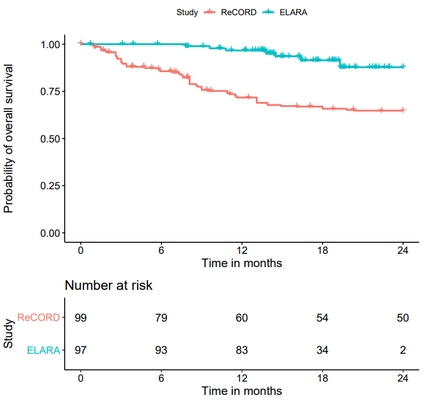
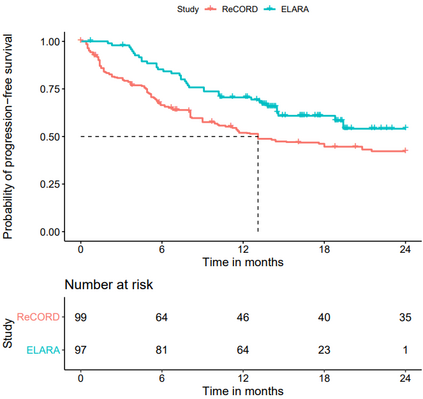
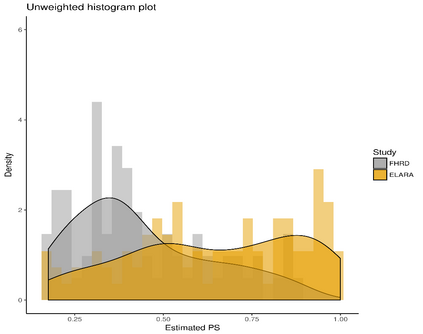
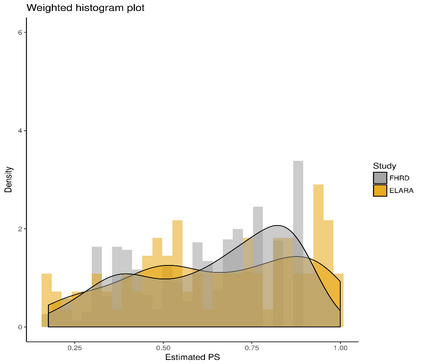
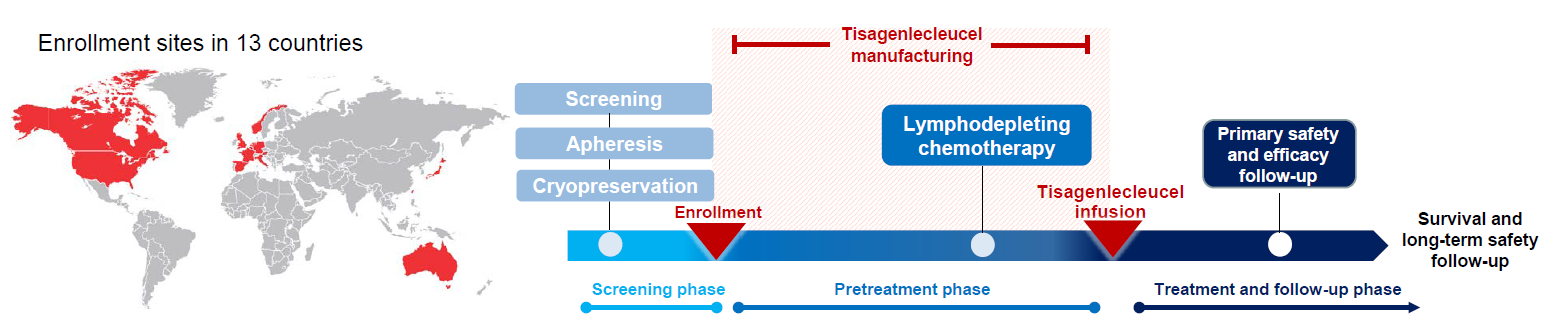
Single-arm trials (SATs) may be used to support regulatory submissions in settings where there is a high unmet medical need and highly promising early efficacy data undermine the equipoise needed for randomization. In this context, patient-level real-world data (RWD) may be used to create an external control arm (ECA) to contextualize the SAT results. However, naive comparisons of the SAT with its ECA will yield biased estimates of causal effects if groups are imbalanced with regards to (un)measured prognostic factors. Several methods are available to adjust for measured confounding, but the interpretation of such analyses is challenging unless the causal question of interest is clearly defined, and the estimator is aligned with the estimand. Additional complications arise when patients in the ECA are eligible for the SAT at multiple timepoints. In this paper, we use a case-study of a pivotal SAT of a novel CAR-T therapy for heavily pre-treated patients with follicular lymphoma to illustrate how a combination of the target trial and the ICH E9(R1) estimand frameworks can be used to define the target estimand and avoid common methodological pitfalls related to the design of the ECA and comparisons with the SAT. We also propose an approach to address the challenge of how to define an appropriate time zero for external controls who meet the SAT inclusion/exclusion criteria at several timepoints. Use of the target trial and estimand frameworks facilitates discussions amongst internal and external stakeholders, as well as an early assessment of the adequacy of the available RWD.
翻译:单臂试验(SATs)可用于支持在医疗需求未得到满足和早期功效数据极有希望的早期效力数据破坏随机化所需的原始设备的情况下提交监管文件;在这方面,病人一级真实世界数据(RWD)可用于建立外部控制部门(ECA),以将SAT结果背景化;然而,如果在(非)诊断性预测性因素方面各组之间出现不平衡,对SAT与其非洲经委会进行天真的比较,则会对因果关系作出偏颇的估计;有几种方法可用于调整,以适应所衡量的混杂情况,但这种分析的解释具有挑战性,除非能明确界定因果关系问题,而且估计数据与估计值相一致。当非洲经委会病人在多个时间点有资格获得SAT的外部控制(ECA)时,则会出现更多的复杂情况。 本文中,我们用一个关键的SAT(CAR-T)新疗法的案例研究,以治疗重症候前病人的淋巴瘤因素为主,说明目标试验与ICH E9(R1)估计值框架之间的现有指标性问题是如何结合的,除非估算值与估计值相一致的外部指标和时间框架能够确定AEASD标准的外部比较。