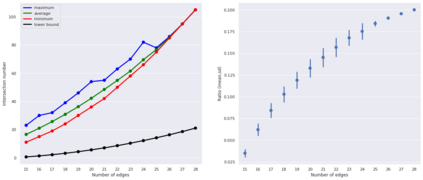
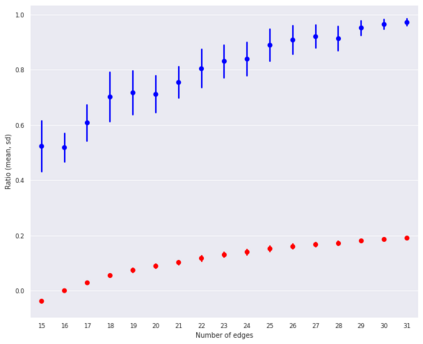
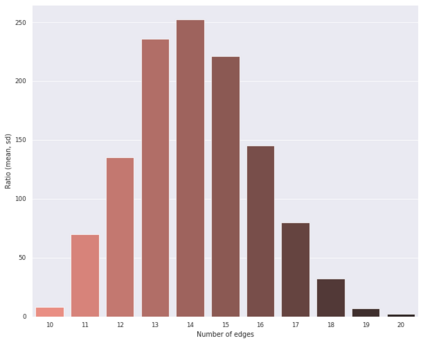
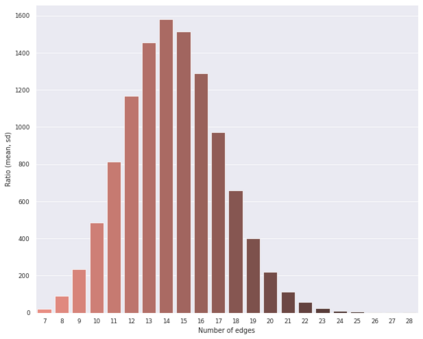
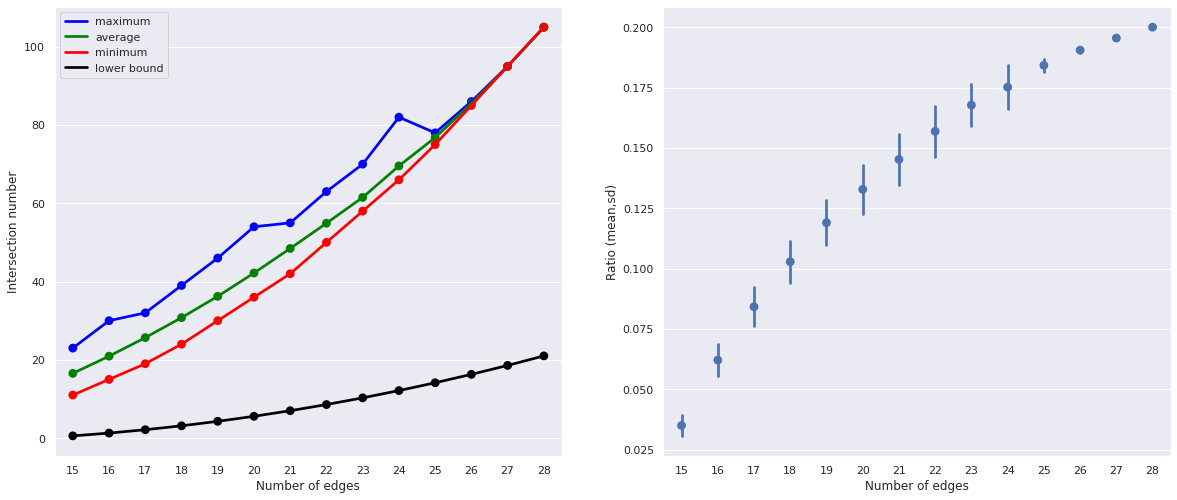
Consider a connected graph $G$ and let $T$ be a spanning tree of $G$. Every edge $e \in G-T$ induces a cycle in $T \cup \{e\}$. The intersection of two distinct such cycles is the set of edges of $T$ that belong to both cycles. The MSTCI problem consists in finding a spanning tree that has the least number of such non-empty intersections and the instersection number is the number of non-empty intersections of a solution. In this article we consider three aspects of the problem in a general context (i.e. for arbitrary connected graphs). The first presents two lower bounds of the intersection number. The second compares the intersection number of graphs that differ in one edge. The last is an attempt to generalize a recent result for graphs with a universal vertex.
翻译：考虑一个连接的图形$G$, 并让$T$成为一棵覆盖以$G$为单位的树。 每一个边缘$e $ $ $ $ $ $ $ $ $ - $ $ $ $ $ $ $ $ $ $$ $ 美元 。 每个边缘 $ $ $ $ $ $ $ $ $ $ $ 美元 的循环以$T\ cup $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $ $
相关内容
微信扫码咨询专知VIP会员