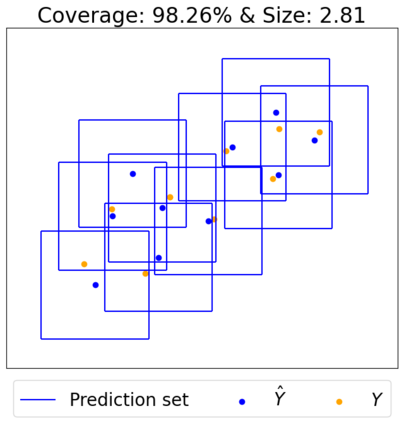
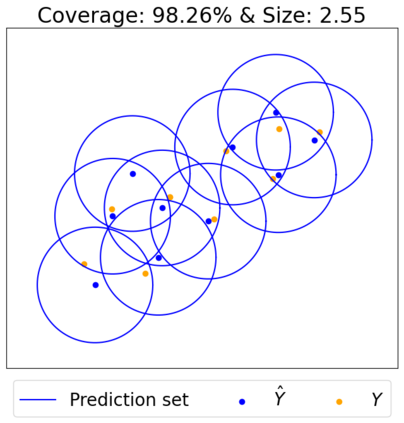
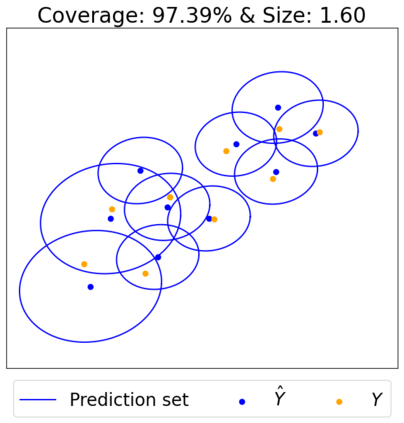
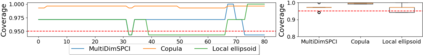
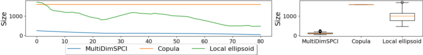
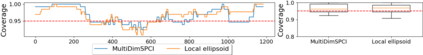
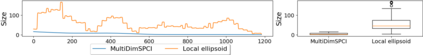
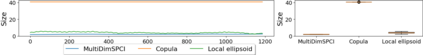
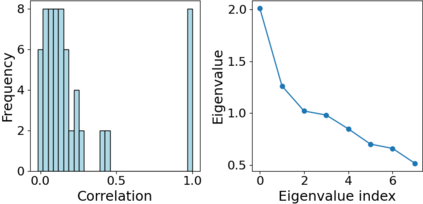
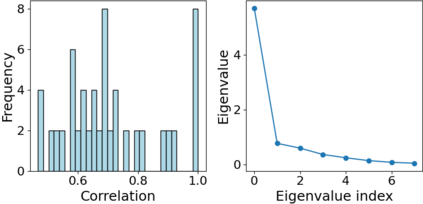
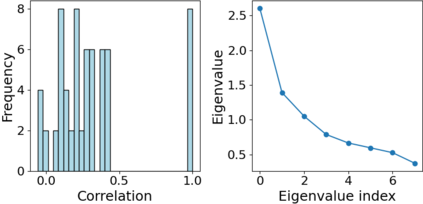
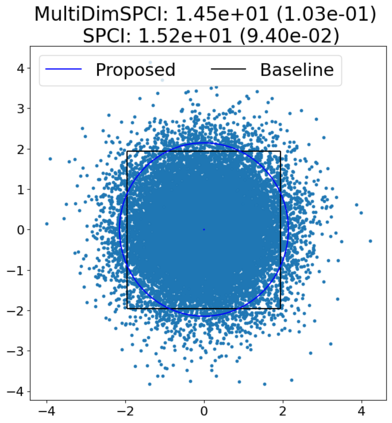
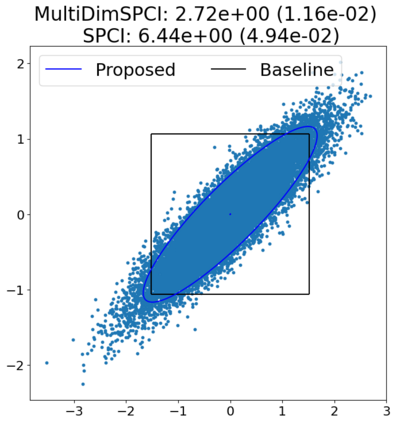
Conformal prediction (CP) has been a popular method for uncertainty quantification because it is distribution-free, model-agnostic, and theoretically sound. For forecasting problems in supervised learning, most CP methods focus on building prediction intervals for univariate responses. In this work, we develop a sequential CP method called $\texttt{MultiDimSPCI}$ that builds prediction regions for a multivariate response, especially in the context of multivariate time series, which are not exchangeable. Theoretically, we estimate finite-sample high-probability bounds on the conditional coverage gap. Empirically, we demonstrate that $\texttt{MultiDimSPCI}$ maintains valid coverage on a wide range of multivariate time series while producing smaller prediction regions than CP and non-CP baselines.
翻译:暂无翻译