The realizability of discs with ribbons on a Möbius strip

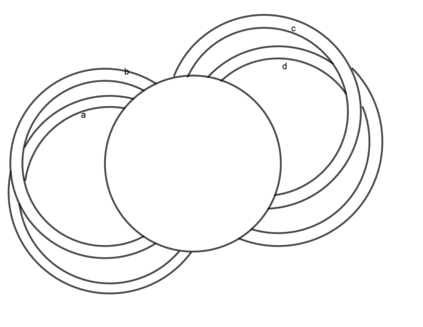
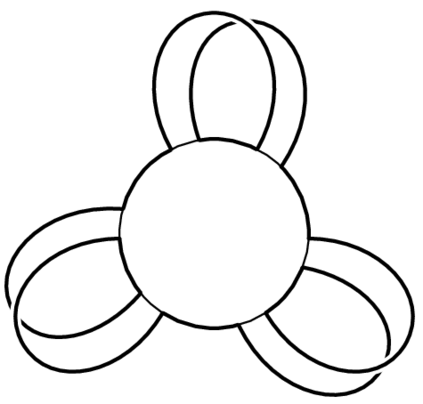
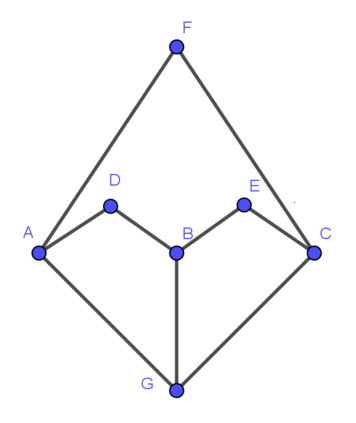
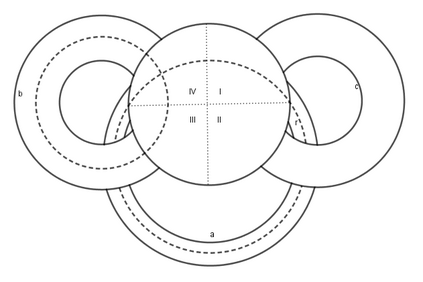
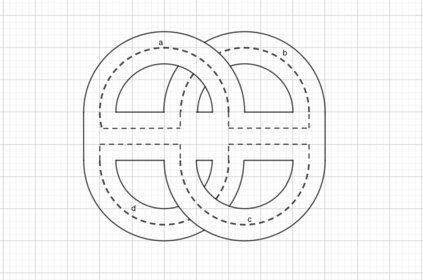
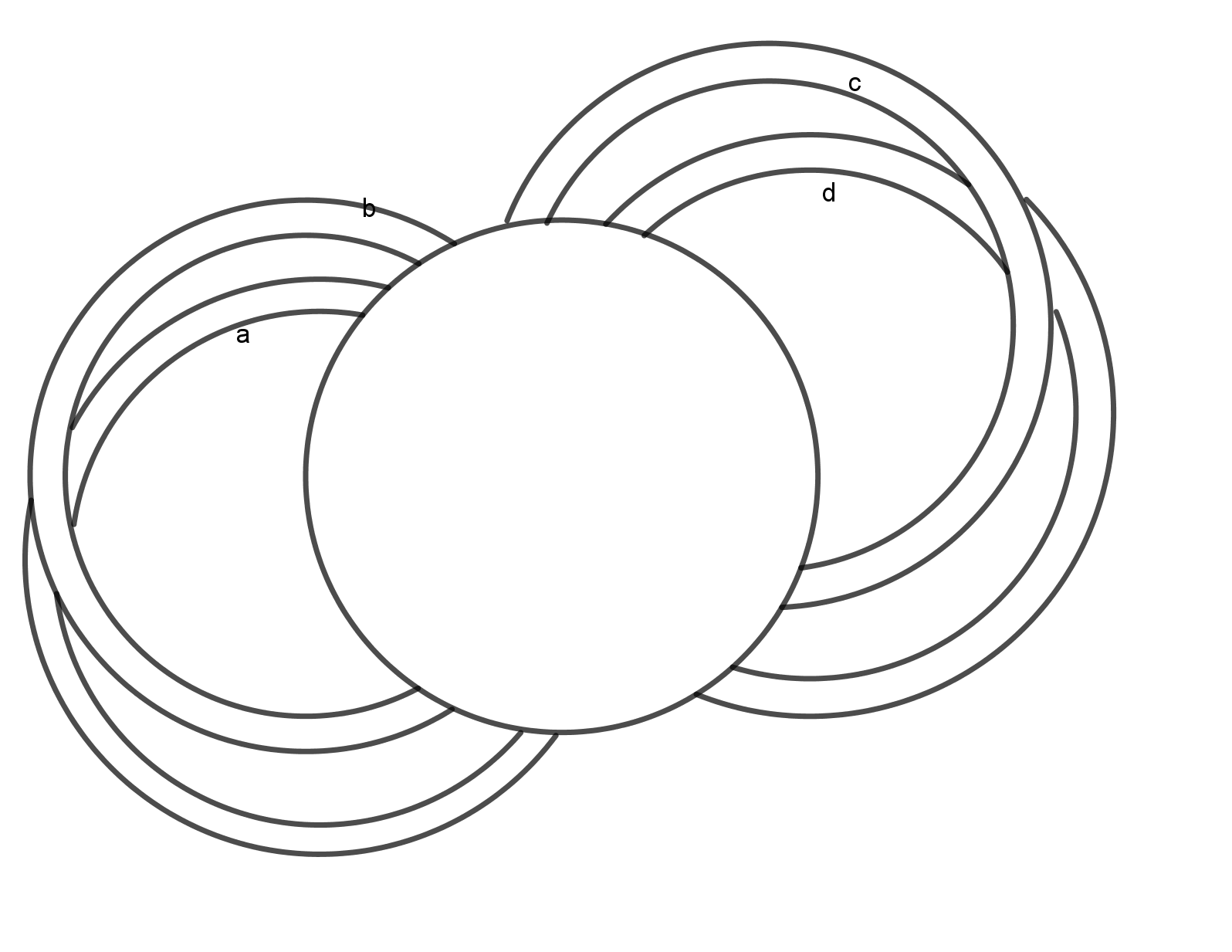
An hieroglyph on n letters is a cyclic sequence of the letters 1,2, . . . , n of length 2n such that each letter appears in the sequence twice.Take an hieroglyph H. Take a convex polygon with 2n sides. Put the letters in the sequence of letters of the hieroglyph on the sides of the convexpolygon in the same order. For each letter i glue the ends of a ribbon to thepair of sides corresponding to the letter i. Call the resulting surface a disk with ribbons corresponding to the hieroglyph H. An hieroglyph H is weakly realizable on the M\"obius strip if some disk with ribbons corresponding to H can be cut out of the M\"obius strip. We give a criterion for weak realizability, which gives a quadratic (in the number of letters) algorithm. Our criterion is based on the Mohar criterion for realizability of a disk with ribbons in the M\"obius strip.