ANOVA for Data in Metric Spaces, with Applications to Spatial Point Patterns

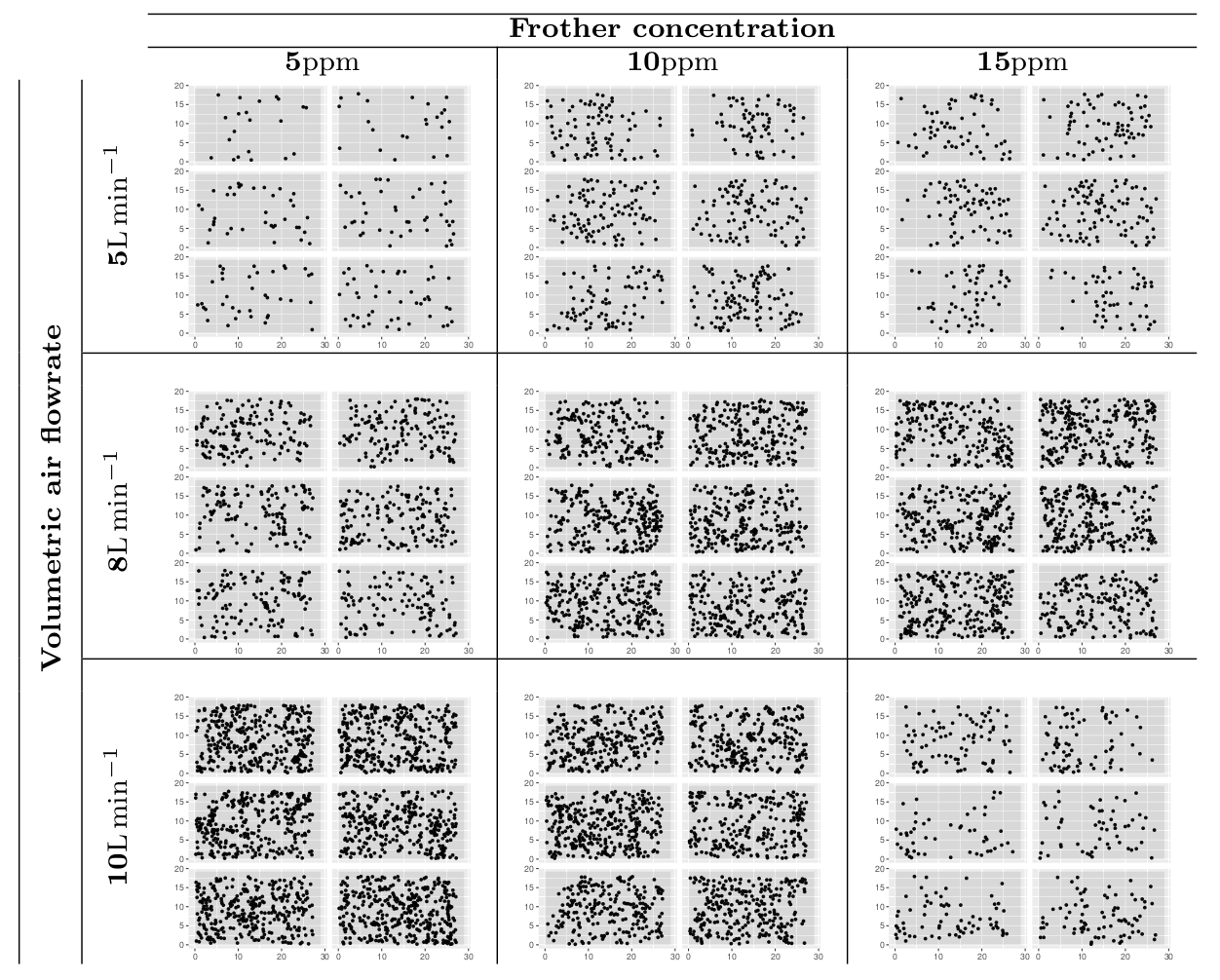
We give a review of recent ANOVA-like procedures for testing group differences based on data in a metric space and present a new such procedure. Our statistic is based on the classic Levene's test for detecting differences in dispersion. It uses only pairwise distances of data points and and can be computed quickly and precisely in situations where the computation of barycenters ("generalized means") in the data space is slow, only by approximation or even infeasible. We show the asymptotic normality of our test statistic and present simulation studies for spatial point pattern data, in which we compare the various procedures in a 1-way ANOVA setting. As an application, we perform a 2-way ANOVA on a data set of bubbles in a mineral flotation process.