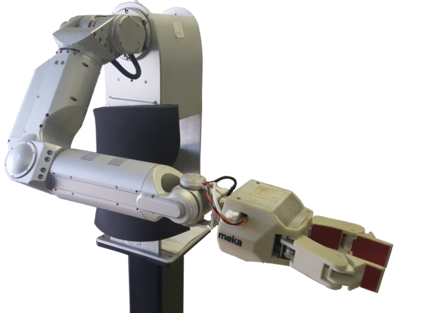
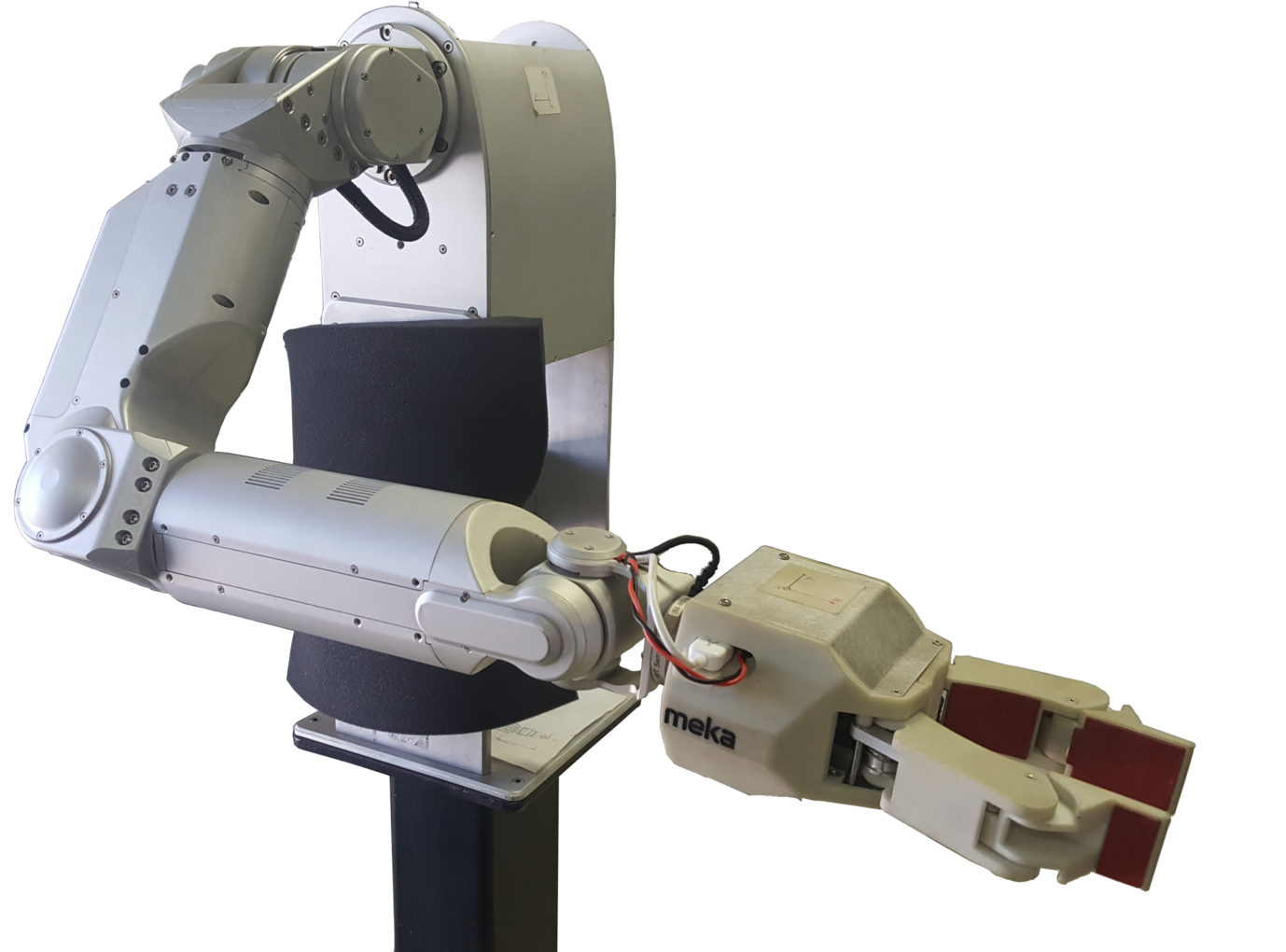
This paper proposes a robust dual-quaternion based H-infinity task-space kinematic controller for robot manipulators. To address the manipulator liability to modeling errors, uncertainties, exogenous disturbances, and their influence upon the kinematics of the end-effector pose, we adapt H-infinity techniques\textemdash suitable only for additive noises\textemdash to unit dual quaternions. The noise to error attenuation within the H-infinity framework has the additional advantage of casting aside requirements concerning noise distributions, which are significantly hard to characterize within the group of rigid-body transformations. Using dual quaternion algebra, we provide a connection between performance effects over the end-effector trajectory and different sources of uncertainties and disturbances while satisfying attenuation requirements with minimum instantaneous control effort. The result is an easy-to-implement closed-form H-infinity control design criterion. The performance of the proposed strategy is evaluated within different realistic simulated scenarios and validated through real experiments.
翻译:本文建议对机器人操纵者采用基于 H- 无限任务- 空间运动控制器。 为解决模拟错误、不确定因素、外生扰动的操纵者责任及其对最终效应构成的动力学的影响,我们调整了H- 无限技术,使之仅适用于添加噪音/ Textemdash, 以配制双四。 H- 无限框架内的降低误差噪音的另一个好处是将噪音分布要求搁置一边,这些要求在硬体变异组中非常难以定性。 使用双重重电代数,我们提供了终端效应和不同不确定性和扰动源的性能效应之间的联系,同时用最低瞬时控制努力满足了减速要求。 结果是易于执行的封闭式H- 无限控制设计标准。 拟议战略的效绩在不同现实的模拟情景中进行评估,并通过实际实验加以验证。