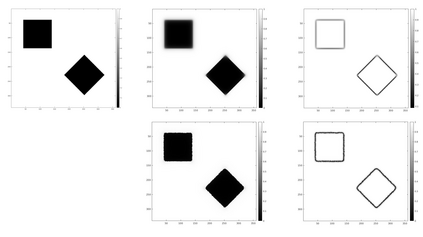
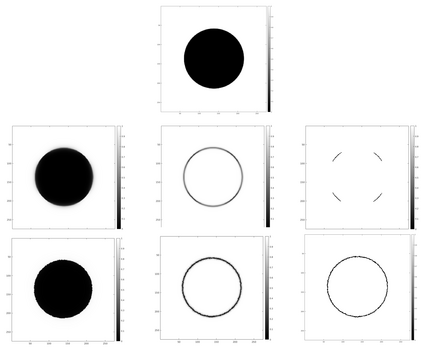
We propose and analyze a finite-difference discretization of the Ambrosio-Tortorelli functional. It is known that if the discretization is made with respect to an underlying periodic lattice of spacing $\delta$, the discretized functionals $\Gamma$-converge to the Mumford-Shah functional only if $\delta\ll\varepsilon$, $\varepsilon$ being the elliptic approximation parameter of the Ambrosio-Tortorelli functional. Discretizing with respect to stationary, ergodic and isotropic random lattices we prove this $\Gamma$-convergence result also for $\delta\sim\varepsilon$, a regime at which the discretization with respect to a periodic lattice converges instead to an anisotropic version of the Mumford-Shah functional.
翻译:我们建议并分析Ambrosio-Tortorelli功能的有限差异分解。 众所周知, 如果对一个基周期间距的间隔值进行分解, 则 Mumford- Shah 的分解功能值为$\delta\ll\ varepsilon, $\ varepsilon$, 是 Ambrosio- Tortorelli 功能的椭圆近似参数。 对固定、 ergodic 和异氧随机拉特克的分解, 我们证明, $\ Gamma$- convergence 结果也是$\delta\ sim\ varepsilon 的, 定期的分解功能值与 Mumford- Shah 功能的亚色版相融合。