$k$-Means Clustering for Persistent Homology

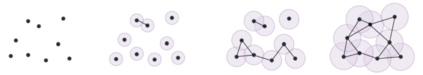
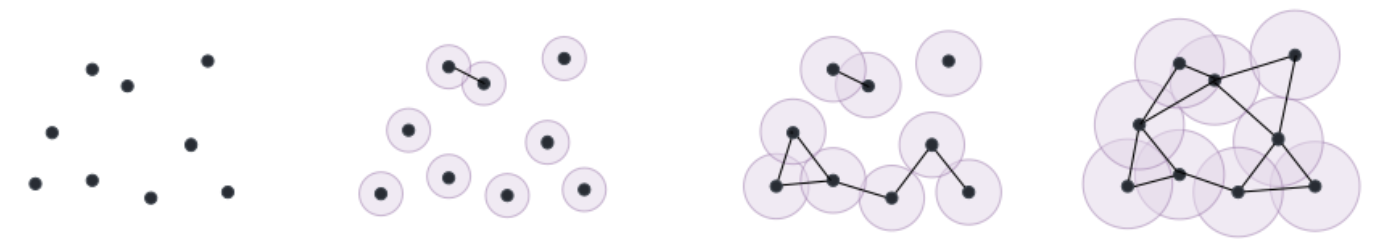
Persistent homology is a fundamental methodology from topological data analysis that summarizes the lifetimes of topological features within a dataset as a persistence diagram; it has recently gained much popularity from its myriad successful applications to many domains. However, a significant challenge to its widespread implementation, especially in statistical methodology and machine learning algorithms, is the format of the persistence diagram as a multiset of half-open intervals. In this paper, we comprehensively study $k$-means clustering where the input is various embeddings of persistence diagrams, as well as persistence diagrams themselves and their generalizations as persistence measures. We show that the clustering performance directly on persistence diagrams and measures far outperform their vectorized representations, despite their more complex representations. Moreover, we prove convergence of the algorithm on persistence diagram space and establish theoretical properties of the solution to the optimization problem in the Karush--Kuhn--Tucker framework.