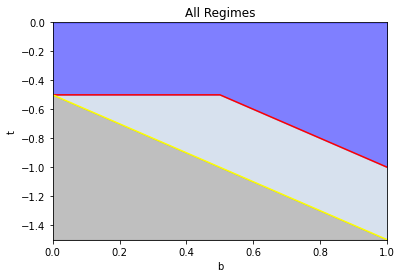
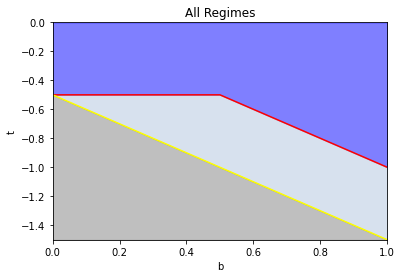
In this paper, we study sparse signal detection problems in Degree Corrected Exponential Random Graph Models (ERGMs). We study the performance of two tests based on the conditionally centered sum of degrees and conditionally centered maximum of degrees, for a wide class of such ERGMs. The performance of these tests match the performance of the corresponding uncentered tests in the $\beta$ model. Focusing on the degree corrected two star ERGM, we show that improved detection is possible at criticality using a test based on (unconditional) sum of degrees. In this setting we provide matching lower bounds in all parameter regimes, which is based on correlations estimates between degrees under the alternative, and of possible independent interest.
翻译:在本文中,我们研究了在度校正指数随机图模型(ERGMs)中微弱的信号检测问题。我们研究了两种测试的性能,其依据是有条件的以度为中心的总和和和有条件的以度为中心的最大度。这些测试的性能与在$\bea$模型中相应的非中心测试的性能相匹配。我们以两颗恒星的校正指数为焦点,通过基于(无条件的)度之和的测试,我们发现在临界度上改进检测是可能的。在此情况下,我们提供所有参数系统中的下限,其依据是替代值各度之间的相关性估计,以及可能的独立利益。